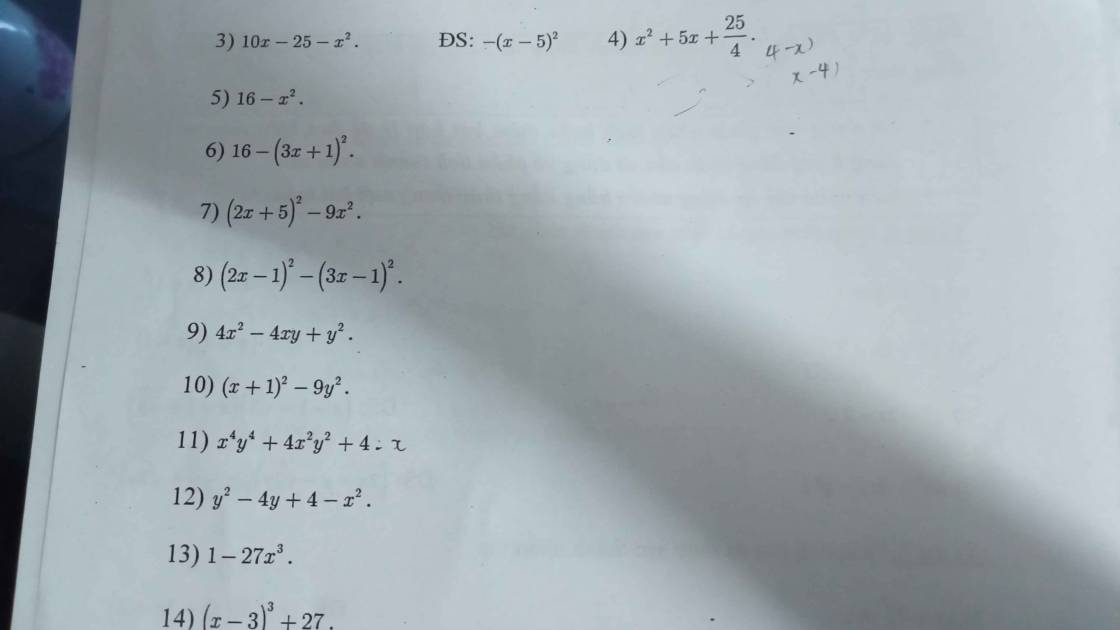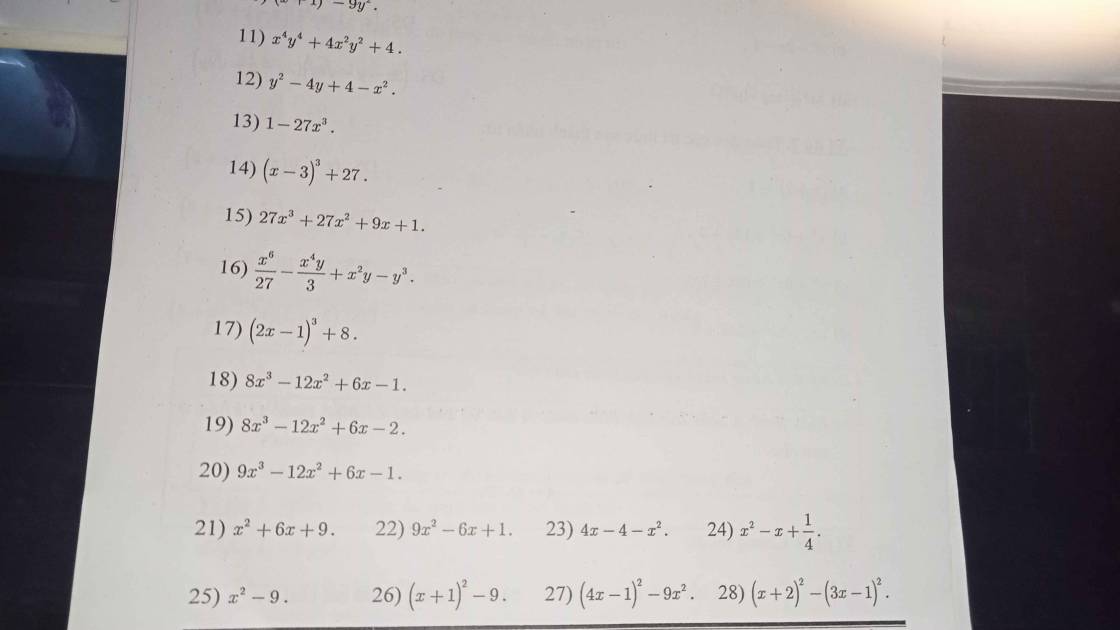Bài mik để dưới phần trả lời ạ
Bài 11: Chia đa thức cho đơn thức
a) (x + 2)³ + 1
= (x + 2)³ + 1³
= [(x + 2) + 1][(x + 2)² - (x + 2).1 + 1²]
= (x + 3)(x² + 4x + 4 - x - 2 + 1)
= (x + 3)(x² + 3x + 3)
b) x³ + 6x² + 12x + 9
= x³ + 3x² + 3x² + 9x + 3x + 9
= (x³ + 3x²) + (3x² + 9x) + (3x + 9)
= x²(x + 3) + 3x(x + 3) + 3(x + 3)
= (x + 3)(x² + 3x + 3)
c) x³ + 6x² + 12x + 7
= x³ + x² + 5x² + 5x + 7x + 7
= (x³ + x²) + (5x² + 5x) + (7x + 7)
= x²(x + 1) + 5x(x + 1) + 7(x + 1)
= (x + 1)(x² + 5x + 7)
d) 2x³ + 6x² + 12x + 8
= 2(x³ + 3x² + 6x + 4)
= 2(x³ + x² + 2x² + 2x + 4x + 4)
= 2[(x³ + x²) + (2x² + 2x) + (4x + 4)]
= 2[x²(x + 1) + 2x(x + 1) + 4(x + 1)]
= 2(x + 1)(x² + 2x + 4)
Đúng 2
Bình luận (0)
a: =(x+2+1)(x^2+4x+4-x-2+1)
=(x+3)(x^2+3x+3)
b: =x^3+6x^2+12x+8+1
=(x+2)^3+1
=(x+3)(x^2+3x+3)
c: =x^3+x^2+5x^2+5x+7x+7
=(x+1)(x^2+5x+7)
Đúng 1
Bình luận (0)
Bài mik để dưới phần trả lời ạ
1: =x^2-2*x*2y+4y^2
=(x-2y)^2
2: 9x^2-y^2=(3x-y)(3x+y)
5: x^3-8=(x-2)(x^2+2x+4)
4: =x^2-(y-2)^2
=(x-y+2)(x+y-2)
7: 8x^3+12x^2+6x+1=(2x+1)^3
14: =x^3-3x^2+3x-1-1
=(x-1)^3-1
=(x-1-1)(x^2-2x+1+x-1+1)
=(x-2)(x^2-x+1)
15: =x^3+x^2-4x^2-4x+7x+7
=(x+1)(x^2-4x+7)
16: =2x^3-x^2-2x^2+x+2x-1
=(2x-1)(x^2-x+1)
Đúng 1
Bình luận (0)
Bài mik để dưới phần trả lời ạ
14
\(=\left(x-3\right)^3+3^3=\left(x-3+3\right)\left[\left(x-3\right)^2-\left(x-3\right).3+3^2\right]\\ =x\left(x^2-6x+9-3x+9+9\right)=x\left(x^2-9x+27\right)\)
Đúng 1
Bình luận (0)
3: =-(x^2-10x+25)
=-(x-5)^2
5: 16-x^2=(4-x)(4+x)
6: 16-(3x+1)^2
=(4-3x-1)(4+3x+1)
=(3x+5)*3(1-x)
7: =(2x+5+3x)(2x+5-3x)
=(5-x)*5*(x+10
8: =(2x-1-3x+1)(2x-1+3x-1)
=-x(5x-2)
9: =(2x-y)^2
10: =(x+1+3y)(x+1-3y)
12: =(y-2)^2-x^2
=(y-2-x)(y-2+x)
Đúng 0
Bình luận (0)
Bài mik để dưới phần trả lời ạ
11: =(x^2y^2)^2+2*x^2y^2*2+2
=(x^2y^2+2)^2
12: =(y-2)^2-x^2
=(y-2-x)(y-2+x)
13: =(1-3x)(1+3x+9x^2)
14: =(x-3+3)(x^2-6x+9-3x+9+9)
=x(x^2-3x+9)
15: =(3x+1)^3
16: =(1/3x^2-y)^3
17: =(2x-1+2)(4x^2-4x+1-4x+2+4)
=(2x+1)(4x^2-8x+7)
18: =(2x-1)^3
19: =(2x-1)^3-1
=(2x-1-1)(4x^2-4x+1+2x-1+1)
=(2x-2)(4x^2-2x+1)
=2(x-1)(4x^2-2x+1)
Đúng 1
Bình luận (0)
a)[2(x-y)3-7(y-x)2-(y-x)]:(x-y)
b)[3(x-y)5-2(x-y)4+3(x-y)2]:[5(x-y)2 ]
a: =2(x-y)^3/(x-y)-7(x-y)^2/(x-y)+(x-y)/(x-y)
=2(x-y)^2-7(x-y)+1
b: =3(x-y)^5/5(x-y)^2-2(x-y)^4/5(x-y)^2+3(x-y)^2/5(x-y)^2
=3/5(x-y)^3-2/5(x-y)^2+3/5
Đúng 1
Bình luận (0)
\(a,\)
\(\left[2\left(x-y\right)^3-7\left(y-x\right)^2-\left(y-x\right)\right]:\left(x-y\right)\)
\(=\left[2\left(x-y\right)^3-7\left(x-y\right)^2+\left(x-y\right)\right]:\left(x-y\right)\)
\(=\left\{\left(x-y\right)\left[2\left(x-y\right)^2-7\left(x-y\right)+1\right]\right\}:\left(x-y\right)\)
\(=2\left(x-y\right)^2-7\left(x-y\right)+1\)
\(b,\)
\(\left[3\left(x-y\right)^5-2\left(x-y\right)^4+3\left(x-y\right)^2\right]:\left[5\left(x-y\right)^2\right]\)
\(=\dfrac{3}{5}\left(x-y\right)^3-\dfrac{2}{5}\left(x-y\right)^2+\dfrac{3}{5}\)
Đúng 1
Bình luận (0)
biết rằng đa thức f(x) khi chia cho x-2 có số dư 6067, khi chia cho x+3 có số dư -4043. Tìm đa thức dư khi f(x) chia cho x2+x-6
Lời giải:
Gọi đa thức dư khi lấy $f(x)$ chia cho $x^2+x-6$ là $ax+b$ với $a,b\in\mathbb{R}$, $Q(x)$ là đa thức thương.
Theo bài ra ta có:
$f(2)=6067$
$f(-3)=-4043$
$f(x)=(x^2+x-6)Q(x)+ax+b=(x-2)(x+3)Q(x)+ax+b$
Cho $x=2$ thì:
$f(2)=0.Q(2)+2a+b=2a+b$
$\Leftrightarrow 6067=2a+b(1)$
Cho $x=-3$ thì:
$f(-3)=0.Q(-3)-3a+b=-3a+b$
$\Leftrightarrow -4043=-3a+b(2)$
Từ $(1); (2)\Rightarrow a=2022; b=2023$
Vậy đa thức dư là $2022x+2023$
Đúng 3
Bình luận (0)
(3x^2-6x):(2-x)
\(\left(3x^2-6x\right):\left(2-x\right)\\ =3x\left(x-2\right):\left(2-x\right)\\ =-3x\left(2-x\right):\left(2-x\right)\\ =-3x\)
Đúng 3
Bình luận (0)
\(\left(3x^2-6x\right):\left(2-x\right)=-3x\left(2-x\right):\left(2-x\right)=-3x\)
Đúng 3
Bình luận (0)
bài 1 :thực hiện phép tính:
1) ( \(10x^2y-8xy^3\))\(:2xy\)
bạn nào trả lời đầu tiên mik sẽ vote sao nhe
\(\left(10x^2y-8xy^3\right):2xy=5x-4y^2\)
Đúng 4
Bình luận (0)
(10x²y - 8xy³) : 2xy
= (10x²y : 2xy) - (8xy³ : 2xy)
= 5x - 4y²
Đúng 2
Bình luận (0)
\(1,\left(10x^2y-8xy^3\right):2xy=5x-4y^2\)
Đúng 1
Bình luận (0)
(x^2 -2x -y^2 + 1): ( x-y-1) giúp mình với các bạn ơi
\(\left(x^2-2x-y^2+1\right)=\left(x^2-2x+1\right)-y^2=\left(x-1\right)^2-y^2=\left(x-1-y\right).\left(x-1+y\right)=\left(x-y-1\right).\left(x+y-1\right)\)
\(\left(x-y-1\right).\left(x-1+y\right):\left(x-y-1\right)=x-1+y\)
\(\Rightarrow\left(x^2-2x-y^2+1\right):\left(x-y-1\right)=x-1+y\)
Đúng 2
Bình luận (0)