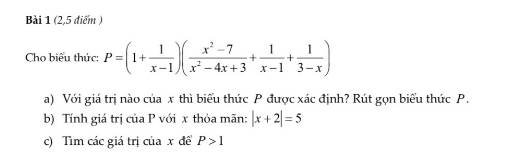
Bài 1: Nhân đơn thức với đa thức
a: ĐKXĐ: x<>1; x<>3; x<>-3
\(P=\dfrac{x-1+1}{x-1}:\dfrac{x^2-7+x-3-x+1}{\left(x-3\right)\left(x-1\right)}\)
\(=\dfrac{x}{x-1}\cdot\dfrac{\left(x-1\right)\left(x-3\right)}{x^2-9}=\dfrac{x}{x+3}\)
b: |x+2|=5
=>x+2=5 hoặc x+2=-5
=>x=-7(nhận) hoặc x=3(loại)
Khi x=-7 thì \(P=\dfrac{-7}{-7+3}=\dfrac{-7}{-4}=\dfrac{7}{4}\)
c: Để P>1 thì P-1>0
=>\(\dfrac{x-x-3}{x+3}>0\)
=>x+3<0
=>x<-3
Đúng 0
Bình luận (0)
a. \(x\ne1,x\ne3\)
\(P=\left(1+\dfrac{1}{x-1}\right)\left(\dfrac{x^2-7}{x^2-4x+3}+\dfrac{1}{x-1}+\dfrac{1}{3-x}\right)\)
\(P=\left(\dfrac{x}{x-1}\right)\left(\dfrac{x^2-7}{\left(x-1\right)\left(x-3\right)}+\dfrac{1}{x-1}-\dfrac{1}{x-3}\right)\)
\(P=\dfrac{x}{x-1}\left(\dfrac{x^2-7+x-3-x+1}{\left(x-1\right)\left(x-3\right)}\right)=\dfrac{x}{x-1}.\dfrac{x^2-9}{\left(x-1\right)\left(x-3\right)}\)
\(P=\dfrac{x}{x-1}.\dfrac{\left(x-3\right)\left(x+3\right)}{\left(x-1\right)\left(x-3\right)}=\dfrac{x^2+3x}{\left(x-1\right)^2}\)
b. \(\left|x+2\right|=5\Leftrightarrow\left[{}\begin{matrix}x=3\left(loại\right)\\x=-7\end{matrix}\right.\)
\(P=\dfrac{49-21}{64}=\dfrac{7}{16}\)
c. \(P>1\Leftrightarrow\dfrac{x^2+3x}{\left(x-1\right)^2}>1\left(x\ne1\right)\)
\(\Leftrightarrow\dfrac{x^2+3x-x^2+2x-1}{\left(x-1\right)^2}>0\Leftrightarrow\dfrac{5x-1}{\left(x-1\right)^2}>0\)
\(\Leftrightarrow5x-1>0\Leftrightarrow x>\dfrac{1}{5}\)
Đúng 0
Bình luận (0)
x2*(5x3-x-1/2)
\(x^2.\left(5x^3-x-\dfrac{1}{2}\right)\)
= \(5x^5-x^3-\dfrac{1}{2}x^2\)
Đúng 0
Bình luận (0)
7x(x-4)-(7x+3)(2X^2+4)
=7x^2-28x-14x^3-28x-6x^2-12
=-14x^3+x^2-56x-12
Đúng 0
Bình luận (0)
`7x . (x-4) - ( 7x + 3 ) . ( 2x^2 + 4 )`
`= 7x^2 - 28x - ( 14x^3 + 28x + 6x^2 + 12 )`
`= 7x^2 - 28x - 14x^3 - 28x - 6x^2 - 12`
`= -14x^3 + x^2 - 56x - 12`
Đúng 0
Bình luận (0)
(2^2-1313xy+y^2). (-3x^3)
\((2^2-1313xy+y^2). (-3x^3)\)
\(=2^2.\left(-3x^3\right)-1313xy.\left(-3x^3\right)+y^2\left(-3x^3\right)\\ =-12x^3+3939x^4y-3x^3y^2\)
Mà này, đề đúng chưa đấy? Nghi quá :v
Đúng 2
Bình luận (1)
a) 2x(3x2 - 5x +3 ) = 6x3 - 10x2 + 6x
b) -2x2 ( x2 + 5x - 3 ) = -2x4 - 10x3 + 6x2
c) - \(\dfrac{1}{2}\)x2 ( 2x3 - 4x +3 ) = -x5 + \(\dfrac{1}{2}\)x3 - \(\dfrac{3}{2}\)x2
d) ( 2x - 1 ) ( x2 + 5 - 4 ) = 2x3 + 10x - 8x - x2 - 5 + 4
= 2x3 - x2 + 2x -1
e) 7x (x -4 ) - ( 7x + 3 ) ( 2x2 - x + 4 )
= 7x2 - 28x - ( 14x3 - 7x2 + 28x + 6x2- 3x + 12 )
=7x2 - 28x - ( 14x3 - x2 + 25x + 12 )
= 7x2 - 28x - 14x3 + x2 - 25x - 12
=8x2 - 53x - 14x3 -12
Đúng 0
Bình luận (0)
a) 2x(3x2 - 5x +3 ) = 6x3 - 10x2 + 6x
b) -2x2 ( x2 + 5x - 3 ) = -2x4 - 10x3 + 6x2
c) - 1212x3 -
Đúng 0
Bình luận (0)
Bài 1:
a.
$2x(3x^2-5x+3)=2x.3x^2-2x.5x+2x.3=6x^3-10x^2+6x$
b.
$-2x^2(x^2+5x-3)=-2x^2.x^2+(-2x^2).5x+(-2x^2)(-3)$
$=-2x^4-10x^3+6x^2$
c.
$\frac{-1}{2}x^2(2x^3-4x+3)=\frac{-1}{2}x^2.2x^3+(\frac{-1}{2}x^2).(-4x)+(\frac{-1}{2}x^2).3$
$=-x^5+2x^3-\frac{3}{2}x^2$
d.
$(2x-1)(x^2+5-4)=(2x-1)(x^2+1)=2x(x^2+1)-(x^2+1)=2x^3+2x-x^2-1$
e.
$7x(x-4)-(7x+3)(2x^2-x+4)$
$=7x^2-28x-(7x.2x^2-7x.x+7x.4+3.2x^2-3x+12)$
$=7x^2-28x-(14x^3-7x^2+28x+6x^2-3x+12)$
$=7x^2-28x-(14x^3-x^2+25x+12)$
$=7x^2-28x-14x^3+x^2-25x-12=-14x^3+8x^2-53x-12$
Đúng 0
Bình luận (0)
Bài 2:
a.
$3x(x+1)-2x(x+2)=-1-x$
$3x^2+3x-2x^2-4x=-1-x$
$x^2-x=-1-x$
$x^2-x+x+1=0$
$x^2+1=0$
$x^2=-1<0$ (vô lý)
Do đó pt vô nghiệm.
b.
$4x(x-2019)-x+2019=0$
$4x(x-2019)-(x-2019)=0$
$(x-2019)(4x-1)=0$
$\Rightarrow x-2019=0$ hoặc $4x-1=0$
$\Rightarrow x=2019$ hoặc $x=\frac{1}{4}$
c.
$(x-4)^2-36=0$
$(x-4)^2-6^2=0$
$(x-4-6)(x-4+6)=0$
$(x-10)(x+2)=0$
$\Rightarrow x-10=0$ hoặc $x+2=0$
$\Rightarrow x=10$ hoặc $x=-2$
Đúng 0
Bình luận (0)
Bài 2:
d.
$x^2+8x+16=0$
$x^2+2.x.4+4^2=0$
$(x+4)^2=0$
$\Rightarrow x+4=0$
$\Rightarrow x=-4$
e.
$x(x+6)-7x-42=0$
$x(x+6)-7(x+6)=0$
$(x+6)(x-7)=0$
$\Rightarrow x+6=0$ hoặc $x-7=0$
$\Rightarrow x=-6$ hoặc $x=7$
f.
$25x^2-9=0$
$(5x)^2-3^2=0$
$(5x-3)(5x+3)=0$
$\Rightarrow 5x-3=0$ hoặc $5x+3=0$
$\Rightarrow x=\frac{3}{5}$ hoặc $x=\frac{-3}{5}$
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Rút gọn (-3x+2)(5x^2-1/3x+4)
\(=-15x^3+x^2-12x+10x^2-\dfrac{2}{3}x+8\)
=-15x^3+11x^2-38/3x+8
Đúng 0
Bình luận (0)
3*(x+2)-2 bằng10
3*(x + 2 ) - 2 = 10
3*(x+2) = 10 + 2
3*(x+2) = 12
x + 2 = 12 : 3
x + 2 = 4
x = 4 - 2
x = 2
vậy x = 2
Đúng 1
Bình luận (0)
1 con vịt và 1 con gà nặng 5kg,con gà đó và con ngỗng nặng 6kg, con ngỗng đó và con vịt nặng 7kg.Hỏi trung bình mỗi con nặng bao nhiêu ? Vì sao
Tổng cân nặng của ba con là;
(5+6+7)/2=9(kg)
Trung bình 1 con nặng;
9/3=3(kg)
Đúng 0
Bình luận (0)






