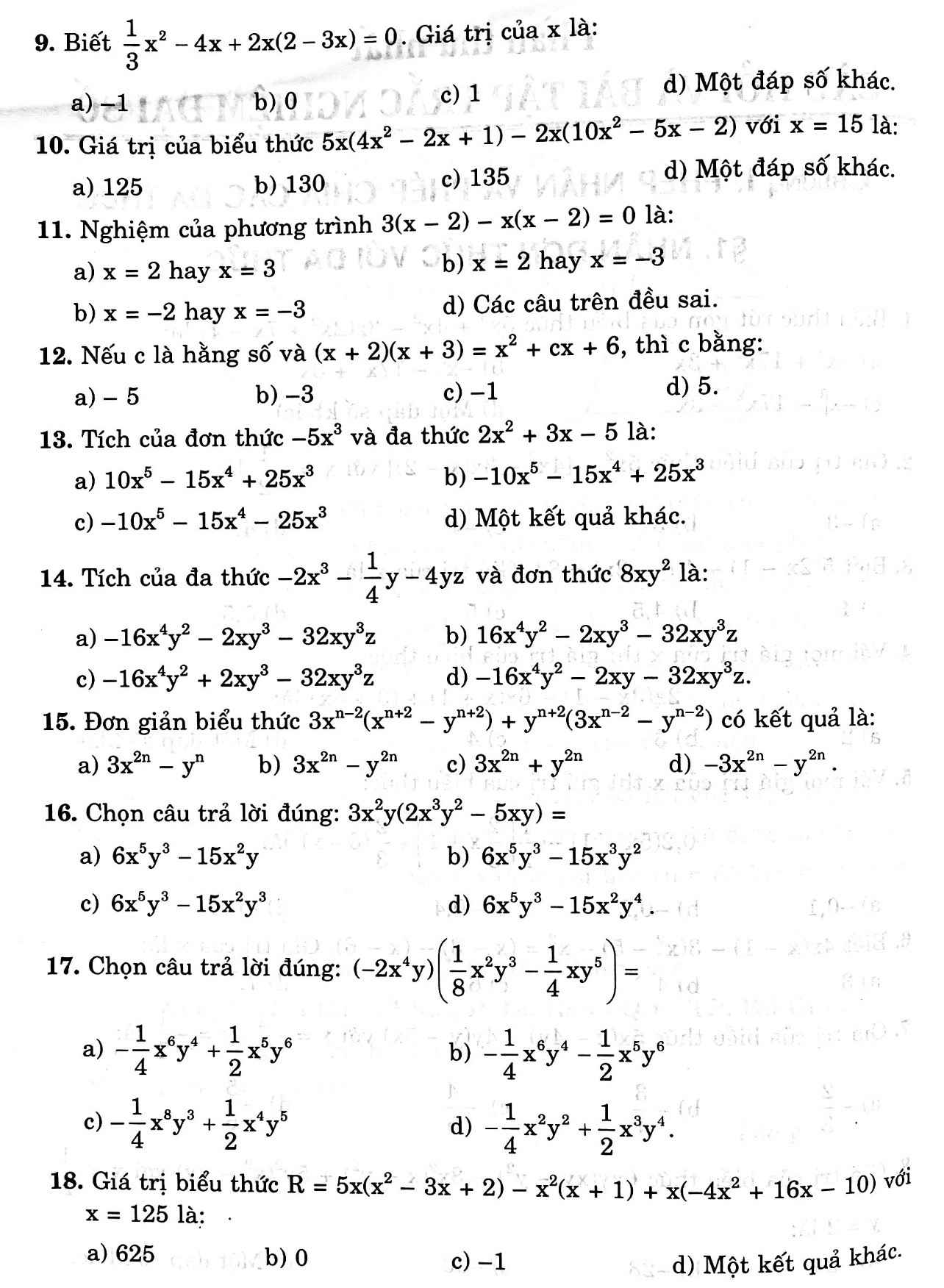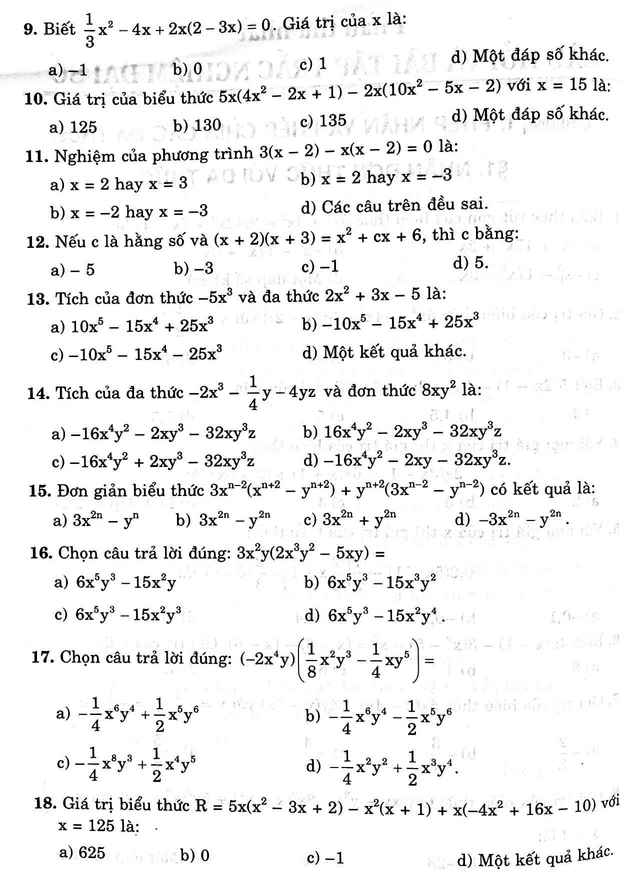Bài 1:
a.
$2x(3x^2-5x+3)=2x.3x^2-2x.5x+2x.3=6x^3-10x^2+6x$
b.
$-2x^2(x^2+5x-3)=-2x^2.x^2+(-2x^2).5x+(-2x^2)(-3)$
$=-2x^4-10x^3+6x^2$
c.
$\frac{-1}{2}x^2(2x^3-4x+3)=\frac{-1}{2}x^2.2x^3+(\frac{-1}{2}x^2).(-4x)+(\frac{-1}{2}x^2).3$
$=-x^5+2x^3-\frac{3}{2}x^2$
d.
$(2x-1)(x^2+5-4)=(2x-1)(x^2+1)=2x(x^2+1)-(x^2+1)=2x^3+2x-x^2-1$
e.
$7x(x-4)-(7x+3)(2x^2-x+4)$
$=7x^2-28x-(7x.2x^2-7x.x+7x.4+3.2x^2-3x+12)$
$=7x^2-28x-(14x^3-7x^2+28x+6x^2-3x+12)$
$=7x^2-28x-(14x^3-x^2+25x+12)$
$=7x^2-28x-14x^3+x^2-25x-12=-14x^3+8x^2-53x-12$
Bài 2:
a.
$3x(x+1)-2x(x+2)=-1-x$
$3x^2+3x-2x^2-4x=-1-x$
$x^2-x=-1-x$
$x^2-x+x+1=0$
$x^2+1=0$
$x^2=-1<0$ (vô lý)
Do đó pt vô nghiệm.
b.
$4x(x-2019)-x+2019=0$
$4x(x-2019)-(x-2019)=0$
$(x-2019)(4x-1)=0$
$\Rightarrow x-2019=0$ hoặc $4x-1=0$
$\Rightarrow x=2019$ hoặc $x=\frac{1}{4}$
c.
$(x-4)^2-36=0$
$(x-4)^2-6^2=0$
$(x-4-6)(x-4+6)=0$
$(x-10)(x+2)=0$
$\Rightarrow x-10=0$ hoặc $x+2=0$
$\Rightarrow x=10$ hoặc $x=-2$
Bài 2:
d.
$x^2+8x+16=0$
$x^2+2.x.4+4^2=0$
$(x+4)^2=0$
$\Rightarrow x+4=0$
$\Rightarrow x=-4$
e.
$x(x+6)-7x-42=0$
$x(x+6)-7(x+6)=0$
$(x+6)(x-7)=0$
$\Rightarrow x+6=0$ hoặc $x-7=0$
$\Rightarrow x=-6$ hoặc $x=7$
f.
$25x^2-9=0$
$(5x)^2-3^2=0$
$(5x-3)(5x+3)=0$
$\Rightarrow 5x-3=0$ hoặc $5x+3=0$
$\Rightarrow x=\frac{3}{5}$ hoặc $x=\frac{-3}{5}$