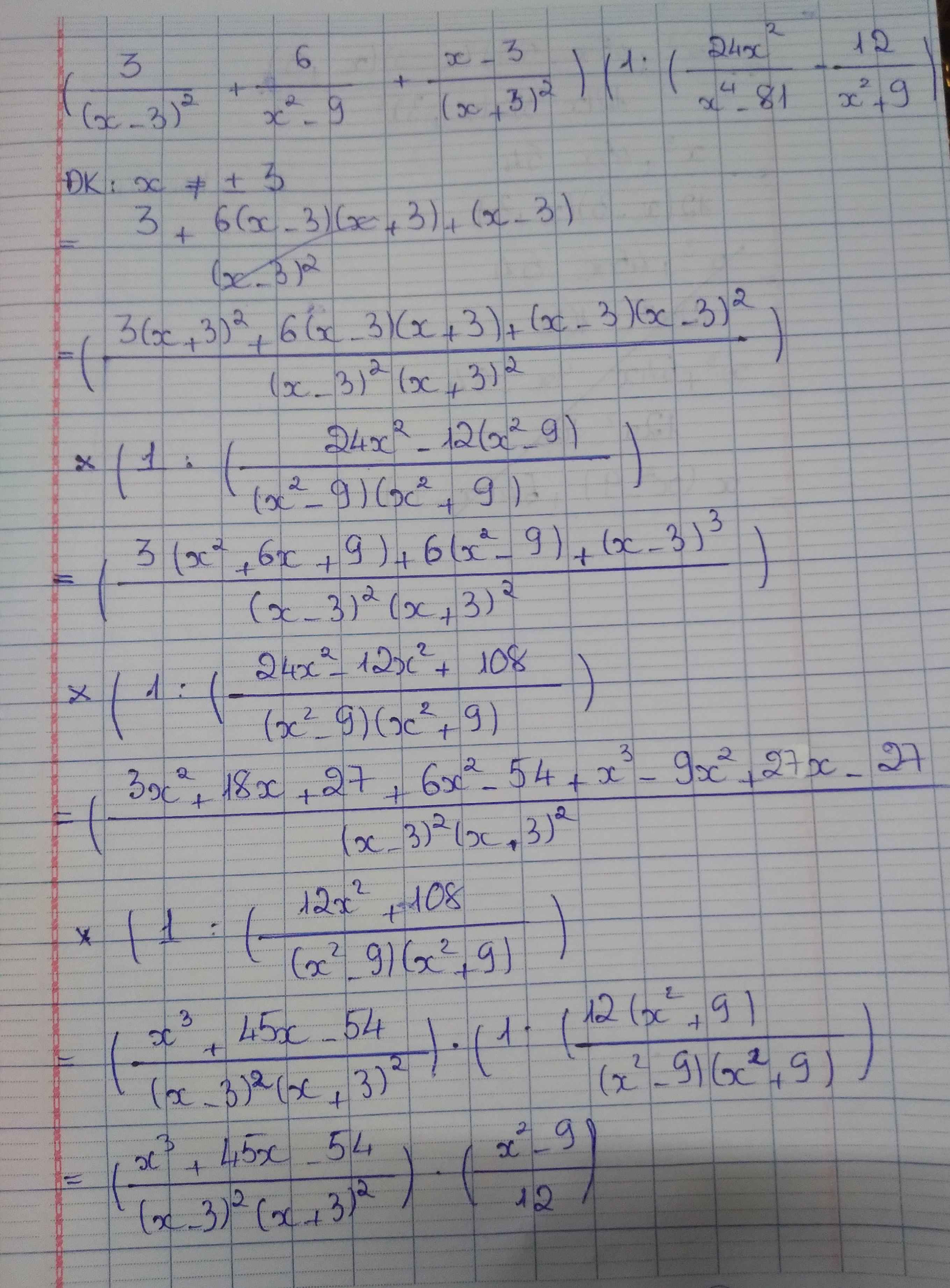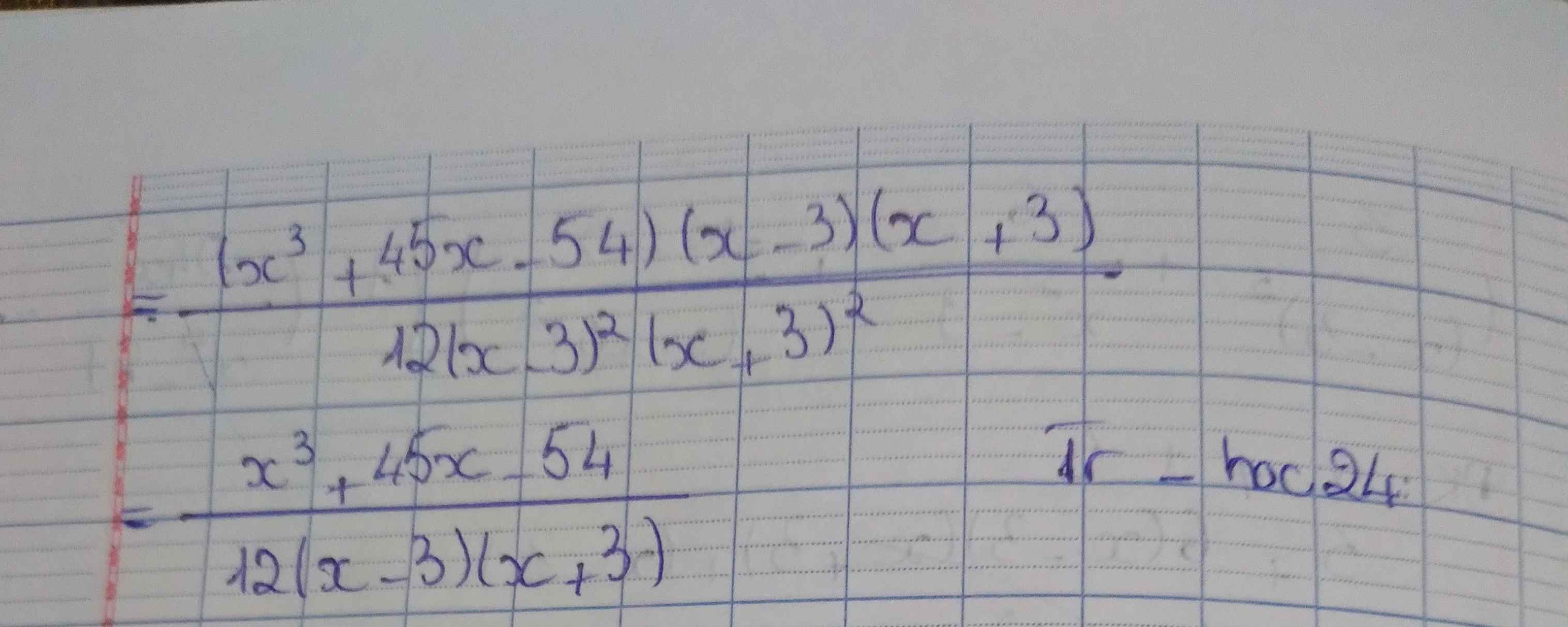\(\dfrac{x+2}{x-3}\)+\(\dfrac{x-2}{x+3}\)=\(\dfrac{2\left(x^2+6\right)}{x^2-9}\)
H24
Những câu hỏi liên quan
a)\(\dfrac{2}{x+2}-\dfrac{1}{x+3}+\dfrac{2x+5}{\left(x+2\right)\left(x+3\right)}\)
b)\(\dfrac{2}{x+1}-\dfrac{1}{x+5}+\dfrac{2x+6}{\left(x+5\right)\left(x+1\right)}\)
c)\(\dfrac{-6}{x^2-9}-\dfrac{1}{x+3}+\dfrac{3}{x-3}\)
d)\(\dfrac{x}{x-2}-\dfrac{x}{x+2}+\dfrac{8}{x^2-4}\)
Rút gọn:
\(A=\left[\dfrac{x+3}{\left(x-3\right)^2}+\dfrac{6}{x^2-9}-\dfrac{x-3}{\left(x+3\right)^2}\right]\left[1:\left(\dfrac{24x^2}{x^4-81}-\dfrac{12}{x^2+9}\right)\right]\)
\(B=\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right):\left[\left(x-2\right)+\dfrac{10-x^2}{x+2}\right]\)
a) \(A=\left[\dfrac{x+3}{\left(x-3\right)^2}+\dfrac{6}{x^2-9}-\dfrac{x-3}{\left(x+3\right)^2}\right]\left[1:\left(\dfrac{24x^2}{x^4-81}-\dfrac{12}{x^2+9}\right)\right]\)
\(\left(ĐKXĐ:x\ne\pm3\right)\)
\(=\dfrac{\left(x+3\right)^3+6\left(x-3\right)\left(x+3\right)-\left(x-3\right)^3}{\left(x-3\right)^2\left(x+3\right)^2}\cdot\left[1:\dfrac{24x^2-12\left(x^2-9\right)}{\left(x^2-9\right)\left(x^2+9\right)}\right]\)
\(=\dfrac{x^3+9x^2+27x+27+6x^2-54-x^3+9x^2-27x+27}{\left(x-3\right)^2\left(x+3\right)^2}\cdot\dfrac{\left(x^2-9\right)\left(x^2+9\right)}{24x^2-12x^2+108}\)
\(=\dfrac{24x^2\left(x^2+9\right)\left(x-3\right)\left(x+3\right)}{12\left(x^2+9\right)\left(x-3\right)^2\left(x+3\right)^2}\)
\(=\dfrac{2x^2}{x^2-9}\)
b) \(B=\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right):\left[\left(x-2\right)+\dfrac{10-x^2}{x+2}\right]\)
\(=\left(\dfrac{x}{x^2-4}-\dfrac{2}{x-2}+\dfrac{1}{x+2}\right):\left(\dfrac{x-2}{1}+\dfrac{10-x^2}{x+2}\right)\)
\(=\dfrac{x-2\left(x+2\right)+x-2}{\left(x-2\right)\left(x+2\right)}:\dfrac{\left(x-2\right)\left(x+2\right)+10-x^2}{x+2}\)
\(=\dfrac{x-2x-4+x-2}{x^2-4}\cdot\dfrac{x+2}{x^2-4+10-x^2}\)
\(=\dfrac{-6\left(x+2\right)}{6\left(x+2\right)\left(x-2\right)}\)
\(=\dfrac{-1}{x-2}\)
Đúng 0
Bình luận (0)
phần b điều kiện xác định là \(x\ne\pm2\) nhé
Đúng 0
Bình luận (0)
Tính tổng sau:
a, \(\dfrac{-1}{x^2-x}+\dfrac{-1}{x^2-3x+2}+\dfrac{-1}{x^2-5x+6}+\dfrac{-1}{x^2-7x+12}+\dfrac{-1}{x^2-9x+20}+\dfrac{1}{x-5}\)
b, \(\dfrac{3}{x\left(x+3\right)}+\dfrac{3}{\left(x+3\right)\left(x+6\right)}+\dfrac{3}{\left(x+6\right)\left(x+9\right)}+\dfrac{1}{x+9}\)
a: \(=-\dfrac{1}{x\left(x-1\right)}+\dfrac{-1}{\left(x-1\right)\left(x-2\right)}+\dfrac{-1}{\left(x-2\right)\left(x-3\right)}+...+-\dfrac{1}{\left(x-4\right)\left(x-5\right)}+\dfrac{1}{x-5}\)
\(=\dfrac{1}{x}-\dfrac{1}{x-1}+\dfrac{1}{x-1}-\dfrac{1}{x-2}+\dfrac{1}{x-2}-\dfrac{1}{x-3}+...+\dfrac{1}{x-4}-\dfrac{1}{x-5}+\dfrac{1}{x-5}\)
=1/x
b: \(=\dfrac{1}{x}-\dfrac{1}{x+3}+\dfrac{1}{x+3}-\dfrac{1}{x+6}+\dfrac{1}{x+6}-\dfrac{1}{x+9}+\dfrac{1}{x+9}\)
=1/x
Đúng 0
Bình luận (0)
Tìm x a,3-xx+1,8b,2x-57x+35c,2left(x+10right)3left(x-6right)d,8left(x-dfrac{3}{8}right)+16left(dfrac{1}{6}+xright)+xe,dfrac{2}{9}-3xdfrac{4}{3}-xg,dfrac{1}{2}x+dfrac{5}{6}dfrac{3}{4}x-dfrac{1}{2}h,x-4dfrac{5}{6}left(6-dfrac{6}{5}xright)k,7x^2-116x^2-2m,5left(x+3.2^3right)10^2n,dfrac{4}{9}-(dfrac{1}{6^2})dfrac{2}{3}left(x-dfrac{2}{3}right)^2+dfrac{5}{12}
Đọc tiếp
Tìm x
\(a,3-x=x+1,8\)
\(b,2x-5=7x+35\)
\(c,2\left(x+10\right)=3\left(x-6\right)\)
\(d,8\left(x-\dfrac{3}{8}\right)+1=6\left(\dfrac{1}{6}+x\right)+x\)
\(e,\dfrac{2}{9}-3x=\dfrac{4}{3}-x\)
\(g,\dfrac{1}{2}x+\dfrac{5}{6}=\dfrac{3}{4}x-\dfrac{1}{2}\)
\(h,x-4=\dfrac{5}{6}\left(6-\dfrac{6}{5}x\right)\)
\(k,7x^2-11=6x^2-2\)
\(m,5\left(x+3.2^3\right)=10^2\)
\(n,\dfrac{4}{9}-(\dfrac{1}{6^2})=\dfrac{2}{3}\left(x-\dfrac{2}{3}\right)^2+\dfrac{5}{12}\)
\(a,3-x=x+1,8\)
\(\Rightarrow-x-x=1,8-3\)
\(\Rightarrow-2x=-1,2\)
\(\Rightarrow x=0,6\)
\(b,2x-5=7x+35\)
\(\Rightarrow2x-7x=35+5\)
\(\Rightarrow-5x=40\)
\(\Rightarrow x=-8\)
\(c,2\left(x+10\right)=3\left(x-6\right)\)
\(\Rightarrow2x+20=3x-18\)
\(\Rightarrow2x-3x=-18-20\)
\(\Rightarrow-x=-38\)
\(\Rightarrow x=38\)
\(d,8\left(x-\dfrac{3}{8}\right)+1=6\left(\dfrac{1}{6}+x\right)+x\)
\(\Rightarrow8x-3+1=1+6x+x\)
\(\Rightarrow8x-3=7x\)
\(\Rightarrow8x-7x=3\)
\(\Rightarrow x=3\)
\(e,\dfrac{2}{9}-3x=\dfrac{4}{3}-x\)
\(\Rightarrow-3x+x=\dfrac{4}{3}-\dfrac{2}{9}\)
\(\Rightarrow-2x=\dfrac{10}{9}\)
\(\Rightarrow x=-\dfrac{5}{9}\)
Đúng 4
Bình luận (0)
\(g,\dfrac{1}{2}x+\dfrac{5}{6}=\dfrac{3}{4}x-\dfrac{1}{2}\)
\(\Rightarrow\dfrac{1}{2}x-\dfrac{3}{4}x=-\dfrac{1}{2}-\dfrac{5}{6}\)
\(\Rightarrow-\dfrac{1}{4}x=-\dfrac{4}{3}\)
\(\Rightarrow x=\dfrac{16}{3}\)
\(h,x-4=\dfrac{5}{6}\left(6-\dfrac{6}{5}x\right)\)
\(\Rightarrow x-4=5-x\)
\(\Rightarrow x+x=5+4\)
\(\Rightarrow2x=9\)
\(\Rightarrow x=\dfrac{9}{2}\)
\(k,7x^2-11=6x^2-2\)
\(\Rightarrow7x^2-6x^2=-2+11\)
\(\Rightarrow x^2=9\Rightarrow\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\)
\(m,5\left(x+3\cdot2^3\right)=10^2\)
\(\Rightarrow5\left(x+24\right)=100\)
\(\Rightarrow x+24=20\)
\(\Rightarrow x=-4\)
\(n,\dfrac{4}{9}-\left(\dfrac{1}{6^2}\right)=\dfrac{2}{3}\left(x-\dfrac{2}{3}\right)^2+\dfrac{5}{12}\)
\(\Rightarrow\dfrac{2}{3}\left(x-\dfrac{2}{3}\right)^2+\dfrac{5}{12}=\dfrac{4}{9}-\dfrac{1}{36}\)
\(\Rightarrow\dfrac{2}{3}\left(x-\dfrac{2}{3}\right)^2+\dfrac{5}{12}=\dfrac{5}{12}\)
\(\Rightarrow\dfrac{2}{3}\left(x-\dfrac{2}{3}\right)^2=0\)
\(\Rightarrow x-\dfrac{2}{3}=0\Rightarrow x=\dfrac{2}{3}\)
#\(Urushi\text{☕}\)
Đúng 4
Bình luận (0)
a: 3-x=x+1,8
=>-2x=-1,2
=>x=0,6
b: 2x-5=7x+35
=>-5x=40
=>x=-8
c: 2(x+10)=3(x-6)
=>3x-18=2x+20
=>x=38
d; 8(x-3/8)+1=6(1/6+x)+x
=>8x-3+1=1+6x+x
=>8x-2=7x+1
=>x=3
e: =>-3x+x=4/3-2/9
=>-2x=12/9-2/9=10/9
=>x=-5/9
g: =>3/4x-1/2x=5/6+1/2
=>1/4x=5/6+3/6=8/6=4/3
=>x=4/3*4=16/3
h: =>x-4=-x+5
=>2x=9
=>x=9/2
Đúng 2
Bình luận (0)
\(\left(\dfrac{3}{\left(x-3\right)^2}+\dfrac{6}{x^2-9}+\dfrac{x-3}{\left(x+3\right)^2}\right)\left(1:\left(\dfrac{24x^2}{x^4-81}-\dfrac{12}{x^2+9}\right)\right)\)
Nhờ mn giúp mình rút gọn với ạ
Bạn ơi mik ra \(\dfrac{x^3+45x-54}{12\left(x-3\right)\left(x+3\right)}\) có đúng không bạn?
Đúng 1
Bình luận (2)
\(\left(6\right)\dfrac{3\sqrt{x}}{5\sqrt{x}-1}\le-3\)
\(\left(7\right)\dfrac{8\sqrt{x}+8}{6\sqrt{x}+9}>\dfrac{8}{3}\)
\(\left(8\right)\dfrac{\sqrt{x}-2}{2\sqrt{x}-3}< -4\)
\(\left(9\right)\dfrac{4\sqrt{x}+6}{5\sqrt{x}+7}\le-\dfrac{2}{3}\)
\(\left(10\right)\dfrac{6\sqrt{x}-2}{7\sqrt{x}-1}>-6\)
6:ĐKXĐ: x>=0; x<>1/25
BPT=>\(\dfrac{3\sqrt{x}}{5\sqrt{x}-1}+3< =0\)
=>\(\dfrac{3\sqrt{x}+15\sqrt{x}-5}{5\sqrt{x}-1}< =0\)
=>\(\dfrac{18\sqrt{x}-5}{5\sqrt{x}-1}< =0\)
=>\(\dfrac{1}{5}< \sqrt{x}< =\dfrac{5}{18}\)
=>\(\dfrac{1}{25}< x< =\dfrac{25}{324}\)
7:
ĐKXĐ: x>=0
BPT \(\Leftrightarrow\dfrac{\sqrt{x}+1}{2\sqrt{x}+3}>\dfrac{8}{3}:\dfrac{8}{3}=1\)
=>\(\dfrac{\sqrt{x}+1}{2\sqrt{x}+3}-1>=0\)
=>\(\dfrac{\sqrt{x}+1-2\sqrt{x}-3}{2\sqrt{x}+3}>=0\)
=>\(-\sqrt{x}-2>=0\)(vô lý)
8:
ĐKXĐ: x>=0; x<>9/4
BPT \(\Leftrightarrow\dfrac{\sqrt{x}-2}{2\sqrt{x}-3}+4< 0\)
=>\(\dfrac{\sqrt{x}-2+8\sqrt{x}-12}{2\sqrt{x}-3}< 0\)
=>\(\dfrac{9\sqrt{x}-14}{2\sqrt{x}-3}< 0\)
TH1: 9căn x-14>0 và 2căn x-3<0
=>căn x>14/9 và căn x<3/2
=>14/9<căn x<3/2
=>196/81<x<9/4
TH2: 9căn x-14<0 và 2căn x-3>0
=>căn x>3/2 hoặc căn x<14/9
mà 3/2<14/9
nên trường hợp này Loại
9:
ĐKXĐ: x>=0
\(BPT\Leftrightarrow\dfrac{2\sqrt{x}+3}{5\sqrt{x}+7}< =-\dfrac{1}{3}\)
=>\(\dfrac{2\sqrt{x}+3}{5\sqrt{x}+7}+\dfrac{1}{3}< =0\)
=>\(\dfrac{6\sqrt{x}+9+5\sqrt{x}+7}{3\left(5\sqrt{x}+7\right)}< =0\)
=>\(\dfrac{11\sqrt{x}+16}{3\left(5\sqrt{x}+7\right)}< =0\)(vô lý)
10:
ĐKXĐ: x>=0; x<>1/49
\(BPT\Leftrightarrow\dfrac{6\sqrt{x}-2}{7\sqrt{x}-1}+6>0\)
=>\(\dfrac{6\sqrt{x}-2+42\sqrt{x}-6}{7\sqrt{x}-1}>0\)
=>\(\dfrac{48\sqrt{x}-8}{7\sqrt{x}-1}>0\)
=>\(\dfrac{6\sqrt{x}-1}{7\sqrt{x}-1}>0\)
TH1: 6căn x-1>0 và 7căn x-1>0
=>căn x>1/6 và căn x>1/7
=>căn x>1/6
=>x>1/36
TH2: 6căn x-1<0 và 7căn x-1<0
=>căn x<1/6 và căn x<1/7
=>căn x<1/7
=>0<=x<1/49
Đúng 0
Bình luận (1)
Giải phương trình:
1, left(x+3right)left(3x^4+8x^2+12x+21right)5left(x^2+1right)^3
2, 3left(x^2+2x-1right)^2-2left(x^2+3x-1right)^2+5x^20
3, dfrac{x^2+x+1}{x+1}+dfrac{x^2+2x+2}{x+2}-dfrac{x^2+3x+3}{x+3}-dfrac{x^2+4x+4}{x+4}0
4, left(dfrac{x+6}{x-6}right)left(dfrac{x+4}{x-4}right)^2+left(dfrac{x-6}{x+6}right)left(dfrac{x+9}{x-9}right)^22.dfrac{x^2+36}{x^2-36}
Đọc tiếp
Giải phương trình:
1, \(\left(x+3\right)\left(3x^4+8x^2+12x+21\right)=5\left(x^2+1\right)^3\)
2, \(3\left(x^2+2x-1\right)^2-2\left(x^2+3x-1\right)^2+5x^2=0\)
3, \(\dfrac{x^2+x+1}{x+1}+\dfrac{x^2+2x+2}{x+2}-\dfrac{x^2+3x+3}{x+3}-\dfrac{x^2+4x+4}{x+4}=0\)
4, \(\left(\dfrac{x+6}{x-6}\right)\left(\dfrac{x+4}{x-4}\right)^2+\left(\dfrac{x-6}{x+6}\right)\left(\dfrac{x+9}{x-9}\right)^2=2.\dfrac{x^2+36}{x^2-36}\)
a/ \(\left(x+3\right)\left(3\left(x^2+1\right)^2+2\left(x+3\right)^2\right)=5\left(x^2+1\right)^3\)
\(\Leftrightarrow3\left(x+3\right)\left(x^2+1\right)^2+2\left(x+3\right)^3-5\left(x^2+1\right)^3=0\)
\(\Leftrightarrow3\left(x+3\right)\left(x^2+1\right)^2-3\left(x^2+1\right)^3+2\left(x+3\right)^3-2\left(x^2+1\right)^3=0\)
\(\Leftrightarrow3\left(x^2+1\right)^2\left(-x^2+x+2\right)+2\left(-x^2+x+2\right)\left(\left(x+3\right)^2+\left(x+3\right)\left(x^2+1\right)+\left(x^2+1\right)^2\right)=0\)
\(\Leftrightarrow\left(-x^2+x+2\right)\left[3\left(x^2+1\right)^2+2\left(x+3+\dfrac{x^2+1}{2}\right)^2+\dfrac{3\left(x^2+1\right)^2}{4}\right]=0\)
\(\Leftrightarrow-x^2+x+2=0\) (phần ngoặc phía sau luôn dương)
\(\Rightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)
Đúng 0
Bình luận (0)
b/ \(3\left(x^2+2x-1\right)^2-2\left(x^2+3x-1\right)^2+5\left(x^2+3x-1-\left(x^2+2x-1\right)\right)^2=0\)
Đặt \(\left\{{}\begin{matrix}a=x^2+2x-1\\b=x^2+3x-1\end{matrix}\right.\)
\(3a^2-2b^2+5\left(b-a\right)^2=0\Leftrightarrow8a^2+3b^2-10ab=0\)
\(\Leftrightarrow\left(4a-3b\right)\left(2a-b\right)=0\Leftrightarrow\left[{}\begin{matrix}4a=3b\\2a=b\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4\left(x^2+2x-1\right)=3\left(x^2+3x-1\right)\\2\left(x^2+2x-1\right)=x^2+3x-1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x^2-x-1=0\\x^2+x-1=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{1+\sqrt{5}}{2}\\x=\dfrac{1-\sqrt{5}}{2}\\x=\dfrac{-1+\sqrt{5}}{2}\\x=\dfrac{-1-\sqrt{5}}{2}\end{matrix}\right.\)
Đúng 0
Bình luận (0)
c/ ĐKXĐ: \(x\ne-1;-2;-3;-4\)
\(\dfrac{x^2+x+1}{x+1}-1+\dfrac{x^2+2x+2}{x+2}-1+1-\dfrac{x^2+3x+3}{x+3}+1-\dfrac{x^2+4x+4}{x+4}=0\)
\(\Leftrightarrow\dfrac{x^2}{x+1}+\dfrac{x^2+x}{x+2}-\dfrac{x^2+2x}{x+3}-\dfrac{x^2+3x}{x+4}=0\)
\(\Leftrightarrow x\left(\dfrac{x}{x+1}+\dfrac{x+1}{x+2}-\dfrac{x+2}{x+3}-\dfrac{x+3}{x+4}\right)=0\)
\(\Leftrightarrow x\left(\dfrac{-1}{x+1}-\dfrac{1}{x+2}+\dfrac{1}{x+3}+\dfrac{1}{x+4}\right)=0\)
\(\Leftrightarrow x\left(\dfrac{-2}{\left(x+1\right)\left(x+3\right)}-\dfrac{2}{\left(x+4\right)\left(x+2\right)}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\\left(x+1\right)\left(x+3\right)+\left(x+2\right)\left(x+4\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\2x^2+10x+11=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{-5-\sqrt{3}}{2}\\x=\dfrac{-5+\sqrt{3}}{2}\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Bài 1: GPT
a) \(\dfrac{x+2}{x-2}-\dfrac{x-2}{x+2}=\dfrac{4x^2}{x^2-4}\)
b) \(\dfrac{6}{x-1}-\dfrac{4}{x-3}=\dfrac{8}{\left(x-1\right)\left(x-3\right)}\)
c)\(\dfrac{x+3}{x-3}-\dfrac{48}{x^2-9}=\dfrac{x-3}{x+3}\)
a: ĐKXĐ: x<>2; x<>-2
PT =>(x+2)^2-(x-2)^2=4x^2
=>4x^2=x^2+4x+4-x^2+4x-4=8x
=>4x^2-8x=0
=>4x(x-2)=0
=>x=0(loại) hoặc x=2(loại)
b: ĐKXĐ: x<>1; x<>3
PT =>6x-18-4x+4=8
=>2x-14=8
=>2x=22
=>x=11(nhận)
c: ĐKXĐ: x<>3; x<>-3
PT =>(x+3)^2-48=(x-3)^2
=>x^2+6x+9-48=x^2-6x+9
=>12x=48
=>x=4(nhận)
Đúng 0
Bình luận (0)
Rút gọn \(\left[\dfrac{x}{2x-6}-\dfrac{x^2}{x^2-9}+\dfrac{x}{2x-9}.\left(\dfrac{3}{x}-\dfrac{1}{x-3}\right)\right]:\dfrac{x^2-5x-6}{18-2x^2}\)
ĐKXĐ: \(x\ne\pm3,x\ne\dfrac{9}{2}\)
= \(\left[\dfrac{x}{2\left(x-3\right)}-\dfrac{x^2}{\left(x-3\right)\left(x+3\right)}+\dfrac{x}{2x-9}.\dfrac{3\left(x-3\right)-x}{x\left(x-3\right)}\right]\) : \(\dfrac{x^2-5x-6}{-2\left(x-3\right)\left(x+3\right)}\)
= \(\left[\dfrac{x}{2\left(x-3\right)}-\dfrac{x^2}{\left(x-3\right)\left(x+3\right)}+\dfrac{1}{x-3}\right]:\dfrac{-\left(x^2-5x-6\right)}{2\left(x-3\right)\left(x+3\right)}\)
= \(\dfrac{x\left(x+3\right)-2x^2+2\left(x+3\right)}{2\left(x-3\right)\left(x+3\right)}:\dfrac{-\left(x^2-5x-6\right)}{2\left(x-3\right)\left(x+3\right)}\)
= \(\dfrac{-2\left(x^2-5x-6\right)\left(x-3\right)\left(x+3\right)}{-2\left(x^2-5x-6\right)\left(x-3\right)\left(x+3\right)}=1\)
Đúng 0
Bình luận (0)
Rút gọn:
\(A=\left[\left(\dfrac{3}{1+x}-\dfrac{x}{x^2+x+1}\right):\dfrac{2x^2+3x}{x+1}+\dfrac{3}{x+1}\right]\cdot\dfrac{x^2+x}{1+3x}\)
\(B=\left[\dfrac{a}{2a-6}-\dfrac{a^2}{a^2-9}+\dfrac{a}{2a-9}\cdot\left(\dfrac{3}{a}+\dfrac{1}{3-a}\right)\right]:\dfrac{a^2-5a-6}{18-2a^2}\)