5x+y^2 phần x^2y -5y-x^2 phần xy^2
Bài 6: Phép trừ các phân thức đại số
\(\dfrac{5x+y^2}{x^2y}-\dfrac{5y+x^2}{xy^2}\)
\(=\dfrac{y\left(5x+y^2\right)}{x^2y^2}-\dfrac{x\left(5y-x^2\right)}{x^2y^2}\)
\(=\dfrac{5xy+y^3}{x^2y^2}-\dfrac{5xy+x^3}{x^2y^2}\)
\(=\dfrac{5xy+y^3-5xy+x^3}{x^2y^2}\)
\(=\dfrac{\left(5xy-5xy\right)+x^3+y^3}{x^2y^2}\)
\(=\dfrac{x^3+y^3}{x^2y^2}\)
Đúng 1
Bình luận (0)
\(\dfrac{5x+y^2}{x^2y}-\dfrac{5y-x^2}{xy^2}=\dfrac{y\left(5x+y^2\right)}{y\cdot x^2y}-\dfrac{x\left(5y-x^2\right)}{x\cdot xy^2}\)
\(=\dfrac{5xy+y^3-5xy+x^3}{x^2y^2}=\dfrac{x^3+y^3}{x^2y^2}\) \(\left(x,y\ne0\right)\)
Đúng 0
Bình luận (0)
5x-5/(x+1)^2 : 20x^2-20/3x+3
\(\dfrac{5x-5}{\left(x+1\right)^2}:\dfrac{20x^2-20}{3x+3}\\ =\dfrac{5\left(x-1\right)}{\left(x+1\right)^2}:\dfrac{20\left(x^2-1\right)}{3\left(x+1\right)}\\ =\dfrac{5\left(x-1\right)}{\left(x+1\right)^2}\cdot\dfrac{3\left(x+1\right)}{20\left(x^2-1\right)}\\ =\dfrac{3}{4\left(x+1\right)^2}\)
Đúng 3
Bình luận (1)
5x-5/(x+1)^2 - 20x^2-20/3x+3
\(\dfrac{5x-5}{\left(x+1\right)^2}-\dfrac{20x^2-20x}{3x+3}\\ =\dfrac{5\left(x-1\right)}{\left(x+1\right)^2}-\dfrac{20x\left(x-1\right)}{3\left(x+1\right)}\\ =\dfrac{15\left(x-1\right)}{3\left(x+1\right)^2}-\dfrac{20x\left(x^2-1\right)}{3\left(x+1\right)^2}\\ =\dfrac{15x-15-20x^3+20x}{3\left(x+1\right)^2}\\ =\dfrac{-20x^3+35x-15}{3\left(x+1\right)^2}\)
Đúng 2
Bình luận (0)
Thực hiện phép tính: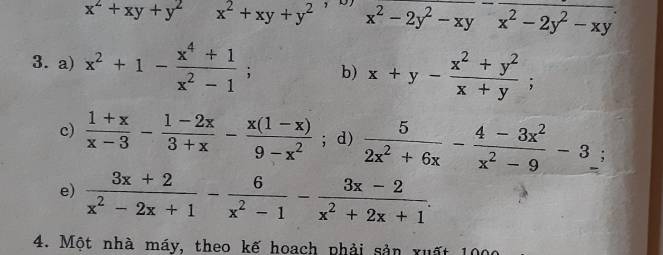
a: \(=\dfrac{\left(x^2+1\right)\left(x^2-1\right)-x^4-1}{x^2-1}\)
\(=\dfrac{x^4-1-x^4-1}{x^2-1}=-\dfrac{2}{x^2-1}\)
b: \(=\dfrac{\left(x+y\right)^2-x^2-y^2}{x+y}\)
\(=\dfrac{x^2+2xy+y^2-x^2-y^2}{x+y}=\dfrac{2xy}{x+y}\)
c: \(=\dfrac{\left(x+1\right)\left(x+3\right)+\left(2x-1\right)\left(x-3\right)+x\left(1-x\right)}{x^2-9}\)
\(=\dfrac{x^2+4x+3+2x^2-7x+3+x-x^2}{x^2-9}\)
\(=\dfrac{2x^2-2x+6}{x^2-9}\)
d: \(\dfrac{5}{2x^2+6x}-\dfrac{4-3x^2}{x^2-9}-3\)
\(=\dfrac{5}{2x\left(x+3\right)}+\dfrac{3x^2-4}{\left(x-3\right)\left(x+3\right)}-3\)
\(=\dfrac{5\left(x-3\right)+2x\left(3x^2-4\right)-3\cdot2x\left(x^2-9\right)}{2x\left(x+3\right)\left(x-3\right)}\)
\(=\dfrac{5x-15+6x^3-8x-6x^3+54x}{2x\left(x+3\right)\left(x-3\right)}\)
\(=\dfrac{51x-15}{2x\left(x+3\right)\left(x-3\right)}\)
Đúng 1
Bình luận (0)
e: \(=\dfrac{3x+2}{\left(x-1\right)^2}-\dfrac{6}{\left(x-1\right)\left(x+1\right)}-\dfrac{3x-2}{\left(x+1\right)^2}\)
\(=\dfrac{\left(3x+2\right)\left(x+1\right)^2-6\left(x^2-1\right)-\left(3x-2\right)\left(x-1\right)^2}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{\left(3x+2\right)\left(x+1\right)\left(x+1\right)-\left(3x-2\right)\left(x-1\right)\left(x-1\right)-6\left(x^2-1\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{\left(3x^2+5x+2\right)\left(x+1\right)-\left(3x^2-5x+2\right)\left(x-1\right)-6\left(x^2-1\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{3x^3+8x^2+7x+2-3x^3+8x^2-7x+2-6x^2+6}{\left(x-1\right)^2\left(x+1\right)^2}\)
\(=\dfrac{10x^2+10}{\left(x-1\right)^2\left(x+1\right)^2}\)
Đúng 1
Bình luận (0)
Giải phương trình x+1/2021+x+2/2020+x+3/2019+x+2028/2=0
=>\(\left(\dfrac{x+1}{2021}+1\right)+\left(\dfrac{x+2}{2020}+1\right)+\left(\dfrac{x+3}{2019}+1\right)+\left(\dfrac{x+2028}{2}-3\right)=0\)
=>x+2022=0
=>x=-2022
Đúng 0
Bình luận (0)
câu 1: tháng trc 2 tổ sản xuất đc 5000 sản phẩm, tháng này tổ 1 tăng 10%, tổ 2 tăng 5% nên tháng này cả 2 tổ sản xuất đc 5400 sản phẩm. hỏi tháng trc mỗi tổ sản xuất đc bao nhiêu sản phẩm?câu 2: 1 cửa hàng điện máy thực hiện chương trình khuyến mãi giảm tất cả các mặt hàng 10% theo giá niêm yết. Nếu khách hàng mua hàng trên 10tr đc giảm thêm 2% số tiền, mua trên 15tr giảm thêm 4% số tiền, mua trên 40tr đc giảm thêm 8% số tiền. ông A mua TV giá niêm yết là 9.200.000đ và tủ lạnh giá niêm yết là 7....
Đọc tiếp
câu 1: tháng trc 2 tổ sản xuất đc 5000 sản phẩm, tháng này tổ 1 tăng 10%, tổ 2 tăng 5% nên tháng này cả 2 tổ sản xuất đc 5400 sản phẩm. hỏi tháng trc mỗi tổ sản xuất đc bao nhiêu sản phẩm?
câu 2: 1 cửa hàng điện máy thực hiện chương trình khuyến mãi giảm tất cả các mặt hàng 10% theo giá niêm yết. Nếu khách hàng mua hàng trên 10tr đc giảm thêm 2% số tiền, mua trên 15tr giảm thêm 4% số tiền, mua trên 40tr đc giảm thêm 8% số tiền. ông A mua TV giá niêm yết là 9.200.000đ và tủ lạnh giá niêm yết là 7.100.000đ. hỏi với chương trình khuyến mãi của cửa hàng ông A phải trả bao nhiêu tiền?
câu 3: có 2 thùng dầu thùng a chứa số lượng dầu gấp 4 lần lượng dầu ở thùng b. nếu lấy bớt ở thùng a ra 40 lít dầu và đổ vào thùng b 20 lít dầu thì lượng dầu trg 2 thùng = nhau. tính số lít dầu trg mỗi thùng lúc đầu
câu 4: lớp 8a có 45 học sinh để chuẩn bị cho buổi tham quan học tập. tập thể lớp 8a sau khi bàn bạc đã quyết định đặt mua cty may mặc đồng phục tham quan cho lớp. mỗi bạn đc đặt một bộ. mỗi bộ đồng phục nam có giá 120000đ mỗi bộ đồng phục nữ có giá 100000đ. tổng số tiền lớp phải trả cho cty may là 4 900 000đ. tính số HS nam và HS nữ lớp 8a
Câu 4:
Gọi số học sinh nam, số học sinh nữ lần lượt là a,b
Theo đề,ta có: a+b=45 và 120000a+100000b=4900000
=>a=20 và b=25
Cau 3:
Gọi thể tích dầu ban đầu ở thùng A và thùng B lần lượt là a,b
Theo đề, ta có:
a=4b và a-40=b+20
=>a-4b=0 và a-b=60
=>a=80 và b=20
Đúng 0
Bình luận (0)
câu 1: mẹ sinh ra An lúc 28t. hỏi An bao nhiêu tuổi bt rằng tuổi mẹ gấp 3 lần tuổi An?
câu 2: bác 4 mua 1 thùng trái cây gồm táo và xoài. trg đó táo 30.000đ/kg, xoài 50.000đ/kg. hỏi bác 4 mua bao nhiêu kg táo, bao nhiêu kg xoài biết giá tiền thùng trái cây là 500.000đ
Câu 1:
Hiệu số phần bằng nhau:
3-1=2(phần)
Tuổi mẹ hiện nay:
28:2 x 3= 42(tuổi)
Tuổi An hiện nay:
42:3=14(tuổi)
Đúng 0
Bình luận (0)
Câu 2: TH1: 5 kí táo và 7 kí xoài
TH2: 10 kí táo và 4 kí xoài
TH3: 15 kí táo và 1 kí xoài
Đúng 0
Bình luận (0)
ai giải thích giúp vì sao -x-x=2x ko
-2x thì làm sao bằng 2x được bạn, trừ khi x=0
Đúng 0
Bình luận (0)
Thực hiện phép tính:
\(\dfrac{x+7}{x-7}-\dfrac{x-7}{x+7}+\dfrac{4x^2}{x^2-49}\)
\(đk:x\ne7;-7\\ =\dfrac{x+7}{x-7}-\dfrac{x-7}{x+7}+\dfrac{4x^2}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{\left(x+7\right)^2-\left(x-7\right)^2+4x^2}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{x^2+14x+49-x^2+14x-49+4x^2}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{4x^2+28x}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{4x\left(x+7\right)}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{4x}{x-7}\)
Đúng 1
Bình luận (0)
\(\dfrac{x+7}{x-7}-\dfrac{x-7}{x+7}+\dfrac{4x^2}{x^2-49}\left(x\ne\pm7\right)\)
\(=\dfrac{\left(x+7\right)^2}{x^2-49}-\dfrac{\left(x-7\right)^2}{x^2-49}+\dfrac{4x^2}{x^2-49}\)
\(=\dfrac{x^2+14x+49-x^2+14x-49+4x^2}{x^2-49}\)
\(=\dfrac{4x^2+28x}{x^2-49}=\dfrac{4x\left(x+7\right)}{\left(x+7\right)\left(x-7\right)}=\dfrac{4x}{x-7}\)
Đúng 1
Bình luận (0)
\(\dfrac{x+7}{x-7}-\dfrac{x-7}{x+7}+\dfrac{4x^2}{x^2-49}\\ =\dfrac{x+7}{x-7}-\dfrac{x-7}{x+7}+\dfrac{4x^2}{\left(x-7\right)\left(x+7\right)}\)
\(=\dfrac{\left(x+7\right)^2}{\left(x-7\right)\left(x+7\right)}-\dfrac{\left(x-7\right)^2}{\left(x-7\right)\left(x+7\right)}+\dfrac{4x^2}{\left(x-7\right)\left(x+7\right)}\)
\(=\dfrac{x^2+14x+49-x^2+14x-49+4x^2}{\left(x-7\right)\left(x+7\right)}\)
\(=\dfrac{x^2-x^2+4x^2+14x+14x+49-49}{\left(x-7\right)\left(x+7\right)}\)
\(=\dfrac{4x^2+28x}{\left(x-7\right)\left(x+7\right)}\)
\(=\dfrac{4x\left(x+7\right)}{\left(x-7\right)\left(x+7\right)}\\ =\dfrac{4x}{x-7}\)
Đúng 0
Bình luận (0)
Thực hiện phép trừ
\(\dfrac{x^2-8}{4x-8}-\dfrac{x-3}{x-2}\)
\(đk:x\ne2\\ =\dfrac{x^2-8}{4x-8}-\dfrac{x-3}{x-2}\\ =\dfrac{x^2-8}{4\left(x-2\right)}-\dfrac{x-3}{x-2}\\ =\dfrac{x^2-8-4\left(x-3\right)}{4\left(x-2\right)}\\ =\dfrac{x^2-8-4x+12}{4\left(x-2\right)}\\ =\dfrac{x^2-4x+4}{4\left(x-2\right)}\\ =\dfrac{\left(x-2\right)^2}{4\left(x-2\right)}\\ =\dfrac{x-2}{4}\)
Đúng 0
Bình luận (0)





