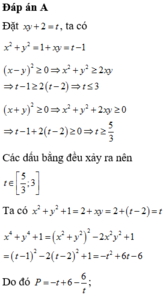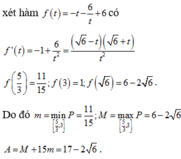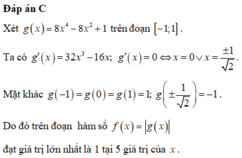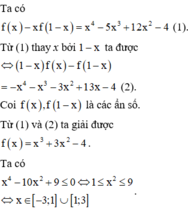x4+y4+2x2y2-10x2-9y2+9=0. Tìm giá trị nhỏ nhất của A=x2+y2
DL
Những câu hỏi liên quan
Cho x, y là những số thực thỏa mãn
x
2
–
x
y
+
y
2
1
. Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của
P
x
4
+
y
4
+
1
x...
Đọc tiếp
Cho x, y là những số thực thỏa mãn x 2 – x y + y 2 = 1 . Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của P = x 4 + y 4 + 1 x 2 + y 2 + 1 . Giá trị của A = M + 15 m là
A. A = 17 - 2 6
B. A = 17 - 6
C. A = 17 + 6
D. A = 17 + 2 6
Cho x,y là những số thực thỏa mãn
x
2
-
x
y
+
y
2
1
. Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của
P
x
4
+
y
4
+
1
x
2
+
y...
Đọc tiếp
Cho x,y là những số thực thỏa mãn x 2 - x y + y 2 = 1 . Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của P = x 4 + y 4 + 1 x 2 + y 2 + 1 . Giá trị của A = M + 15m là
A. A = 17 - 2 6
B. A = 17 + 6
C. A = 17 + 2 6
D. A = 17 - 6
cho hai số x,y thỏa mãn x2 + y2 =1 + xy , gọi M và m lần lượt là giá trị lớn nhất , giá trị nhỏ nhất của P = x4 + y4 -x2y2 , tính tích Mm
\(x^2+y^2=1+xy\Rightarrow x^2+y^2-xy=1\)
Ta có: \(1+xy=x^2+y^2\ge2xy\Rightarrow xy\le1\)
\(1+xy=x^2+y^2\ge-2xy\Rightarrow xy\ge-\dfrac{1}{3}\)
\(P=\left(x^2+y^2\right)^2-x^2y^2-2x^2y^2=\left(x^2+y^2-xy\right)\left(x^2+y^2+xy\right)-2x^2y^2\)
\(=x^2+y^2+xy-2x^2y^2=-2x^2y^2+2xy+1\)
Đặt \(a=xy\Rightarrow P=f\left(a\right)=-2a^2+2a+1\)
Xét hàm \(f\left(a\right)=-2a^2+2a+1\) trên \(\left[-\dfrac{1}{3};1\right]\)
\(-\dfrac{b}{2a}=\dfrac{1}{2}\in\left[-\dfrac{1}{3};1\right]\)
\(f\left(-\dfrac{1}{3}\right)=\dfrac{1}{9}\) ; \(f\left(\dfrac{1}{2}\right)=\dfrac{3}{2}\) ; \(f\left(1\right)=1\)
\(\Rightarrow M=\dfrac{3}{2}\) ; \(m=\dfrac{1}{9}\) \(\Rightarrow Mm=\dfrac{1}{6}\)
Đúng 4
Bình luận (0)
Bài 7 : hoàn thiện các hằng đẳng thức sau
a. .... - 10x + 25x = ( x - .... )2
b. .... - 4x2 + x4 = ( .... - x2 )2
c. x2 - .... + 9y2 = ( x - ....)2
d. ( 2x + ....) ( .... - y2 ) = 4x2 - y4
a. \(x^2-10x+25=\left(x-5\right)^2\)
b.\(4-4x^2+x^4=\left(2-x^2\right)^2\)
c. \(x^2-6y+9y^2=\left(x-3y\right)^2\)
d. \(\left(2x+y^2\right)\left(2x-y^2\right)=4x^2-y^4\)
Đúng 1
Bình luận (0)
a) x2 - 10x + 25x = ( x - 5)2
b) 4 - 4x2 + x4 = ( 2 - x2 )2
c) x2 - 6xy + 9y2 = (x - 3y )2
d_ (2x + y2 ). (2x - y2 ) = 4x2 - y4
Đúng 0
Bình luận (2)
Tìm x
(x-5)2=(3+2x)2
27x3-54x2+36x=9
cho bt x-y=4 và xy=1 tính giá trị của các biểu thức A=x2+y2,B=x3-y3,C=x4+y4
a) \(\left(x-5\right)^2=\left(3+2x\right)^2\)
\(\Rightarrow\left(3+2x\right)^2-\left(x-5\right)^2=0\)
\(\Rightarrow\left(3+2x+x-5\right)\left(3+2x-x+5\right)=0\)
\(\Rightarrow\left(3x-2\right)\left(x+8\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}3x-2=0\\x+8=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-8\end{matrix}\right.\)
b) \(27x^3-54x^2+36x=9\)
\(\Rightarrow27x^3-54x^2+36x-9=0\)
\(\Rightarrow27x^3-54x^2+36x-8+8-9=0\)
\(\Rightarrow\left(3x-2\right)^3-1=0\)
\(\Rightarrow\left(3x-2-1\right)\left[\left(3x-2\right)^2+3x-2+1\right]=0\)
\(\Rightarrow\left(3x-3\right)\left[\left(3x-2\right)^2+3x-2+\dfrac{1}{4}-\dfrac{1}{4}+1\right]=0\)
\(\Rightarrow\left(3x-3\right)\left[\left(3x-2+\dfrac{1}{2}\right)^2+\dfrac{3}{4}\right]=0\)
\(\Rightarrow\left(3x-3\right)\left[\left(3x-\dfrac{3}{2}\right)^2+\dfrac{3}{4}\right]=0\left(1\right)\)
mà \(\left(3x-\dfrac{3}{2}\right)^2+\dfrac{3}{4}>0,\forall x\)
\(\left(1\right)\Rightarrow3x-3=0\Rightarrow3x=3\Rightarrow x=1\)
Đúng 2
Bình luận (0)
(\(x-5\))2 = (3 +2\(x\))2 ⇒ \(\left[{}\begin{matrix}x-5=3+2x\\x-5=-3-2x\end{matrix}\right.\) ⇒ \(\left[{}\begin{matrix}x=-8\\x=\dfrac{2}{3}\end{matrix}\right.\) vậy \(x\in\){-8; \(\dfrac{2}{3}\)}
27\(x^3\) - 54\(x^2\) + 36\(x\) = 9
27\(x^3\) - 54\(x^2\) + 36\(x\) - 8 = 1
(3\(x\) - 2)3 = 1 ⇒ 3\(x\) - 2 = 1 ⇒ \(x\) = 1
Đúng 2
Bình luận (0)
Xem thêm câu trả lời
Cho
f
x
là hàm đa thức thỏa mãn
f
x
-
x
f
1
-
x
x
4
-
5
x
3
+
12
x
2
-
4
∀
x
∈
ℝ
. Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất...
Đọc tiếp
Cho f x là hàm đa thức thỏa mãn f x - x f 1 - x = x 4 - 5 x 3 + 12 x 2 - 4 ∀ x ∈ ℝ . Gọi M và m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của hàm số y = f x trên tập D = x ∈ ℝ | x 4 - 10 x 2 + 9 ≤ 0 . Giá trị của 21 m + 6 M + 2019 bằng
A. 2235.
B. 2319.
C. 3045.
D. 3069.
chứng minh giá trị biểu thức sau không phụ thuộc vào giá trị của biến:
a, A = y (x2 - y2) (x2 + y2) - y (x4 - y4)
b, B = (x - 1)3 - (x - 1) (x2 + x + 1) - 3 (1 - x) x
a) \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)=0\)
b) \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3\left(1-x\right)x=x^3-3x^2+3x-1-x^3-x^2-x+x^2+x+1-3x+3x^2=0\)
Đúng 1
Bình luận (0)
a: Ta có: \(A=y\left(x^2-y^2\right)\left(x^2+y^2\right)-y\left(x^4-y^4\right)\)
\(=y\left(x^4-y^4\right)-y\left(x^4-y^4\right)\)
=0
b: Ta có: \(B=\left(x-1\right)^3-\left(x-1\right)\left(x^2+x+1\right)-3x\left(1-x\right)\)
\(=x^3-3x^2+3x-1-x^3+1-3x+3x^2\)
=0
Đúng 0
Bình luận (0)
1, Cho biết x+y=15 và xy=50. Tính giá trị của các biểu thức:
a. A=x2+y2
b. B=x4+y4
c. C=x2-y2
2, Cho biết x-y=15 và xy=50. Hãy tính x2+y2 ; x2-y2 rồi từ đó suy ra kết quả của x4-y4.
a: \(A=x^2+y^2=\left(x+y\right)^2-2xy=15^2-2\cdot50=115\)
c: \(x-y=\sqrt{\left(x+y\right)^2-4xy}=\sqrt{15^2-4\cdot50}=5\)
\(C=x^2-y^2=\left(x+y\right)\left(x-y\right)=15\cdot5=75\)
Đúng 0
Bình luận (0)
Cho biết x + y 15 và xy 50. Tính giá trị của các biểu thức:a) A x2 + y2b) B x4 + y4c) C x2 − y2Nếu thay giả thiết thành x − y 15 và xy 50. Hãy tính x2 + y2; x2 − y2. Từ đó suy ra kết quả của x4 − y4.
Đọc tiếp
Cho biết x + y = 15 và xy = 50. Tính giá trị của các biểu thức:
a) A = x2 + y2
b) B = x4 + y4
c) C = x2 − y2
Nếu thay giả thiết thành x − y = 15 và xy = 50. Hãy tính x2 + y2; x2 − y2. Từ đó suy ra kết quả của x4 − y4.
a: \(A=x^2+y^2=\left(x+y\right)^2-2xy=15^2-2\cdot50=125\)
b:\(B=x^4+y^4\)
\(=\left(x^2+y^2\right)^2-2x^2y^2\)
\(=125^2-2\cdot2500\)
=10625
c: \(x-y=\sqrt{\left(x+y\right)^2-4xy}=\sqrt{15^2-4\cdot50}=5\)
\(C=x^2-y^2=\left(x-y\right)\left(x+y\right)=15\cdot5=75\)
Đúng 0
Bình luận (0)
Cho x+y=a , xy=b . Tính giá trị của các biểu thức sau theo giá trị của a và b: a) x2+y2 ; b) x3+y3 ; c) x4+y4 ; d) x5+y5
\(\text{a) x^2 + y^2 = (x+y)^2 - 2xy = a^2 - 2b}\)
\(\text{b) x^3 + y^3 = (x+y)^3 - 3xy(x+y) = a^3 - 3ab}\)
\(\text{c) x^4 + y^4 = (x^2+y^2)^2 - 2x^2y^2 = (a^2-2b)^2 - 2b^2 = a^4 - 4a^2b + 2b^2}\)
\(\text{d) x^5 + y^5 = (x^3+y^3)(x^2+y^2) - x^2y^2(x+y) = a^5 - 5a^3b + 5ab^2}\)
Đúng 1
Bình luận (0)