Giúp mình bài 6,7 với ạ mình cảm ơn nhiều
VT
Những câu hỏi liên quan
Giải hộ mình câu 6,7 với ạ. cảm ơn mn nhiều
bài 7
\(x^{n+2}-x^n\)
\(=x^{n+2-n}=x^2\)
\(\left(b\right)x^{x+3}-x^{x+1}=x^{x+3-x-1}=X^2\)
c)
\(x^{2m}+x^m=x^{2m+m}=x^{2m}\)
d)
\(x^{2n+1}-x^{4n}=x^{2n+1-4n}=x^{1-2n}\)
Đúng 1
Bình luận (0)
Giúp mình bài 6,7 với ạ mình cảm ơnnn
Bài 6.
a)Công suất ấm: \(P=\dfrac{A}{t}=\dfrac{900\cdot1000}{10\cdot60}=1500W\)
Dòng điện qua ấm: \(I=\dfrac{P}{U}=\dfrac{1500}{220}=\dfrac{75}{11}A\)
Điện trở dây nung: \(R=\dfrac{U}{I}=\dfrac{220}{\dfrac{75}{11}}=\dfrac{484}{15}\Omega\)
b)Điện năng tiêu thụ trong 1 tháng (30 ngày):
\(T=900\cdot1000\cdot30\cdot3600=9,72\cdot10^{10}J=27000kWh\)
Tiền điện phải trả: \(T=27000\cdot1500=40500\left(k.đồng\right)\)
c)Công suất tiêu thụ thực:
\(P=UI=\dfrac{U^2}{R}=\dfrac{110^2}{\dfrac{484}{15}}=375W\)
Đúng 0
Bình luận (0)
Bài 7.
CTM: \(\left(Đ_1ntR_b\right)//Đ_2\)
\(R_1=\dfrac{U_{Đ1}^2}{P_{Đ1}}=\dfrac{10^2}{2}=50\Omega;I_{Đ1đm}=\dfrac{P_{Đ1}}{U_{Đ1}}=\dfrac{2}{10}=0,2A\)
\(R_2=\dfrac{U^2_{Đ2}}{P_{Đ2}}=\dfrac{12^2}{3}=48\Omega;I_{Đ2đm}=\dfrac{P_{Đ2}}{U_{Đ2}}=\dfrac{3}{12}=0,25A\)
Để đèn 1 sáng bình thường \(\Rightarrow I_b=I_{Đ1đm}=0,2A\)
\(R_{Đ1+b}=\dfrac{12}{0,2}=60\Omega\)
\(R_b=60-R_{Đ1}=60-50=10\Omega\)
Đúng 0
Bình luận (0)
Giúp mình bài bài 5 bài 6 với ạ. Mình cần trc 18:40 ạ. Mình cảm ơn nhiều
Câu 5:
a: Xét ΔABC có \(BC^2=AB^2+AC^2\)
nên ΔABC vuông tại A
c: Xét tứ giác AEDF có
\(\widehat{EAF}=\widehat{AFD}=\widehat{AED}=90^0\)
Do đó: AEDF là hình chữ nhật
mà AD là tia phân giác của \(\widehat{FAE}\)
nên AEDF là hình vuông
Đúng 0
Bình luận (0)
Giúp mình bài này với ạ. Mình cảm ơn nhiều
Giúp mình bài này với ạ , mình cảm ơn nhiều 
ai giúp mình bài này với ạ, mình cảm ơn nhiều ![]()

c)\(\left\{{}\begin{matrix}u_1+u_3=3\\u_1^2+u_3^2=5\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_3=3\\\left(u_1+u_3\right)^2-2u_1u_3=5\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_3=3\\u_1u_3=2\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}u_1=2\\u_3=1\end{matrix}\right.\\\left\{{}\begin{matrix}u_1=1\\u_3=2\end{matrix}\right.\end{matrix}\right.\)
Làm nốt (sử dụng công thức: \(u_n=u_1+\left(n-1\right)d\) để tìm được công sai
\(S_n=nu_1+\dfrac{n\left(n-1\right)}{2}d\) để tính tổng 15 số hạng đầu)
d)\(\left\{{}\begin{matrix}u_1+u_2+u_3=14\\u_1u_2u_3=64\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}u_2-d+u_2+u_2+d=14\\\left(u_2-d\right)u_2\left(u_2+d\right)=64\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u_2=\dfrac{14}{3}\\\left(u_2^2-d^2\right)u_2=64\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}\dfrac{14}{3}=u_2=u_1+d\\d=\dfrac{2\sqrt{889}}{21}\end{matrix}\right.\\\left\{{}\begin{matrix}\dfrac{14}{3}=u_1+d\\d=\dfrac{-2\sqrt{889}}{21}\end{matrix}\right.\end{matrix}\right.\)
(Làm nốt,số xấu quá)
e)\(\left\{{}\begin{matrix}u_1+u_2+u_3=7\\u_1^2+u_2^2+u_3^2=21\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u_1+u_2+u_3=7\\u_1u_2u_3=\dfrac{21-\left(u_1+u_2+u_3\right)^2}{2}=-14\end{matrix}\right.\)
Làm như ý d)
Đúng 1
Bình luận (1)

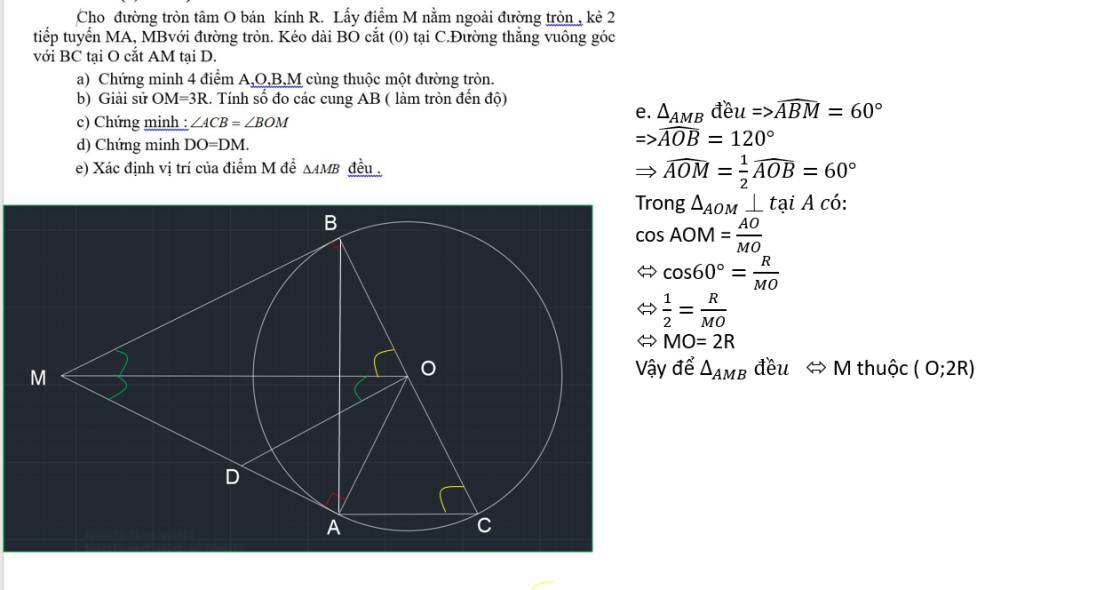
giúp mình bài này với ạ, Mình cảm ơn mn nhiều
a: Xét tứ giác AOBM có
góc OAM+góc OBM=180 độ
=>AOBM nội tiếp
b: \(cosAOM=\dfrac{OA}{OM}=\dfrac{1}{3}\)
nên \(\widehat{AOM}\simeq71^0\)
=>\(\widehat{AOB}\simeq142^0\)
=>sđ cung nhỏ AB là 142 độ; sđ cung lơn AB=360-142=218 độ
c:
Xét (O) có
ΔBAC nội tiếp
BC là đường kính
=>ΔBAC vuông tại A
=>BA vuông góc AC
Xét(O) có
MA,MB là tiêp tuyến
nên MA=MB
mà OA=OB
nên OM là trung trực của AB
=>OM//AC
góc ACB=góc OAC
góc OAC=góc AOM
=>góc ACB=góc AOM=góc BOM
d: góc DOM+góc BOM=90 độ
góc DMO+góc AOM=90 độ
mà góc BOM=góc AOM
nên góc DOM=góc DMO
=>DO=DM
Đúng 1
Bình luận (0)
Ai giúp mình giải tự luận bài này với ạ!! Mình cảm ơn nhiều ạ!
Đề bài là: Tính cos2x
Cảm ơn mn nhiều ạ!
Đúng 0
Bình luận (0)
`sin3x sinx+sin(x-π/3) cos (x-π/6)=0`
`<=> 1/2 (cos2x - cos4x) + 1/2(-sin π/6 + sin (2x-π/2)=0`
`<=> cos2x-cos4x-1/2+ sin(2x-π/2)=0`
`<=>cos2x-cos4x-1/2+ sin2x .cos π/2 - cos2x. sinπ/2=0`
`<=> cos2x - cos4x - cos2x = 1/2`
`<=> cos4x = cos(2π)/3`
`<=>` \(\left[{}\begin{matrix}4x=\dfrac{2\text{π}}{3}+k2\text{π}\\4x=\dfrac{-2\text{π}}{3}+k2\text{π}\end{matrix}\right.\)
`<=>` \(\left[{}\begin{matrix}x=\dfrac{\text{π}}{6}+k\dfrac{\text{π}}{2}\\x=-\dfrac{\text{π}}{6}+k\dfrac{\text{π}}{2}\end{matrix}\right.\)
Đúng 1
Bình luận (1)
\(sin3x.sinx+sin\left(x-\dfrac{\pi}{3}\right)cos\left(x-\dfrac{\pi}{6}\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}cos2x-\dfrac{1}{2}cos4x+\dfrac{1}{2}sin\left(2x-\dfrac{\pi}{2}\right)+\dfrac{1}{2}sin\left(-\dfrac{\pi}{6}\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}cos2x-\dfrac{1}{2}cos4x-\dfrac{1}{2}cos2x-\dfrac{1}{4}=0\)
\(\Leftrightarrow cos4x+\dfrac{1}{2}=0\)
\(\Leftrightarrow2cos^22x-1+\dfrac{1}{2}=0\)
\(\Leftrightarrow cos^22x=\dfrac{1}{4}\)
\(\Rightarrow cos2x=\pm\dfrac{1}{2}\)
Đúng 1
Bình luận (0)
Xem thêm câu trả lời