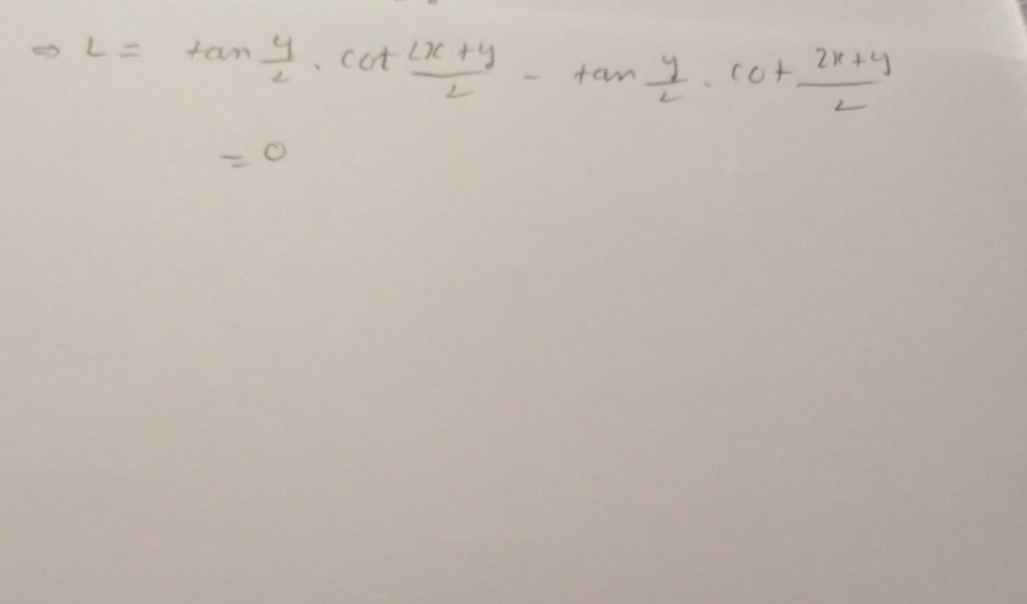Biết sinx+cosx=m . Tính |sin³x-cos³x|. Giúp mình với ạ.
LV
Những câu hỏi liên quan
1) chứng minh:
sin^4 x + sin^2 x * cos^2 x + 3cos^2 x =1+2 sin^ 2 x|
2) cho sinx * cosx =√3/4, tính sinx, cosx, tanx, cotx
em cần gấp trc 7h ạ nên giúp em vs
2: \(\left(sinx+cosx\right)^2=1+2\cdot sinx\cdot cosx=1+2\cdot\dfrac{\sqrt{3}}{4}=1+\dfrac{\sqrt{3}}{2}=\dfrac{2+\sqrt{3}}{2}\)
=>\(sinx+cosx=\dfrac{\sqrt{3}+1}{2}\)
mà sin x*cosx=căn 3/4
nên sinx,cosx là các nghiệm của phương trình là:
\(a^2-\dfrac{\sqrt{3}+1}{2}\cdot a+\dfrac{\sqrt{3}}{4}=0\)
=>\(\left[{}\begin{matrix}a=\dfrac{\sqrt{3}}{2}\\a=\dfrac{1}{2}\end{matrix}\right.\)
Ta sẽ có hai trường hợp:
TH1: sin x=căn 3/2; cosx=1/2
tan x=sinx/cosx=căn 3
cot x=1/căn 3
TH2: sin x=1/2; cosx=căn 3/2
tan x=sin x/cosx=1/căn 3
cot x=1:1/căn 3=căn 3
Đúng 0
Bình luận (0)
sin^3 x +cos^3 x -3sinx cosx+1=0
3 cosx -3sin2x= √3(cos2x+sinx)
4sin^3x +3sin^2x cosx -sinx-cos^3x=0
√3sin4x-cos4x=sinx- √3cosx
m.n giúp mk chứng minh với ạ
Giúp mình giải này với Căn 3 cos mũ 3 x - 5 sin mũ 3 x +7 sinx-8/ cosx=0
Rút gọn :
\(A=\dfrac{sin\left(x+y\right)-sinx}{sin\left(x+y\right)+sinx}-\dfrac{cos\left(x+y\right)+cosx}{cos\left(x+y\right)-cosx}\)
Cho sinx - cosx = x. Tìm
A=sinx . cosx
B= sinx + cosx
C= sin3x - cos3x
a) nếu sin x = 3.cos x Tính sin x. cos x = .....?
b) cho sin x\(=\frac{2}{3}\). tính \(5cos^2x+2sin^2x=...?\)
c) sin x + cos x = \(\frac{5}{7}\). tính tan x = ...?
d) tan x = \(\frac{1}{2}\) tính \(\frac{sinx+cosx}{cosx-sinx}=...?\)
m.n giúp mk nha, ai nhanh mk tick cho nha
cho góc nhọn x biết
1. sinx=1/4 tính cosx. 2. tgx= 1/3, tính sinx.
mọi người giúp mình với ạ
1.
\(sin^2x+cos^2x=1\Rightarrow\left(\dfrac{1}{4}\right)^2+cos^2x=1\)
\(\Rightarrow cos^2x=\dfrac{15}{16}\Rightarrow cosx=\dfrac{\sqrt{15}}{4}\)
2.
\(tanx=\dfrac{1}{3}\Rightarrow tan^2x=\dfrac{1}{9}\Rightarrow\dfrac{sin^2x}{cos^2x}=\dfrac{1}{9}\)
\(\Rightarrow\dfrac{sin^2x}{1-sin^2x}=\dfrac{1}{9}\Rightarrow9sin^2x=1-sin^2x\)
\(\Rightarrow sin^2x=\dfrac{1}{10}\Rightarrow sinx=\dfrac{\sqrt{10}}{10}\)
Đúng 0
Bình luận (0)
Biết \(sinx+cosx=m\).
Tính giá trị biểu thức sau theo m: \(sin^3x+cos^3x\)
sin x+cosx=m
=>(sinx+cosx)^2=m^2
=>1+2*cosx*sinx=m^2
=>2*sinx*cosx=m^2-1
=>\(sinx\cdot cosx=\dfrac{m^2-1}{2}\)
\(sin^3x+cos^3x=\left(sinx+cosx\right)^3-3\cdot sinx\cdot cosx\cdot\left(sinx+cosx\right)\)\(=m^3-3\cdot\dfrac{m^2-1}{2}\cdot m\)
\(=m^3-\dfrac{3m^3-3m}{2}\)
\(=\dfrac{2m^3-3m^3+3m}{2}=\dfrac{-m^3+3m}{2}\)
Đúng 0
Bình luận (0)
Rut gon
A=\(\frac{sin\left(x+y\right)-sinx}{sin\left(x+y\right)+sinx}\) - \(\frac{cos\left(x+y\right)+cosx}{cos\left(x-y\right)-cosx}\)
Lời giải:
$A=\frac{2\cos \frac{2x+y}{2}\sin \frac{x}{2}}{2\sin \frac{2x+y}{2}.\cos \frac{x}{2}}-\frac{2\cos \frac{2x+y}{2}\cos \frac{x}{2}}{-2\sin \frac{2x+y}{2}\sin \frac{x}{2}}$
$=\tan \frac{x}{2}.\cot \frac{2x+y}{2}+\cot \frac{x}{2}.\cot \frac{2x+y}{2}=\cot \frac{2x+y}{2}(\tan \frac{x}{2}+\cot \frac{x}{2})$
Đúng 0
Bình luận (0)