\(sin\left(3x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{3}}{2}\)

\(\Leftrightarrow sin\left(3x-\dfrac{\pi}{4}\right)=sin\dfrac{\pi}{3}\)

\(\Leftrightarrow\left[{}\begin{matrix}3x-\dfrac{\pi}{4}=\dfrac{\pi}{3}+k2\pi\\3x-\dfrac{\pi}{4}=\pi-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7\pi}{36}+\dfrac{k2\pi}{3}\\x=\dfrac{11\pi}{36}+\dfrac{k2\pi}{3}\end{matrix}\right.\)

\(\Rightarrow\) Nghiệm âm lớn nhất: \(x_1=\dfrac{11\pi}{36}-\dfrac{2\pi}{3}=-\dfrac{13\pi}{36}\)

Nghiệm dương bé nhất: \(x_2=\dfrac{7\pi}{36}\)

\(\Rightarrow x_1+x_2=-\dfrac{\pi}{6}\)

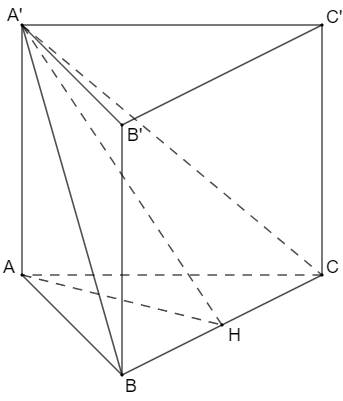
Góc giữa hai mặt phẳng (A'BC) và (ABC) bằng 60⁰

\(\Rightarrow\widehat{A'HA}=60^\circ\)

Tam giác AA'H vuông tại A: \(AH=AA'.cot60^\circ=\dfrac{2a\sqrt{3}}{3}\)

Tam giác ABC đều nên \(AH=\dfrac{BC\sqrt{3}}{2}\) \(\Rightarrow BC=\dfrac{4a}{3}\)

Thể tích khối lăng trụ là: \(V=\dfrac{1}{2}AH\cdot BC\cdot AA'=\dfrac{8\sqrt{3}}{9}a^3\).

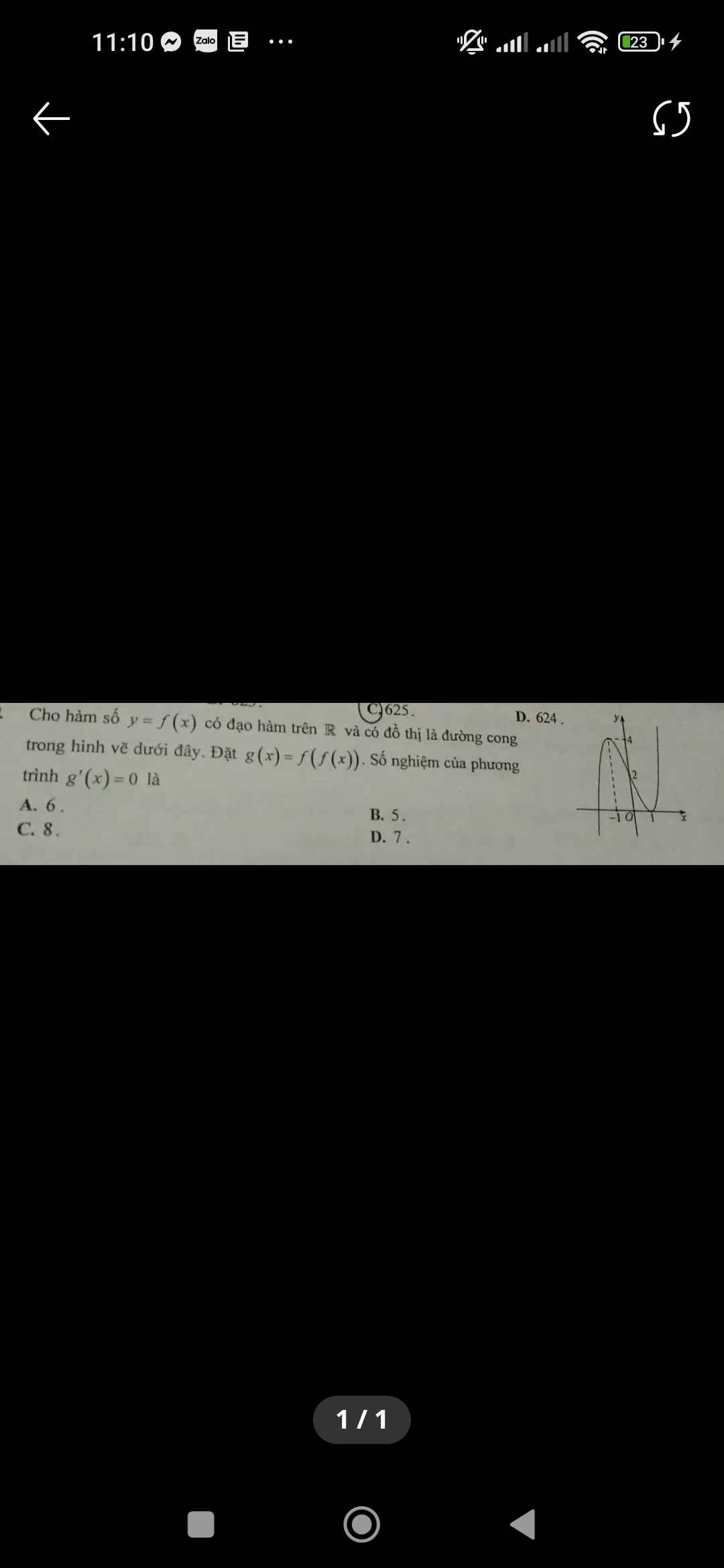
\(g'\left(x\right)=f'\left(x\right)f\left(f\left(x\right)\right)=0\Leftrightarrow\left[{}\begin{matrix}f'\left(x\right)=0\\f\left(f\left(x\right)\right)=0\end{matrix}\right.\)

\(f'\left(x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=1\end{matrix}\right.\)

\(f\left(f\left(x\right)\right)=0\Leftrightarrow\left[{}\begin{matrix}f\left(x\right)=x_1\left(x_1< -1\right)\\f\left(x\right)=1\end{matrix}\right.\)

\(f\left(x\right)=x_1\left(x_1< -1\right):\) phương trình có 1 nghiệm.

\(f\left(x\right)=1:\) phương trình có 3 nghiệm.

Số nghiệm của phương trình \(g'\left(x\right)=0\) là 6.

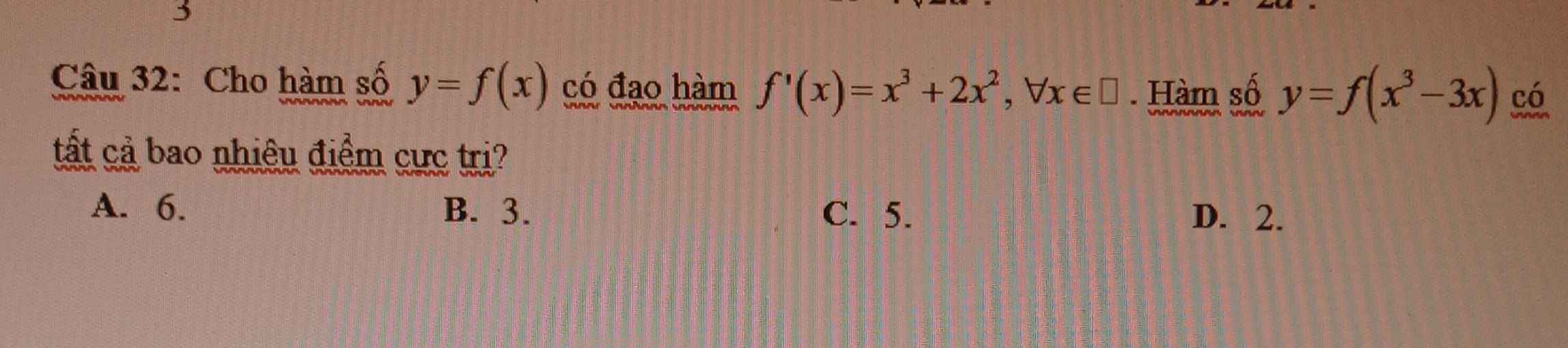
\(f'\left(x\right)=x^2\left(x+2\right)\) có đúng 1 nghiệm bội lẻ \(x=-2\)

\(y=f\left(x^3-3x\right)\Rightarrow y'=\left(3x^2-3\right).f'\left(x^3-3x\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}3x^2-3=0\\x^3-3x=-2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2-1=0\\\left(x-1\right)^2\left(x+2\right)=0\end{matrix}\right.\)

\(\Rightarrow x=\left\{-1;1;-2\right\}\) hàm có 3 điểm cực trị

f
′
(
x
)
=
x
2
(
x
+
2
)
 có đúng 1 nghiệm bội lẻ 
x
=
−
2

y
=
f
(
x
3
−
3
x
)
⇒
y
′
=
(
3
x
2
−
3
)
.
f
′
(
x
3
−
3
x
)
=
0

⇒
[
3
x
2
−
3
=
0
x
3
−
3
x
=
−
2
  
⇒
[
x
2
−
1
=
0
(
x
−
1
)
2
(
x
+
2
)
=
0
 

⇒
x
=
{
−
1
;
1
;
−
2
}
 hàm có 3 điểm cực trị

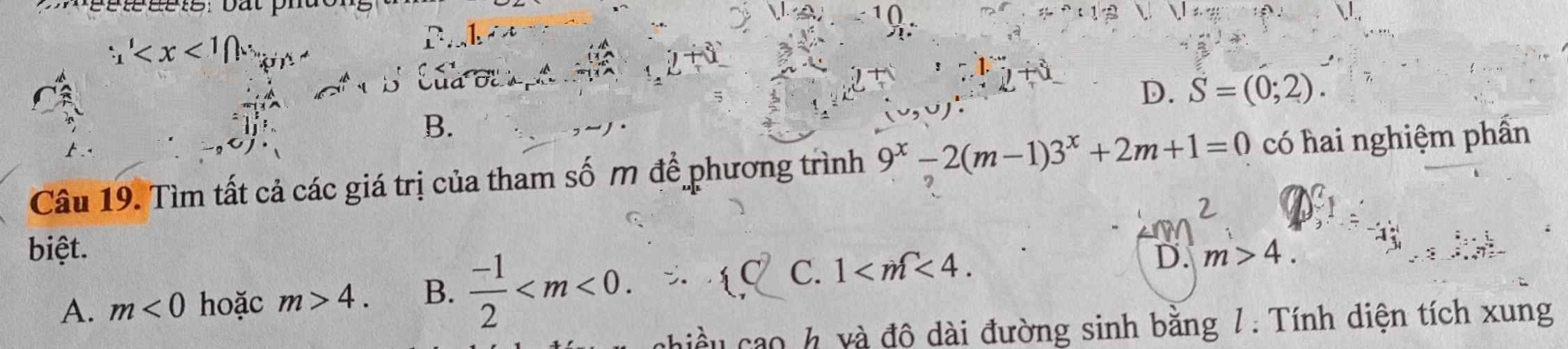
Đặt \(3^x=t,\left(t>0\right)\)

Phương trình đã cho trở thành: \(t^2-2\left(m-1\right)t+2m+1=0\) (2)

Yêu cầu bài toán tương đương với phương trình (2) có hai nghiệm dương phân biệt

\(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=\left(m-1\right)^2-2m-1>0\\t_1+t_2=2\left(m-1\right)>0\\t_1\cdot t_2=2m+1>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}m< 0\\m>4\end{matrix}\right.\\m>1\\m< -\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow m>4\)

Hàm số có tập xác định là \(ℝ\) \(\Leftrightarrow x^2-2x-m+1>0\forall x\inℝ\)

\(\Leftrightarrow\Delta'=1-\left(-m+1\right)< 0\)

\(\Leftrightarrow m< 0\)

\(m\in\left(-2021;2021\right)\), m nguyên nên có 2020 giá trị của m thỏa mãn.

\(y'=x^2+2mx+2m\)

Hàm đồng biến trên R khi và chỉ khi \(y'\ge0;\forall x\)

\(\Rightarrow\left\{{}\begin{matrix}a=1>0\\\Delta'=m^2-2m\le0\end{matrix}\right.\)

\(\Rightarrow0\le m\le2\)

Có 2 giá trị nguyên dương của m thỏa mãn (1 và 2)

Giả sử (P) cắt đáy nón theo đoạn thẳng AB

Độ dài AB là: \(2\left(1+\sqrt{5}\right)a-2.a\sqrt{5}=2a\)

Gọi H là trung điểm AB \(\Rightarrow OH\perp AB\)

\(AH=\dfrac{1}{2}AB=a\); \(SO=\sqrt{\left(a\sqrt{5}\right)^2-\left(2a\right)^2}=a\)

\(OH=\sqrt{R^2-AH^2}=a\sqrt{3}\)

Từ O kẻ \(OK\perp SH\Rightarrow OK=d\left(O;\left(P\right)\right)\)

Áp dụng hệ thức lượng trong tam giac vuông SOH:

\(\dfrac{1}{OK^2}=\dfrac{1}{SO^2}+\dfrac{1}{OH^2}=\dfrac{4}{3a^2}\Rightarrow OK=\dfrac{a\sqrt{3}}{2}\)