Question

Giải chi tiết giúp e vs

Answer 1

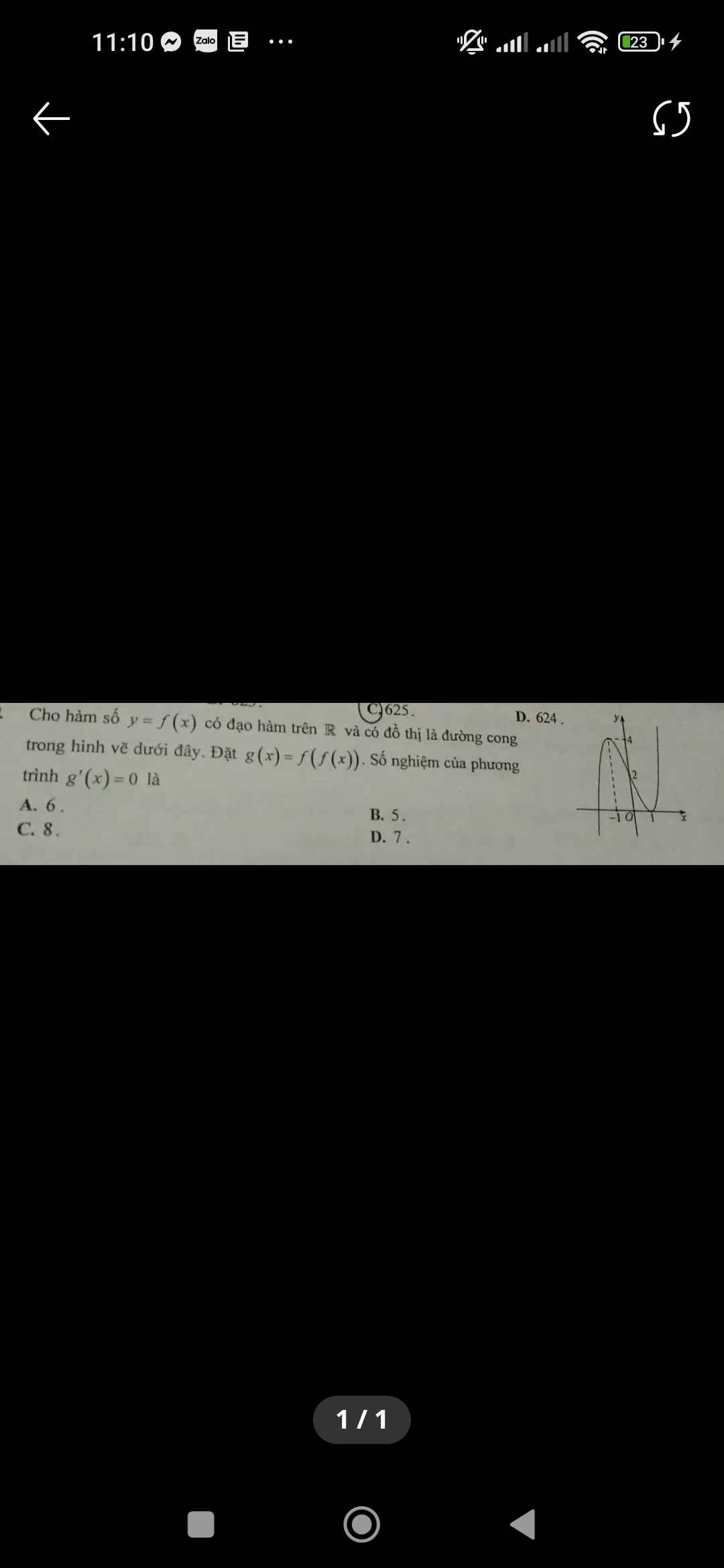
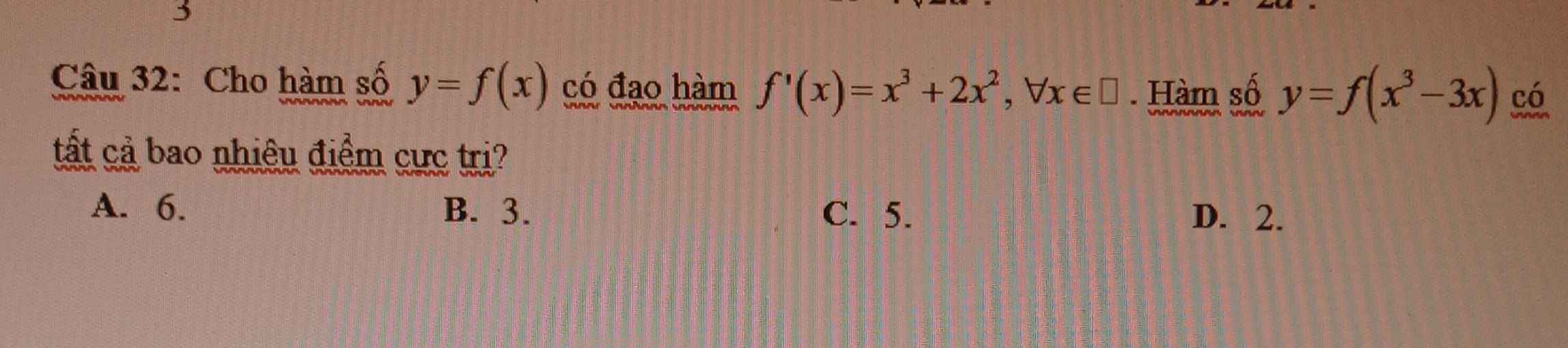\(f'\left(x\right)=x^2\left(x+2\right)\) có đúng 1 nghiệm bội lẻ \(x=-2\)

\(y=f\left(x^3-3x\right)\Rightarrow y'=\left(3x^2-3\right).f'\left(x^3-3x\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}3x^2-3=0\\x^3-3x=-2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2-1=0\\\left(x-1\right)^2\left(x+2\right)=0\end{matrix}\right.\)

\(\Rightarrow x=\left\{-1;1;-2\right\}\) hàm có 3 điểm cực trị

Answer 2

f
′
(
x
)
=
x
2
(
x
+
2
)
 có đúng 1 nghiệm bội lẻ 
x
=
−
2

y
=
f
(
x
3
−
3
x
)
⇒
y
′
=
(
3
x
2
−
3
)
.
f
′
(
x
3
−
3
x
)
=
0

⇒
[
3
x
2
−
3
=
0
x
3
−
3
x
=
−
2
  
⇒
[
x
2
−
1
=
0
(
x
−
1
)
2
(
x
+
2
)
=
0
 

⇒
x
=
{
−
1
;
1
;
−
2
}
 hàm có 3 điểm cực trị