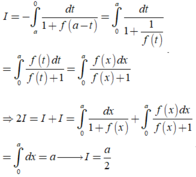Giả sử rằng f ( x - 2 ) sin 3 x d x = - ( x - 3 ) cos 3 x n + sin 3 x p + c . Tính giá trị của m+n+p
A. 14
B. -2
C. 9
D. 10
Cho \(f\left(x\right)=\frac{2}{3}x^3+\left(\cos a-3\sin a\right)x^2-8\left(1+\cos a\right)x+1\)
a) Chứng minh rằng hàm số luôn có cực đại và cực tiểu
b) Giả sử hàm số đạt cực trị tại \(x_1,x_2\). Chứng minh rằng \(x_1^2+x_2^2\le18\)
a) Xét phương trình : \(f'\left(x\right)=2x^2+2\left(\cos a-3\sin a\right)x-8\left(1+\cos2a\right)=0\)
Ta có : \(\Delta'=\left(\cos a-3\sin a\right)^2+16\left(1+\cos2a\right)=\left(\cos a-3\sin a\right)^2+32\cos^2\), \(a\ge0\) với mọi a
Nếu \(\Delta'=0\Leftrightarrow\cos a-3\sin a=\cos a=0\Leftrightarrow\sin a=\cos a\Rightarrow\sin^2a+\cos^2a=0\) (Vô lí)
Vậy \(\Delta'>0\)
với mọi a \(\Rightarrow f'\left(x\right)=0\)
có 2 nghiệm phân biệt \(x_1,x_2\) và hàm số có cực đại, cực tiểu
b) Theo Viet ta có \(x_1+x_2=3\sin a-\cos a\)
\(x_1x_2=-4\left(1+\cos2a\right)\)
\(x^2_1+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2=\left(3\sin a-\cos a\right)^2+8\left(1+\cos2a\right)=9+8\cos^2a-6\sin a\cos a\)
\(=9+9\left(\sin^2a+\cos^2a\right)-\left(3\sin a+\cos a\right)^2=18-\left(3\sin a+\cos2a\right)\le18\)
Cho hàm số \(f\left(x\right)=\left\{{}\begin{matrix}2\sin^2x+1,x< 0\\2^x;x\ge0\end{matrix}\right.\). Giả sử \(F\left(x\right)\) là một nguyên hàm của hàm số \(f\left(x\right)\) trên \(R\) và thỏa mãn điều kiện \(F\left(1\right)=\dfrac{2}{ln2}\). Tính \(F\left(-\pi\right)\)
A. \(F\left(-\pi\right)=-2\pi+\dfrac{1}{ln2}\) B. \(F\left(-\pi\right)=-2\pi-\dfrac{1}{ln2}\)
C. \(F\left(-\pi\right)=-\pi-\dfrac{1}{ln2}\) D. \(F\left(-\pi\right)=-2\pi\)
Mình cần bài giải ạ, mình cảm ơn nhiều ♥
Xem chi tiết
Chứng minh các đẳng thức sau(giả sử các biểu thức sau đều có nghĩa)
a) $\sin ^{4} x+\cos ^{4} x=1-2 \sin ^{2} x \cdot \cos ^{2} x$.
b) $\dfrac{1+\cot x}{1-\cot x}=\dfrac{\tan x+1}{\tan x-1}$.
c) $\dfrac{\cos x+\sin x}{\cos ^{3} x}=\tan ^{3} x+\tan ^{2} x+\tan x+1$.
\(a)sin^4x+cos^4x=1-2sin^2x\cdot cos^2x\)
\(\Leftrightarrow sin^4x+2sin^2x\cdot cos^2x+cos^4x=1\)
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^2=1\)(luôn đúng)
a)
b)
c)
a) VT=(sin2x + cos 2 x)2 - 2sin2 x . cos2 x = VP
b) VT= \(\dfrac{1+\dfrac{1}{tanx}}{1-\dfrac{1}{tanx}}\)=VP
c) VT= \(\dfrac{1}{cos^2x}+\dfrac{sinx}{cosx}.\dfrac{1}{cos^2x}=1+tan^2x+tanx.\left(1+tan^2x\right)=VP\)
Chứng minh rằng \(f'\left(x\right)=0;\forall x\in R\) nếu :
a) \(f\left(x\right)=3\left(\sin^4x+\cos^4x\right)-2\left(\sin^6x+\cos^6x\right)\)
b) \(f\left(x\right)=\cos^6x+2\sin^4x.\cos^2x+3\sin^2x\cos^4x+\sin^4x\)
c) \(f\left(x\right)=\cos\left(x-\dfrac{\pi}{3}\right)\cos\left(x+\dfrac{\pi}{4}\right)+\cos\left(x+\dfrac{\pi}{6}\right)\cos\left(x+\dfrac{3\pi}{4}\right)\)
d) \(f\left(x\right)=\cos^2x+\cos^2\left(\dfrac{2\pi}{3}+x\right)+\cos^2\left(\dfrac{2\pi}{3}-x\right)\)
Chứng minh các biểu thức đã cho không phụ thuộc vào x.
Từ đó suy ra f'(x)=0
a) f(x)=1⇒f′(x)=0f(x)=1⇒f′(x)=0 ;
b) f(x)=1⇒f′(x)=0f(x)=1⇒f′(x)=0 ;
c) f(x)=\(\frac{1}{4}\)(\(\sqrt{2}\)-\(\sqrt{6}\))=>f'(x)=0
d,f(x)=\(\frac{3}{2}\)=>f'(x)=0
1. Cho f(x) là đa thức bậc 2 và a, b, c là 3 số thực phân biệt thỏa mãn f(a)=bc, f(b)=ca, f(c)=ab. Chứng minh rằng f(a+b+c)=ab+bc+ca.
2. Giả sử a, b, c, d là 2 trong 4 nghiệm của P(x)=\(x^4+x^3-1\), chứng minh rằng ab là nghiệm của \(x^6+x^4+x^3-x^2-1\)
Em xin cảm ơn!
Bài tập 3: Cho hàm số
f( x )=c o s x. Chứng minh rằng:
2f'(x+pi/3).f'(x-pi/6)=f'(0)-f(2x+pi/6)
Bài tập 4: Cho hàm số y=3(sin^4 x +cos^4 )-2(sin^6 x +cos^6 x). Chứng minh rằng: y'=0 \-/ x€ Z
Bài tập 5: Cho hàm số
Y= (sin x/ 1+cos x)^3. CMR: y'.sinx-3y=0
3.
\(f\left(x+\frac{\pi}{3}\right)=cos\left(x+\frac{\pi}{3}\right)\Rightarrow f'\left(x+\frac{\pi}{3}\right)=-sin\left(x+\frac{\pi}{3}\right)\)
\(f'\left(x-\frac{\pi}{6}\right)=-sin\left(x-\frac{\pi}{6}\right)\)
\(f'\left(0\right)=-sin\left(0\right)=0\)
\(2f'\left(x+\frac{\pi}{3}\right).f'\left(x-\frac{\pi}{6}\right)=2sin\left(x+\frac{\pi}{3}\right)sin\left(x-\frac{\pi}{6}\right)\)
\(=cos\left(\frac{\pi}{2}\right)-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)=0-cos\left(2x+\frac{\pi}{6}\right)=-cos\left(2x+\frac{\pi}{6}\right)\)
\(\Rightarrow2f'\left(x+\frac{\pi}{3}\right)f'\left(x-\frac{\pi}{6}\right)=f'\left(0\right)-f\left(2x+\frac{\pi}{6}\right)\) (đpcm)
4.
\(y=3\left(sin^4x+cos^4x\right)-2\left(sin^6x+cos^6x\right)\)
\(=3\left(sin^2x+cos^2x\right)^2-6sin^2x.cos^2x-2\left(sin^2x+cos^2x\right)^3+6sin^2x.cos^2x\left(sin^2x+cos^2x\right)\)
\(=3-2=1\)
\(\Rightarrow y'=0\) ; \(\forall x\)
5.
\(y=\left(\frac{sinx}{1+cosx}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{1-cos^2x}\right)^3=\left(\frac{sinx\left(1-cosx\right)}{sin^2x}\right)^3=\left(\frac{1-cosx}{sinx}\right)^3\)
\(y'=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{sin^2x-cosx\left(1-cosx\right)}{sin^2x}\right)=3\left(\frac{1-cosx}{sinx}\right)^2\left(\frac{1-cosx}{sin^2x}\right)=\frac{3\left(1-cosx\right)^3}{sin^4x}\)
\(\Rightarrow y'.sinx-3y=\frac{3\left(1-cosx\right)^3}{sin^3x}-3\left(\frac{1-cosx}{sinx}\right)^3=0\) (đpcm)
Bài 1: Cho đa thức bậc nhất: f(x) = ax + b và g(x) = bx + a (a và b khác 0). Giả sử đa thức f(x) có nghiệm là x0, tìm nghiệm của đa thức g(x)
Bài 2: Chứng tỏ rằng f(x) = -8x4 + 6x3 - 4x2 + 2x - 1 không có nghiệm nguyên.
Bài 3: Cho đa thức f(x) = ax3 + bx2 + cx + d có giá trị nguyên với mọi x thuộc Z. Chứng tỏ rằng 6a và 2b là các số nguyên
Giả sử hàm số \(f\left(x\right)\) liên tục trên đoạn \(\left[a;b\right]\). Chứng minh rằng :
\(\int\limits^{\dfrac{\pi}{2}}_0f\left(\sin x\right)dx=\int\limits^{\dfrac{\pi}{2}}_0f\left(\cos x\right)dx\)
Cho hàm số y = f(x) liên tục trên R và a > 0. Giả sử rằng với mọi x ∈ 0 ; a , ta có f(x) > 0 và f(x)f(a – x) = 1. Tính I = ∫ 0 a d x 1 + f ( x ) .
A. a 2 .
B. 2a.
C. a 3 .
D. aln(a + 1).
Chọn A.
Từ giả thiết, suy ra f a - x = 1 f x
Đặt t=a-x suy ra dt=-dx . Đổi cận: x = 0 → t = a x = a → t = 0
Khi đó