Biết ∫ 1 2 ln x x 2 d x = a ln 2 + b c (với a là số hữu tỉ, b, c là các số nguyên dương và b c là phân số tối giản). Tính giá trị của S = 2 a + 3 b + c
![]()
![]()
![]()
![]()
4. Tính đạo hàm của các hàm số sau:
a) \(y = (3x^2-4x+1)^{-4}\)
b) \(y = 3^{x^2-1} + e^{-x+1}\)
c) \(y = \ln (x^2-4x) + \log_{3} (2x-1)\)
d) \(y =x . \ln x + 2^{\frac{x-1}{x+1}}\)
e) \(y = x^{-7} - \ln (x^2-1)\)
`a)TXĐ:R\\{1;1/3}`
`y'=[-4(6x-4)]/[(3x^2-4x+1)^5]`
`b)TXĐ:R`
`y'=2x. 3^[x^2-1] ln 3-e^[-x+1]`
`c)TXĐ: (4;+oo)`
`y'=[2x-4]/[x^2-4x]+2/[(2x-1).ln 3]`
`d)TXĐ:(0;+oo)`
`y'=ln x+2/[(x+1)^2].2^[[x-1]/[x+1]].ln 2`
`e)TXĐ:(-oo;-1)uu(1;+oo)`
`y'=-7x^[-8]-[2x]/[x^2-1]`
Lời giải:
a.
$y'=-4(3x^2-4x+1)^{-5}(3x^2-4x+1)'$
$=-4(3x^2-4x+1)^{-5}(6x-4)$
$=-8(3x-2)(3x^2-4x+1)^{-5}$
b.
$y'=(3^{x^2-1})'+(e^{-x+1})'$
$=(x^2-1)'3^{x^2-1}\ln 3 + (-x+1)'e^{-x+1}$
$=2x.3^{x^2-1}.\ln 3 -e^{-x+1}$
c.
$y'=\frac{(x^2-4x)'}{x^2-4x}+\frac{(2x-1)'}{(2x-1)\ln 3}$
$=\frac{2x-4}{x^2-4x}+\frac{2}{(2x-1)\ln 3}$
d.
\(y'=(x\ln x)'+(2^{\frac{x-1}{x+1}})'=x(\ln x)'+x'\ln x+(\frac{x-1}{x+1})'.2^{\frac{x-1}{x+1}}\ln 2\)
\(=x.\frac{1}{x}+\ln x+\frac{2}{(x+1)^2}.2^{\frac{x-1}{x+1}}\ln 2\\ =1+\ln x+\frac{2^{\frac{2x}{x+1}}\ln 2}{(x+1)^2}\)
e.
\(y'=-7x^{-8}-\frac{(x^2-1)'}{x^2-1}=-7x^{-8}-\frac{2x}{x^2-1}\)
Tính đạo hàm cấp hai của mỗi hàm số sau:
a) \(y = 2{x^4} - 3{x^3} + 5{x^2}\)
b) \(y = \frac{2}{{3 - x}}\)
c) \(y = \sin 2x\cos x\)
d) \(y = {e^{ - 2x + 3}}\)
e) \(y = \ln (x + 1)\)
f) \(y = \ln ({e^x} + 1)\)
\(a,y'=8x^3-9x^2+10x\\ \Rightarrow y''=24x^2-18x+10\\ b,y'=\dfrac{2}{\left(3-x\right)^2}\\ \Rightarrow y''=\dfrac{4}{\left(3-x\right)^3}\)
\(c,y'=2cos2xcosx-sin2xsinx\\ \Rightarrow y''=-5sin\left(2x\right)cos\left(x\right)-4cos\left(2x\right)sin\left(x\right)\\ d,y'=-2e^{-2x+3}\\ \Rightarrow y''=4e^{-2x+3}\)
e,
\(y = \ln (x + 1) \Rightarrow y' = \frac{1}{{x + 1}} \Rightarrow y'' = - \frac{1}{{{{\left( {x + 1} \right)}^2}}}\)
f,
\(y = \ln ({e^x} + 1) \Rightarrow y' = \frac{{{e^x}}}{{{e^x} + 1}} \Rightarrow y'' = - \frac{{{e^x}.{e^x}}}{{{{\left( {{e^x} + 1} \right)}^2}}} = - \frac{{{e^{2x}}}}{{{{\left( {{e^x} + 1} \right)}^2}}}\)
Giải các phương trình sau :
a) \(\ln\left(4x+2\right)-\ln\left(x-1\right)=\ln x\)
b) \(\log_2\left(3x+1\right)\log_3x=2\log_2\left(3x+1\right)\)
c) \(2^{\log_3x^2}.5^{\log_3x}=400\)
d) \(\ln^3x-3\ln^2x-4\ln x+12=0\)
a) Điều kiện: \(\left\{{}\begin{matrix}4x+2>0\\x-1>0\\x>0\end{matrix}\right.\)
Hay là: \(x>1\)
Khi đó biến đổi pương trình như sau:
\(\ln\dfrac{4x+2}{x-1}=\ln x\)
\(\Leftrightarrow\dfrac{4x+2}{x-1}=x\)
\(\Leftrightarrow4x+2=x\left(x-1\right)\)
\(\Leftrightarrow x^2-5x-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{5+\sqrt{33}}{2}\\x_2=\dfrac{5-\sqrt{33}}{2}\left(loại\right)\end{matrix}\right.\)
Vậy nghiệm của phương trình là: \(x=\dfrac{5+\sqrt{33}}{2}\)
b) Điều kiện: \(\left\{{}\begin{matrix}3x+1>0\\x>0\end{matrix}\right.\)
Hay là: \(x>0\)
Biến đổi phương trình như sau:
\(\log_2\left(3x+1\right)\log_3x-2\log_2\left(3x+1\right)=0\)
\(\Leftrightarrow\log_2\left(3x+1\right)\left(\log_3x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\log_2\left(3x+1\right)=0\\\log_3x=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x+1=2^0\\x=3^2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(loại\right)\\x=9\end{matrix}\right.\)
Vậy nghiệm là x = 9.
c) Điều kiện: x > 0.
Khi đó biến đổi phương trình như sau:
\(2^{\log_3x^2}.5^{\log_3x}=400\)
\(\Leftrightarrow2^{2\log_3x}.5^{\log_3x}=400\)
\(\Leftrightarrow\left(2^2.5\right)^{\log_3x}=400\)
\(\Leftrightarrow20^{\log_3x}=20^2\)
\(\Leftrightarrow\log_3x=2\)
\(\Leftrightarrow x=3^2=9\) (thỏa mãn)
Giải các bất phương trình sau:
a) (2x − 7)ln(x + 1) > 0;
b) (x − 5)(logx + 1) < 0;
c) 2 log 3 2 x + 5 log 2 2 x + log 2 x – 2 ≥ 0
d) ln(3 e x − 2) ≤ 2x
a) Bất phương trình đã cho tương đương với hệ sau:
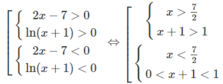
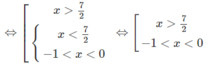
Vậy tập nghiệm là (−1;0) ∪ (7/2; + ∞ )
b) Tương tự câu a), tập nghiệm là (1/10; 5)
c) Đặt t = log 2 x , ta có bất phương trình 2 t 3 + 5 t 2 + t – 2 ≥ 0 hay (t + 2)(2 t 2 + t − 1) ≥ 0 có nghiệm −2 ≤ t ≤ −1 hoặc t ≥ 1/2
Suy ra 1/4 ≤ x ≤ 1/2 hoặc x ≥ 2
Vậy tập nghiệm của bất phương trình đã cho là: [1/4; 1/2] ∪ [ 2 ; + ∞ )
d) Bất phương trình đã cho tương đương với hệ:
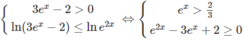
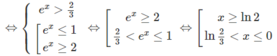
Vậy tập nghiệm là (ln(2/3); 0] ∪ [ln2; + ∞ )
Giải các bất phương trình lôgarit sau :
a) \(\dfrac{\ln x+2}{\ln x-1}< 0\)
b) \(\log^2_{0,2}x-\log_{0,2}x-6\le0\)
c) \(\log\left(x^2-x-2\right)< 2\log\left(3-x\right)\)
d) \(\ln\left|x-2\right|+\ln\left|x+4\right|\le3\ln2\)
Giải các phương trình sau:
a) \(\log \left( {x + 1} \right) = 2;\)
b) \(2{\log _4}x + {\log _2}\left( {x - 3} \right) = 2;\)
c) \(\ln x + \ln \left( {x - 1} \right) = \ln 4x;\)
d) \({\log _3}\left( {{x^2} - 3x + 2} \right) = {\log _3}\left( {2x - 4} \right).\)
a, ĐK: \(x+1>0\Leftrightarrow x>-1\)
\(log\left(x+1\right)=2\\ \Leftrightarrow x+1=10^2\\ \Leftrightarrow x+1=100\\ \Leftrightarrow x=99\left(tm\right)\)
b, ĐK: \(\left\{{}\begin{matrix}x-3>0\\x>0\end{matrix}\right.\Rightarrow x>3\)
\(2log_4x+log_2\left(x-3\right)=2\\ \Leftrightarrow log_2x+log_2\left(x-3\right)=2\\ \Leftrightarrow log_2\left(x^2-3x\right)=2\\ \Leftrightarrow x^2-3x=4\\ \Leftrightarrow x^2-3x-4=0\\ \Leftrightarrow\left(x+1\right)\left(x-4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=-1\left(ktm\right)\\x=4\left(tm\right)\end{matrix}\right.\)
c, ĐK: \(x>1\)
\(lnx+ln\left(x-1\right)=ln4x\\ \Leftrightarrow ln\left[x\left(x-1\right)\right]-ln4x=0\\ \Leftrightarrow ln\left(\dfrac{x-1}{4}\right)=0\\ \Leftrightarrow\dfrac{x-1}{4}=1\\ \Leftrightarrow x-1=4\\ \Leftrightarrow x=5\left(tm\right)\)
d, ĐK: \(\left\{{}\begin{matrix}x^2-3x+2>0\\2x-4>0\end{matrix}\right.\Rightarrow x>2\)
\(log_3\left(x^2-3x+2\right)=log_3\left(2x-4\right)\\ \Leftrightarrow x^2-3x+2=2x-4\\ \Leftrightarrow x^2-5x+6=0\\ \Leftrightarrow\left(x-2\right)\left(x-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\left(ktm\right)\\x=3\left(tm\right)\end{matrix}\right.\)
Tìm đạo hàm của các hàm số sau:
1, \(y=3^{(\dfrac{x}{\ln(x)})}\)
2, \(y=\dfrac{1}{2}tan^2(x)+\ln(tan(x))\)
3, \(y=\sqrt[3]{ln^2(2x)}\)
1.
\(y'=\left(\dfrac{x}{lnx}\right)'.3^{\dfrac{x}{lnx}}.ln3=\dfrac{lnx-1}{ln^2x}.3^{\dfrac{x}{lnx}}.ln3\)
2.
\(y'=\left(tanx\right)'.tanx+\left(tanx\right)'.\dfrac{1}{tanx}=\dfrac{tanx}{cos^2x}+\dfrac{1}{tanx.cos^2x}\)
3.
\(y=\left(ln2x\right)^{\dfrac{2}{3}}\Rightarrow y'=\left(ln2x\right)'.\dfrac{2}{3}.\left(ln2x\right)^{-\dfrac{1}{3}}=\dfrac{1}{3x\sqrt[3]{ln2x}}\)
Giải các phương trình logarit sau :
a) \(\frac{1}{4+\log_3x}+\frac{1}{2-\log_3x}=1\)
b) \(-\ln^3x+2\ln x=2-\ln x\)
c)\(x^{lg^2x^2-3lgx-\frac{9}{2}}=10^{-2lgx}\)
d) \(\log_2\sqrt{\left|x\right|}-4\sqrt{\log_4\left|x\right|}-5=0\)
d) Điều kiện \(\begin{cases}x\ne0\\\log_2\left|x\right|\ge0\end{cases}\)\(\Leftrightarrow\left|x\right|\ge\)1
Phương trình đã cho tương đương với :
\(\log_2\left|x\right|^{\frac{1}{2}}-4\sqrt{\log_{2^2}\left|x\right|}-5=0\)
\(\Leftrightarrow\frac{1}{2}\log_2\left|x\right|-4\sqrt{\frac{1}{4}\log_2\left|x\right|}-5=0\)
Đặt \(t=\sqrt{\frac{1}{2}\log_2\left|x\right|}\) \(\left(t\ge0\right)\) thì phương trình trở thành :
\(t^2-4t-5=0\) hay t=-1 V t=5
Do \(t\ge0\) nên t=5
\(\Rightarrow\frac{1}{2}\log_2\left|x\right|=25\Leftrightarrow\log_2\left|x\right|=50\Leftrightarrow\left|x\right|=2^{50}\) Thỏa mãn
Vậy \(x=\pm2^{50}\) là nghiệm của phương trình
c) Điều kiện x>0. Phương trình đã cho tương đương với :
\(x^{lg^2x^2-3lgx-\frac{9}{2}}=\left(10^{lgx}\right)^{-2}\)
\(\Leftrightarrow lg^2x^2-3lgx-\frac{9}{2}=-2\)
\(\Leftrightarrow8lg^2x-6lgx-5=0\)
Đặt \(t=lgx\left(t\in R\right)\) thì phương trình trở thành
\(8t^2-6t-5=0\) hay\(t=-\frac{1}{2}\) V \(t=\frac{5}{4}\)
Với \(t=-\frac{1}{2}\) thì \(lgx=-\frac{1}{2}\Leftrightarrow x=\frac{1}{\sqrt{10}}\)
Với \(t=\frac{5}{4}\) thì \(lgx=\frac{5}{4}\Leftrightarrow x=\sqrt[4]{10^5}\)
Vậy phương trình đã cho có nghiệm \(x=\sqrt[4]{10^5}\) và \(x=\frac{1}{\sqrt{10}}\)
b) Điều kiện x>0, đặt \(t=lgx\left(t\in R\right)\) , phương trình trở thành
\(t^3-2t^2-t+2=0\Leftrightarrow\left(t-1\right)\left(t+1\right)\left(t-2\right)=0\)
Do đó, t nhận các giá trị : 1, -1 hoặc 2
Với t = 1 thì \(lgx=1\Leftrightarrow x=10^1=10\)
Với t = - thì \(lgx=-1\Leftrightarrow x=10^{-1}=\frac{1}{10}\)
Với t = 2 thì \(lgx=2\Leftrightarrow x=10^2=100\)Biết \(\int_1^e\frac{1-\ln x}{\left(x+\ln x\right)^2}dx=\frac{1}{ae+b}\) với a, b\(\in\) Z. Tính \(a^2+b^2\)
\(I=\int\limits^e_1\frac{\frac{1-lnx}{x^2}}{\left(1+\frac{lnx}{x}\right)^2}dx\)
Đặt \(\frac{lnx}{x}=t\Rightarrow\left(\frac{1-lnx}{x^2}\right)dx=dt\)
\(\Rightarrow I=\int\limits^{\frac{1}{e}}_0\frac{dt}{\left(1+t\right)^2}=-\frac{1}{1+t}|^{\frac{1}{e}}_0=\frac{1}{e+1}\)
\(\Rightarrow a=b=1\Rightarrow a^2+b^2=2\)
Tính các tích phân sau: 1) 2 ln e e x dx ; 2) 1 3 2 0 4 x dx x ; 3) /2 /4 1 tan dx x ; 4) 1 0 x e dx ; 5) 2 1 x xe dx ; 6) 0 1 3 4 dx x ; 7) 2 1 4 4 5 dx x x ; 8) 2 0 ln 1 x dx x (HD: 1 u x ) ĐS: 1) 2 e ; 2) 16 7 5 3 ; 3) ln 2 ; 4) 2