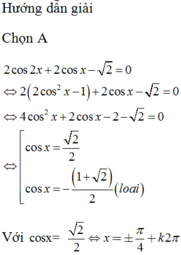2cos4x + 2cos2x - √2 = 0
TT
Những câu hỏi liên quan
2sin5x + 1/sinx = 2cos4x + 2cos2x + 3
\(\Leftrightarrow2sin5x.sinx+1=2cos4x.sinx+2cos2x.sinx+3sinx\)
\(\Leftrightarrow2sin5x.sinx+1=sin5x-sin3x+sin3x-sinx+3sinx\)
\(\Leftrightarrow2sin5x.sinx-sin5x-2sinx+1=0\)
\(\Leftrightarrow sin5x\left(2sinx-1\right)-\left(2sinx-1\right)=0\)
Chứng minh: \(sin9x=sinx\left(1+2cos2x+2cos4x+2cos6x+2cos8x\right)\)
CMR :
sinx (1+ 2cos2x + 2cos4x + 2cos6x ) = sin7x
\(sinx\left(1+2cos2x+2cos4x+2cos6x\right)\)
\(=sinx+2sinx.cos2x+2sinx.cos4x+2sinx.cos6x\)
\(=sinx+sin3x+sin\left(-x\right)+sin5x+sin\left(-3x\right)+sin7x+sin\left(-5x\right)\)
\(=sinx+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)
\(=sin7x\)
Đúng 2
Bình luận (0)
Rút gọn
A= \(\frac{cosx-cos2x-cos3x+cos4x}{sinx-sin2x-sin3x+sin4x}\)
B= sinx(1+2cos2x+2cos4x+2cos6x)
\(A=\frac{cosx-cos3x+cos4x-cos2x}{sinx-sin3x+sin4x-sin2x}=\frac{2sin2x.sinx-2sin3x.sinx}{-2cos2x.sinx+2cos3x.sinx}\)
\(=\frac{sin2x-sin3x}{cos3x-cos2x}=\frac{-2cos\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}{-2sin\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}=cot\left(\frac{5x}{2}\right)\)
\(B=sinx+2cos2x.sinx+2cos4x.sinx+2cos6x.sinx\)
\(=sinx+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)
\(=sin7x\)
Đúng 0
Bình luận (0)
2cos4x+4sin2xcos2x-\(\sqrt{2}\)=0
\(2\cos4x+4\sin2x.\cos2x-\sqrt{2}=0\\ < =>2.\cos4x+2.\sin4x=\sqrt{2}\\ < =>2\sqrt{2}\sin\left(x+\dfrac{\pi}{4}\right)=\sqrt{2}\\ < =>\sin\left(4x+\dfrac{\pi}{4}\right)=\dfrac{1}{2}\\ < =>\left[{}\dfrac{x+\dfrac{\pi}{4}=\dfrac{\pi}{6}+k2\pi}{x+\dfrac{\pi}{4}=\dfrac{5\pi}{6}+k2\pi}}\\ < =>\left[{}\begin{matrix}x=\dfrac{-\pi}{12}+k2\pi\\x=\dfrac{7\pi}{12}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (4)
4x^2 +4xsin2x -2cos2x+2=0
Nghiệm của phương trình 2cos2x + 2cosx
-
2
0
Đọc tiếp
Nghiệm của phương trình 2cos2x + 2cosx - 2 = 0
![]()
![]()
Giải phương trình :
Cotx=tanx +2cos4x\sin2x
Và
sinx +cos2x - 2cosx×cos3x=0
câu 1
⇒ \(\dfrac{cosx}{sinx}\) - \(\dfrac{sinx}{cosx}\) -\(\dfrac{2cos4x}{2sinxcosx}\) =0
⇔ \(\dfrac{cos^2x-sin^2x}{sinx.cosx}\) -\(\dfrac{cos4x}{sinx.cosx}\)= 0
⇔ \(\dfrac{cos2x-cos4x}{sinx.cosx}\) = 0
\(\left[{}\begin{matrix}cos2x=cos4x\\sin2x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=4x+k2\pi\\2x=-4x+k2\pi\\2x=k\pi\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-k\pi\\x=\dfrac{k\pi}{3}\\x=\dfrac{k\pi}{2}\end{matrix}\right.\) (k∈ Z)
Đúng 0
Bình luận (0)
câu 2 dùng công thức biến đổi tích thành tổng thành cos 4x + cos 2x sau đó phương trình trở thành sin x - cos 4x=0
Đúng 0
Bình luận (0)
1. 2cos2x + sinx = sin3x
2. cos2x + 2(sin3x-1)sin2(π/4 - x/2) = 0
1.
\(\Leftrightarrow2cos2x+sinx-sin3x=0\)
\(\Leftrightarrow2cos2x-2cos2x.sinx=0\)
\(\Leftrightarrow2cos2x\left(1-sinx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\sinx=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{2}+k2\pi\\x=\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+k\pi\\x=\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
2.
\(cos^2x+\left(sin3x-1\right)\left(1-cos\left(\dfrac{\pi}{2}-x\right)\right)=0\)
\(\Leftrightarrow1-sin^2x+\left(sin3x-1\right)\left(1-sinx\right)=0\)
\(\Leftrightarrow\left(1-sinx\right)\left(1+sinx\right)+\left(sin3x-1\right)\left(1-sinx\right)=0\)
\(\Leftrightarrow\left(1-sinx\right)\left(1+sinx+sin3x-1\right)=0\)
\(\Leftrightarrow2\left(1-sinx\right)sin2x.cosx=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=1\\sin2x=0\\cosx=0\end{matrix}\right.\)
\(\Leftrightarrow sin2x=0\)
\(\Leftrightarrow x=\dfrac{k\pi}{2}\)
Đúng 0
Bình luận (1)