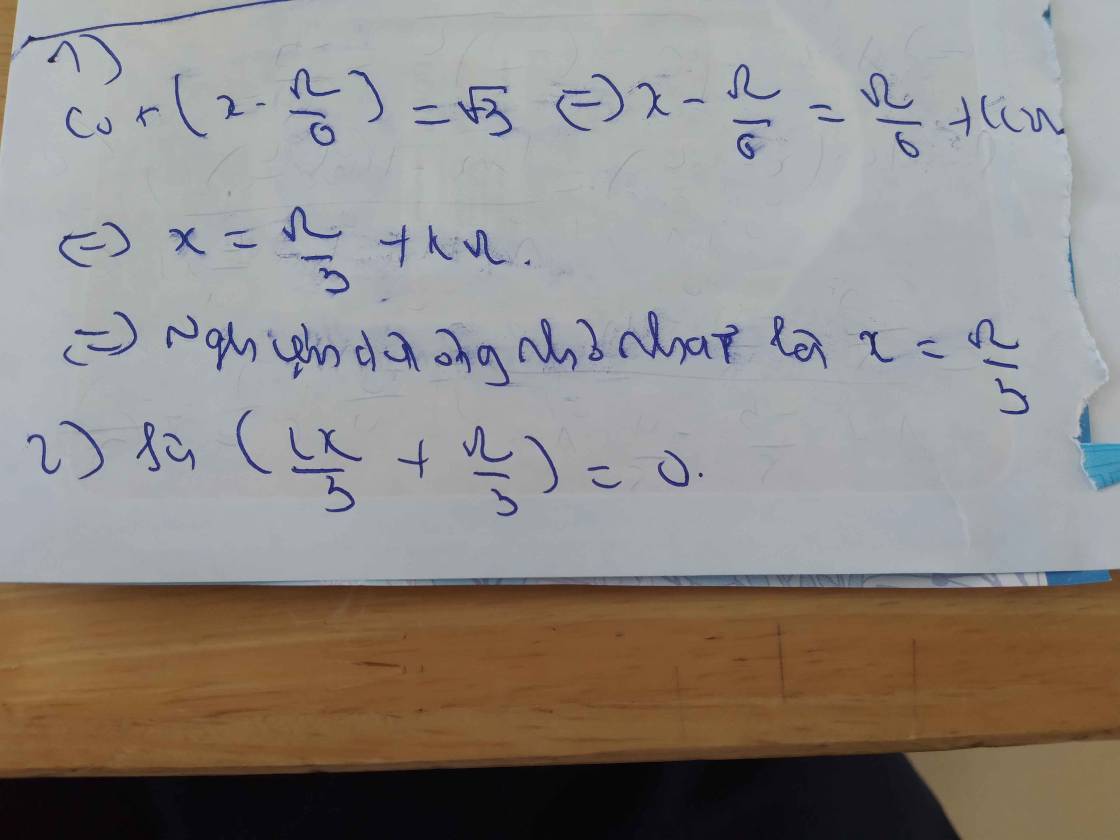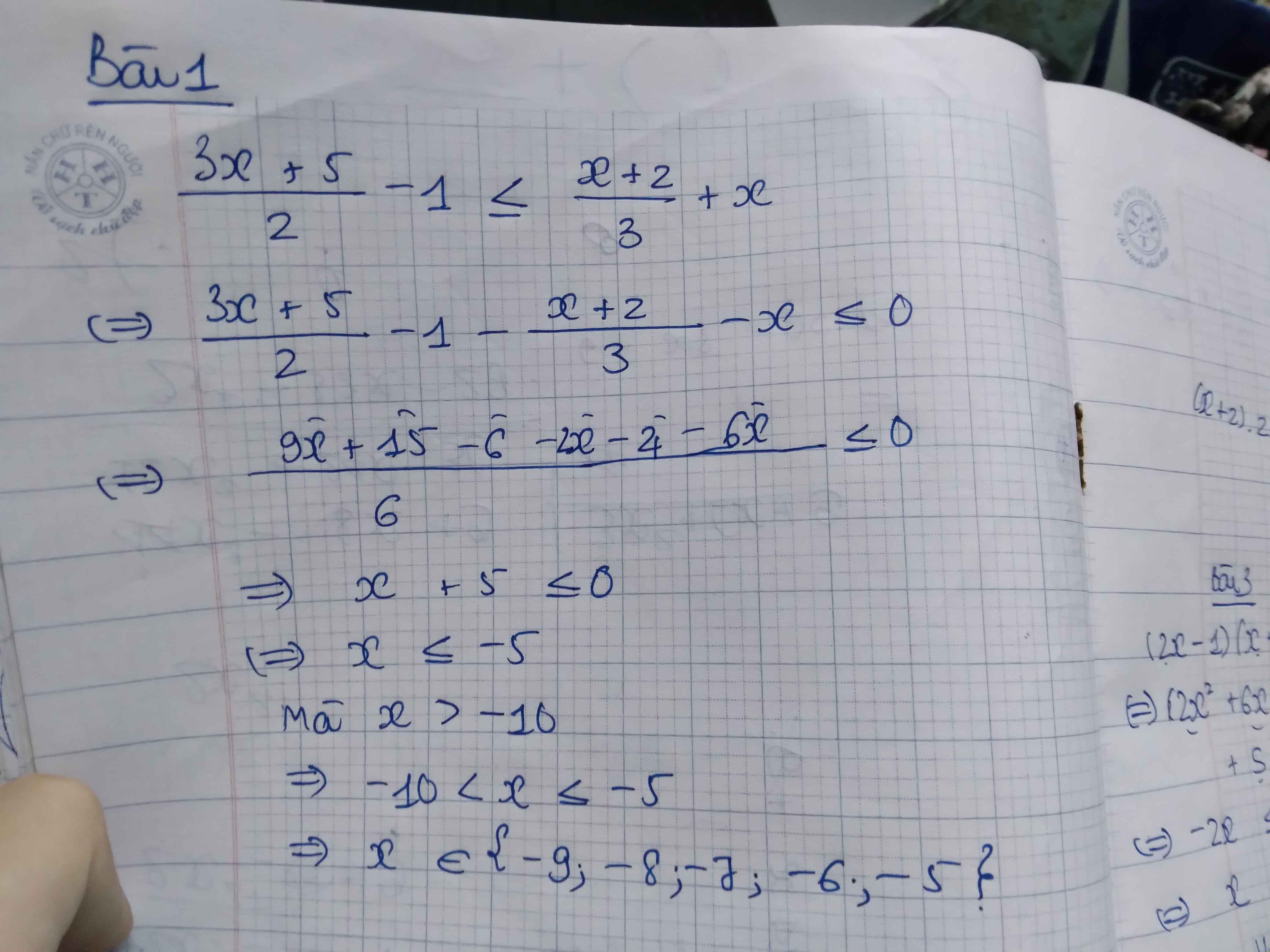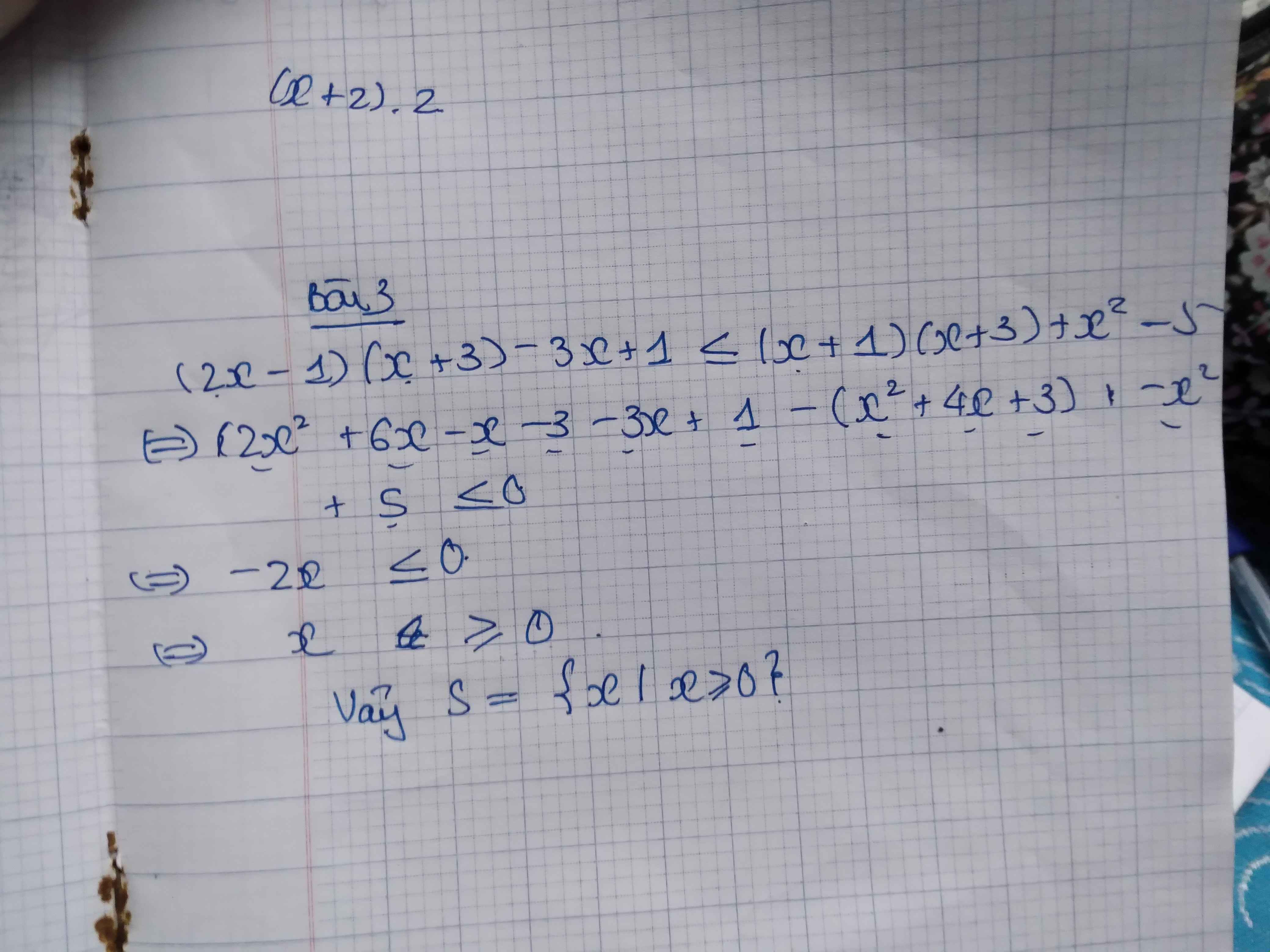(\(\dfrac{1}{2}\)) -x – 2.4x – 3(\(\sqrt{2}\) )2x =0 có nghiệm là
KT
Những câu hỏi liên quan
1) nghiệm dương nhỏ nhất của phương trình \(cot\left(x-\dfrac{\pi}{6}\right)=\sqrt{3}\) là
2) phương trình \(sin\left(\dfrac{2x}{3}+\dfrac{\pi}{3}\right)=0\) có nghiệm là
3) họ nghiệm của phương trình \(cot\)(2x - 30 độ) = \(\sqrt{3}\) là
Trắc nghiệm Câu 1: Biết cos adfrac{2}{3} thì sin a có giá trị là : A. dfrac{1}{3} B.dfrac{sqrt{5}}{3} C dfrac{5}{9} D.dfrac{5}{3}Câu 2 : sqrt{dfrac{2}{x}} có nghĩa khi và chỉ khi là : A. x ≥ 0 B. x 0 C. x ≠ 0 D. x ≠ 2Câu 3 : Δ ABC vuông tại A có góc B 300 , BC 24cm . Độ dài AC bằng : A. 9 B. 6sqrt{3} C. sqrt{18} ...
Đọc tiếp
Trắc nghiệm
Câu 1: Biết \(\cos a=\dfrac{2}{3}\) thì \(\sin a\) có giá trị là : A. \(\dfrac{1}{3}\) B.\(\dfrac{\sqrt{5}}{3}\) C \(\dfrac{5}{9}\) D.\(\dfrac{5}{3}\)
Câu 2 : \(\sqrt{\dfrac{2}{x}}\) có nghĩa khi và chỉ khi là : A. x ≥ 0 B. x > 0 C. x ≠ 0 D. x ≠ 2
Câu 3 : Δ ABC vuông tại A có góc B= 300 , BC= 24cm . Độ dài AC bằng : A. 9 B. \(6\sqrt{3}\) C. \(\sqrt{18}\) D.12
Câu 4 : Kết quả phép tính \(\sqrt{9+4\sqrt{5}}\) là : A. 3-2\(\sqrt{5}\) B.2-\(\sqrt{5}\) C. \(\sqrt{5}-2\) D.\(\sqrt{5}+2\)
giải giúp mk vớiiiiiii ạ
22. Tìm nghiệm dương nhỏ nhất của PT: \(3\sin^2x+2\sin x\cos x-\cos^2x=0\)
23. Giải PT: \(\sqrt{3}\cos x+2\sin^2\left(\dfrac{x}{2}-\dfrac{\pi}{1}\right)=1\)
\(\sqrt{3}cosx+2sin^2\left(\dfrac{x}{2}-\pi\right)=1\)
\(\Leftrightarrow\sqrt{3}cosx+2sin^2\dfrac{x}{2}=1\)
\(\Leftrightarrow\sqrt{3}cosx-cosx=0\Leftrightarrow cosx=0\Leftrightarrow x=\dfrac{\pi}{2}+k\pi\) ( k thuộc Z )
Vậy ...
Đúng 0
Bình luận (0)
22.
Nhận thấy \(cosx=0\) không phải nghiệm, chia 2 vế cho \(cos^2x\)
\(3tan^2x+2tanx-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-1\\tanx=\dfrac{1}{3}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{4}+k\pi\\x=arctan\left(\dfrac{1}{3}\right)+k\pi\end{matrix}\right.\)
Nghiệm dương nhỏ nhất của pt là: \(x=arctan\left(\dfrac{1}{3}\right)\)
Đúng 0
Bình luận (0)
22. PT đã cho tương đương
3 - 4cos2x + 2 sinxcosx = 0
⇔ 3 - 2 - 2cos2x + sin2x = 0
⇔ 1 - 2cos2x + sin2x = 0
⇔ 1 + sin2x = 2cos2x
⇔ sin\(\dfrac{\pi}{2}\) + sin2x = 2cos2x
⇔ \(2sin\left(\dfrac{\pi}{4}+x\right).cos\left(\dfrac{\pi}{4}-x\right)\) = 2cos2x
Do \(\left(\dfrac{\pi}{4}-x\right)+\left(\dfrac{\pi}{4}+x\right)=\dfrac{\pi}{2}\)
⇒ \(sin\left(\dfrac{\pi}{4}+x\right)=cos\left(\dfrac{\pi}{4}-x\right)\)
Vậy sin2\(\left(x+\dfrac{\pi}{4}\right)\) = cos2x
Cái này là hiển nhiên ????
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Tìm tập nghiệm của phương trình
a/ \(x-\sqrt{2x+3}=-2x\)
b/ \(\dfrac{1}{x}=1-\dfrac{1}{x+1}\)
c/ \(\dfrac{2}{\sqrt{x+3}}=\dfrac{1}{\sqrt{x^2-9}}\)
a) \(x-\sqrt{2x+3}=-2x\)
\(\Leftrightarrow\sqrt{2x+3}=x+2x\)
\(\Leftrightarrow\sqrt{2x+3}=3x\)
\(\Leftrightarrow2x+3=9x^2\)
\(\Leftrightarrow9x^2-2x-3=0\)
\(\Rightarrow\Delta=\left(-2\right)^2-4\cdot9\cdot\left(-3\right)=112>0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{2+\sqrt{112}}{18}=\dfrac{1+2\sqrt{7}}{9}\\x_2=\dfrac{2-\sqrt{112}}{18}=\dfrac{1-2\sqrt{7}}{9}\end{matrix}\right.\)
b) \(\dfrac{1}{x}=1-\dfrac{1}{x+1}\) (ĐK: \(x\ne0,x\ne-1\))
\(\Leftrightarrow\dfrac{1}{x}+\dfrac{1}{x+1}=1\)
\(\Leftrightarrow\dfrac{x+1}{x\left(x+1\right)}+\dfrac{x}{x\left(x+1\right)}=1\)
\(\Leftrightarrow\dfrac{x+1+x}{x\left(x+1\right)}=1\)
\(\Leftrightarrow\dfrac{2x+1}{x^2+x}=1\)
\(\Leftrightarrow2x+1=x^2+1\)
\(\Leftrightarrow x^2-2x=0\)
\(\Leftrightarrow x\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x-2=0\end{matrix}\right.\)
\(\Leftrightarrow x=2\left(tm\right)\)
Đúng 2
Bình luận (0)
c) \(\dfrac{2}{\sqrt{x+3}}=\dfrac{1}{\sqrt{x^2-9}}\) (ĐK: \(x\ge3\))
\(\Leftrightarrow2\sqrt{x^2-2}=\sqrt{x+3}\)
\(\Leftrightarrow\sqrt{4\left(x^2-9\right)}=\sqrt{x+3}\)
\(\Leftrightarrow4\left(x^2-9\right)=x+3\)
\(\Leftrightarrow4x^2-36=x+3\)
\(\Leftrightarrow4x^2-x-36-3=0\)
\(\Leftrightarrow4x^2-x-39=0\)
\(\Rightarrow\Delta=\left(-1\right)^2-4\cdot4\cdot\left(-39\right)=625>0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{1+\sqrt{625}}{8}=\dfrac{13}{4}\left(tm\right)\\x_2=\dfrac{1-\sqrt{625}}{8}=-3\left(ktm\right)\end{matrix}\right.\)
Đúng 2
Bình luận (0)
B1
\(\dfrac{3x+5}{2}-1\le\dfrac{x+2}{3}+x\)
Có bnhieu nghiệm nguyên lớn hơn -10
BÀI 2 . Tập nghiệm S của btp\(\left(1-\sqrt{2}\right)x< 3-2\sqrt{2}\)
BÀI 3 \(\left(2X-1\right)\left(x+3\right)-3x+1\le\left(x+1\right)\left(x+3\right)+x^2-5\) có tập nghiệm là?
Bài 1 :
Ta có : \(\dfrac{3x+5}{2}-1\le\dfrac{x+2}{3}+x\)
\(\Leftrightarrow\dfrac{3x+5}{2}-1-\dfrac{x+2}{3}-x\le0\)
\(\Leftrightarrow\dfrac{3\left(3x+5\right)-6-2\left(x+2\right)-6x}{6}\le0\)
\(\Leftrightarrow9x+15-6-2x-4-6x\le0\)
\(\Leftrightarrow x\le-5\)
Mà \(\left\{{}\begin{matrix}x\in Z\\x>-10\end{matrix}\right.\)
Vậy \(x\in\left\{-5;-6;-7;-8;-9\right\}\)
Đúng 2
Bình luận (0)
b3\(\Leftrightarrow2x^2+5x-3-3x+1\le x^2+2x-3+x^2-5\\ \Leftrightarrow0.x\le-6\Leftrightarrow x\in\varnothing\)
Đúng 0
Bình luận (0)
Tập nghiệm của bất phương trình \(x^2+2x+\dfrac{1}{\sqrt{x+4}}>3+\dfrac{1}{\sqrt{x+4}}\) là
TXĐ: \(x>-4\)
Khi đó BPT tương đương:
\(x^2+2x>3\Leftrightarrow x^2+2x-3>0\)
\(\Rightarrow\left[{}\begin{matrix}x>1\\x< -3\end{matrix}\right.\)
Vậy tập nghiệm của BPT là: \(\left[{}\begin{matrix}x>1\\-3< x< -3\end{matrix}\right.\)
Đúng 1
Bình luận (0)
cho B=\(\dfrac{1}{\sqrt{1}+\sqrt{2}}\)+\(\dfrac{1}{\sqrt{2}+\sqrt{3}}+...+\dfrac{1}{\sqrt{98}+\sqrt{99}}+\dfrac{1}{\sqrt{99}+\sqrt{100}}\).số nghiệm của phương trình \(x^3+3Bx^2+27Bx+9B^2=0\) là ?
\(B=\dfrac{\sqrt{2}-1}{\left(\sqrt{2}-1\right)\left(\sqrt{2}+1\right)}+\dfrac{\sqrt{3}-\sqrt{2}}{\left(\sqrt{3}-\sqrt{2}\right)\left(\sqrt{3}+\sqrt{2}\right)}+...+\dfrac{\sqrt{100}-\sqrt{99}}{\left(\sqrt{100}-\sqrt{99}\right)\left(\sqrt{100}+\sqrt{99}\right)}\)
\(=\dfrac{\sqrt{2}-1}{1}+\dfrac{\sqrt{3}-\sqrt{2}}{1}+...+\dfrac{\sqrt{100}-\sqrt{99}}{1}\)
\(=\sqrt{100}-1=9\)
\(x^3+3.9x^2+3.9^2x+9^3=0\)
\(\Leftrightarrow\left(x+9\right)^3=0\)
\(\Leftrightarrow x=-9\)
Đúng 1
Bình luận (0)
1. Tìm m để pt \(\left(x^2+2x\right)^2-\left(x^2+2x\right)-m=0\)
a .có 4 nghiệm pb
b. vô ng
c. có nghiệm duy nhất
d. có nghiệm
e. có nghiệm kép
2. Biết pt: \(x+\sqrt{2x+11}=0\) có nghiệm \(x=a+b\sqrt{3}\). Tính ab
HELP ME
Bài 2.
ĐK: $x\geq \frac{-11}{2}$
$x+\sqrt{2x+11}=0\Leftrightarrow x=-\sqrt{2x+11}$
\(\Rightarrow \left\{\begin{matrix} x\leq 0\\ x^2=2x+11\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\leq 0\\ x^2-2x-11=0(*)\end{matrix}\right.\)
\(\Delta'(*)=12\)
\(\Rightarrow x=1\pm \sqrt{12}=1\pm 2\sqrt{3}\). Với điều kiện của $x$ suy ra $x=1-2\sqrt{3}$
$\Rightarrow a=1; b=-2\Rightarrow ab=-2$
Đúng 11
Bình luận (1)
Bài 1.
Đặt $x^2+2x=t$ thì PT ban đầu trở thành:
$t^2-t-m=0(1)$
Để PT ban đầu có 4 nghiệm phân biệt thì:
Trước tiên PT(1) cần có 2 nghiệm phân biệt. Điều này xảy ra khi $\Delta (1)=1+4m>0\Leftrightarrow m> \frac{-1}{4}(*)$
Với mỗi nghiệm $t$ tìm được, thì PT $x^2+2x-t=0(2)$ cần có 2 nghiệm $x$ phân biệt.
Điều này xảy ra khi $\Delta '(2)=1+t>0\Leftrightarrow t>-1$
Vậy ta cần tìm điều kiện của $m$ để (1) có hai nghiệm $t$ phân biệt đều lớn hơn $-1$
Điều này xảy ra khi \(\left\{\begin{matrix} (t_1+1)(t_2+1)>0\\ t_1+t_2+2>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} t_1t_2+t_1+t_2+1>0\\ t_1+t_2+2>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} -m+1+1>0\\ 1+2>0\end{matrix}\right.\Leftrightarrow m< 2(**)\)
Từ $(*); (**)\Rightarrow \frac{-1}{4}< m< 2$
b)
Để pt ban đầu vô nghiệm thì PT(1) vô nghiệm hoặc có 2 nghiệm $t$ đều nhỏ hơn $-1$
PT(1) vô nghiệm khi mà $\Delta (1)=4m+1<0\Leftrightarrow m< \frac{-1}{4}$
Nếu PT(1) có nghiệm thì $t_1+t_2=1>-2$ nên 2 nghiệm $t$ không thể cùng nhỏ hơn $-1$
Vậy PT ban đầu vô nghiệm thì $m< \frac{-1}{4}$
c) Để PT ban đầu có nghiệm duy nhất thì:
\(\left\{\begin{matrix} \Delta (1)=1+4m=0\\ \Delta' (2)=1+t=0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} m=-\frac{1}{4}\\ t=-1\end{matrix}\right.\).Mà với $m=-\frac{1}{4}$ thì $t=\frac{1}{2}$ nên hệ trên vô lý. Tức là không tồn tại $m$ để PT ban đầu có nghiệm duy nhất.
d)
Ngược lại phần b, $m\geq \frac{-1}{4}$
e)
Để PT ban đầu có nghiệm kép thì PT $(2)$ có nghiệm kép. Điều này xảy ra khi $\Delta' (2)=1+t=0\Leftrightarrow t=-1$
$t=-1\Leftrightarrow m=(-1)^2-(-1)=2$
Đúng 6
Bình luận (3)
Giải các phương trình sau:1) sqrt{3x^2+5x+8}-sqrt{3x^2+5x+1}12) x^2-2x-12+4sqrt{left(4-xright)left(2+xright)}03) 3sqrt{x}+dfrac{3}{2sqrt{x}}2x+dfrac{1}{2x}-74) sqrt{x}-dfrac{4}{sqrt{x+2}}+sqrt{x+2}05)left(x-7right)sqrt{dfrac{x+3}{x-7}}x+46) 2sqrt{x-4}+sqrt{x-1}sqrt{2x-3}+sqrt{4x-16}7) sqrt{x+2sqrt{x-1}}+sqrt{x-2sqrt{x-1}}dfrac{x+3}{2}Giúp mình với ajk, mink đang cần gấp
Đọc tiếp
Giải các phương trình sau:
1) \(\sqrt{3x^2+5x+8}-\sqrt{3x^2+5x+1}=1\)
2) \(x^2-2x-12+4\sqrt{\left(4-x\right)\left(2+x\right)}=0\)
3) \(3\sqrt{x}+\dfrac{3}{2\sqrt{x}}=2x+\dfrac{1}{2x}-7\)
4) \(\sqrt{x}-\dfrac{4}{\sqrt{x+2}}+\sqrt{x+2}=0\)
5)\(\left(x-7\right)\sqrt{\dfrac{x+3}{x-7}}=x+4\)
6) \(2\sqrt{x-4}+\sqrt{x-1}=\sqrt{2x-3}+\sqrt{4x-16}\)
7) \(\sqrt{x+2\sqrt{x-1}}+\sqrt{x-2\sqrt{x-1}}=\dfrac{x+3}{2}\)
Giúp mình với ajk, mink đang cần gấp
tính giới hạn của các hàm số sau:a, limx→0dfrac{sqrt{1+x}-sqrt{1-x}}{sqrt[3]{1+x}-sqrt{1-x}}b, limx→0(dfrac{1}{x}-dfrac{1}{x^2})c, limx→+∞ dfrac{x^4-x^3+11}{2x-7}d, limx→5 ( dfrac{7}{left(x-1right)^2}.dfrac{2x+1}{2x-3} )
Đọc tiếp
tính giới hạn của các hàm số sau:
a, limx→0\(\dfrac{\sqrt{1+x}-\sqrt{1-x}}{\sqrt[3]{1+x}-\sqrt{1-x}}\)
b, limx→0(\(\dfrac{1}{x}-\dfrac{1}{x^2}\))
c, limx→+∞ \(\dfrac{x^4-x^3+11}{2x-7}\)
d, limx→5 ( \(\dfrac{7}{\left(x-1\right)^2}.\dfrac{2x+1}{2x-3}\) )
a. Áp dụng công thức L'Hospital:
\(\lim\limits_{x\to 0}\frac{\sqrt{x+1}-\sqrt{1-x}}{\sqrt[3]{x+1}-\sqrt{1-x}}=\lim\limits_{x\to 0}\frac{\frac{1}{2}(x+1)^{\frac{-1}{2}}+\frac{1}{2}(1-x)^{\frac{-1}{2}}}{\frac{1}{3}(x+1)^{\frac{-2}{3}}+\frac{1}{2}(1-x)^{\frac{-1}{2}}}=\frac{1}{\frac{5}{6}}=\frac{6}{5}\)
b.
\(\lim\limits_{x\to 0}(\frac{1}{x}-\frac{1}{x^2})=\lim\limits_{x\to 0}\frac{x-1}{x^2}=-\infty\)
Đúng 1
Bình luận (0)
c. Áp dụng quy tắc L'Hospital:
\(\lim\limits_{x\to +\infty}\frac{x^4-x^3+11}{2x-7}=\lim\limits_{x\to +\infty}\frac{4x^3-3x^2}{2}=+\infty \)
d.
\(\lim\limits_{x\to 5}\frac{7}{(x-1)^2}.\frac{2x+1}{2x-3}=\frac{7}{(5-1)^2}.\frac{2.5+11}{2.5-3}=\frac{11}{16}\)
Đúng 1
Bình luận (0)