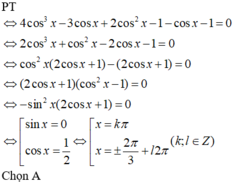cosx + cos2x + cos3x = 0
ND
Những câu hỏi liên quan
cos2x + cos4x + cos6x = 0
1 + cosx + cos2x + cos3x = 0
sin3x - sinx = cos3x - cosx
a. cos2x + cos4x + cos6x = 0
\(\Leftrightarrow\left(cos2x+cos6x\right)+cos4x=0\\ \Leftrightarrow2cos4x.cos2x+cos4x=0\\ \Leftrightarrow cos4x\left(2cos2x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}cos4x=0\\cos2x=\dfrac{-1}{2}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\left(k\in Z\right)}\)
Đúng 0
Bình luận (0)
1.
\(cos2x+cos6x+cos4x=0\)
\(\Leftrightarrow2cos4x.cos2x+cos4x=0\)
\(\Leftrightarrow cos4x\left(2cos2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos4x=0\\cos2x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=\dfrac{\pi}{2}+k\pi\\2x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
2.
\(\Leftrightarrow1+cos2x+cosx+cos3x=0\)
\(\Leftrightarrow1+2cos^2x-1+2cos2x.cosx=0\)
\(\Leftrightarrow cos^2x+cos2x.cosx=0\)
\(\Leftrightarrow cosx\left(cos2x+cosx\right)=0\)
\(\Leftrightarrow cosx\left(2cos^2x+cosx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=-1\\cosx=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k\pi\\x=\pi+k2\pi\\x=\pm\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
a, cos3x + cos2x - cosx - 1 = 0
b, cos(8sinx) = 1
c, 1 + cos2x + cosx = 0
d, 3cosx + |sinx| = 2
a/
\(\Leftrightarrow4cos^3x-3cosx+2cos^2x-1-cosx-1=0\)
\(\Leftrightarrow2cos^3x+cos^2x-2cosx-1=0\)
\(\Leftrightarrow cos^2x\left(2cosx+1\right)-\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left(cos^2x-1\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\2cosx+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=\pm\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
b/
\(cos\left(8sinx\right)=1\)
\(\Leftrightarrow8sinx=k2\pi\)
\(\Leftrightarrow sinx=\frac{k\pi}{4}\)
Do \(-1\le sinx\le1\Rightarrow-1\le\frac{k\pi}{4}\le1\)
\(\Rightarrow k=\left\{-1;0;1\right\}\)
\(\Rightarrow\left[{}\begin{matrix}sinx=-\frac{\pi}{4}\\sinx=0\\sinx=\frac{\pi}{4}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\pm arcsin\left(\frac{\pi}{4}\right)+k2\pi\\x=\pi\pm arcsin\left(\frac{\pi}{4}\right)+k2\pi\\x=k\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
c/
\(\Leftrightarrow1+2cos^2x-1+cosx=0\)
\(\Leftrightarrow2cos^2x-cosx=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=\frac{1}{2}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\x=\pm\frac{\pi}{3}+k2\pi\end{matrix}\right.\)
d/
Đặt \(\left\{{}\begin{matrix}\left|sinx\right|=a\ge0\\cosx=b\end{matrix}\right.\) ta được hệ:
\(\left\{{}\begin{matrix}a+3b=2\\a^2+b^2=1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=2-3b\\a^2+b^2=1\end{matrix}\right.\)
\(\Rightarrow\left(2-3b\right)^2+b^2-1=0\)
\(\Rightarrow10b^2-12b+3=0\Rightarrow\left[{}\begin{matrix}b=\frac{6+\sqrt{6}}{10}\Rightarrow a=\frac{2-3\sqrt{6}}{10}\left(l\right)\\b=\frac{6-\sqrt{6}}{10}\Rightarrow a=\frac{2+3\sqrt{6}}{10}\end{matrix}\right.\)
\(\Rightarrow cosx=\frac{6-\sqrt{6}}{10}\)
\(\Rightarrow x=\pm arccos\left(\frac{6-\sqrt{6}}{10}\right)+k2\pi\)
Đúng 0
Bình luận (0)
cosx + cos2x + cos3x + 1 = 0
Lời giải:
\(\cos x+\cos 2x+\cos 3x+1=0\)
\(\Leftrightarrow (\cos 2x+1)+\cos x+\cos 3x=0\)
\(\Leftrightarrow (2\cos ^2x-1+1)+2.\cos \frac{3x+x}{2}\cos \frac{3x-x}{2}=0\)
\(\Leftrightarrow 2\cos ^2x+2\cos 2x\cos x=0\)
\(\Leftrightarrow \cos x(\cos x+\cos 2x)=0\)
TH1: $\cos x=0$
$\Rightarrow x=\frac{\pi}{2}+k\pi$ (với $k$ nguyên)
TH2: $\cos x+\cos 2x=0$
$\Leftrightarrow \cos x+2\cos ^2x-1=0$
$\Leftrightarrow (2\cos x-1)(\cos x+1)=0$
$\Rightarrow \cos x=\frac{1}{2}$ hoặc $\cos x=-1$
$\Rightarrow x=\pm \frac{\pi}{3}+2k\pi$ với $k$ nguyên
Hoặc $x=(2k+1)\pi$ với $k$ nguyên
Đúng 0
Bình luận (0)
sinx + sin2x + sin3x = 1 + cosx + cos2x
cos3x + sin3x + cosx - sinx = \(\sqrt{2}\)cos2x
sinx + sin2x + sin3x = cosx + cos2x + cos3x
b: \(\Leftrightarrow2\cdot\cos2x\cdot\cos x+2\cdot\sin x\cdot\cos2x=\sqrt{2}\cdot\cos2x\)
\(\Leftrightarrow2\cdot\cos2x\left(\sin x+\cos x\right)=\sqrt{2}\cdot\cos2x\)
\(\Leftrightarrow\sqrt{2}\cdot\cos2x\cdot\left[\sqrt{2}\cdot\sqrt{2}\cdot\sin\left(x+\dfrac{\Pi}{4}\right)-1\right]=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\cos2x=0\\\sin\left(x+\dfrac{\Pi}{4}\right)=\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\Pi}{2}+k\Pi\\x+\dfrac{\Pi}{4}=\dfrac{\Pi}{6}+k2\Pi\\x+\dfrac{\Pi}{4}=\dfrac{5}{6}\Pi+k2\Pi\end{matrix}\right.\)
\(\Leftrightarrow x\in\left\{\dfrac{\Pi}{4}+\dfrac{k\Pi}{2};\dfrac{-1}{12}\Pi+k2\Pi;\dfrac{7}{12}\Pi+k2\Pi\right\}\)
c: \(\Leftrightarrow2\cdot\sin2x\cdot\cos x+\sin2x=2\cdot\cos2x\cdot\cos x+\cos2x\)
\(\Leftrightarrow\sin2x\left(2\cos x+1\right)=\cos2x\left(2\cos x+1\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}\sin2x=\cos2x=\sin\left(\dfrac{\Pi}{2}-2x\right)\\\cos x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Pi}{8}+\dfrac{k\Pi}{4}\\\\x=-\dfrac{2}{3}\Pi+k2\Pi\\x=\dfrac{2}{3}\Pi+k2\Pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Giải phương trình cos3x + cos2x - cosx - 1 = 0
![]()
![]()
![]()
![]()
Số nghiệm thuộc nửa khoảng
-
π
;
0
của phương trình
cos
x
-
cos
2
x
-
cos
3
x
0
là
Đọc tiếp
Số nghiệm thuộc nửa khoảng - π ; 0 của phương trình cos x - cos 2 x - cos 3 x = 0 là
![]()
![]()
![]()
![]()
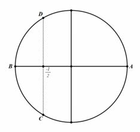
Số nghiệm thuộc nửa khoảng [ - π ; 0 ) của phương trình cosx-cos2x-cos3x+1 = 0 là
A. 3.
B. 1.
C. 4.
D. 2.
Chọn D
Phương trình tương với:
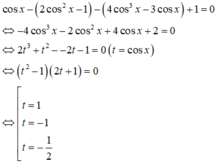
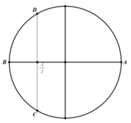
Trên đường tròn đơn vị, các điểm nghiệm của phương trình là 4 điểm A, B, C, D như hình vẽ. Do đó trên nửa khoảng [ - π ; 0 ) , phương trình có đúng 2 nghiệm (là - π và - 2 π 3 ).
Đúng 0
Bình luận (0)
Số nghiệm thuộc nửa khoảng
[
−
π
;
0
)
của phương trình
cos
x
−
cos
2
x
−
cos
3
x
+
1
0
là A. 3 B. 1 C. 4 D. 2
Đọc tiếp
Số nghiệm thuộc nửa khoảng [ − π ; 0 ) của phương trình cos x − cos 2 x − cos 3 x + 1 = 0 là
A. 3
B. 1
C. 4
D. 2
Đáp án D.
Phương trình tương với:
cos x − 2 cos 2 x − 1 − 4 cos 3 x − 3 cos x + 1 = 0 ⇔ − 4 cos 3 x − 2 cos 2 x + 4 cos x + 2 = 0 ⇔ 2 t 3 + t 2 − − 2 t − 1 = 0 t = cos x ⇔ t 2 − 1 2 t + 1 = 0 ⇔ t = 1 t = − 1 t = − 1 2
Trên đường tròn đơn vị, các điểm nghiệm của phương trình là 4 điểm A, B, C, D như hình vẽ. Do đó trên nửa khoảng − π ; 0 , phương trình có đúng 2 nghiệm (là − π và − 2 π 3 ).
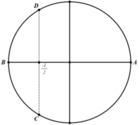
Đúng 0
Bình luận (0)
Giải các phương trình sau:
a, cos3x-4cos2x+3cosx-4=0, ∀x∈[0;14]
b, (2cosx-1)(2cos+cosx)=sin2x-sinx
c, cos3x+cos2x+1+sin2x+cos2x=0
@Nguyễn Việt Lâm giúp em với ạ
a/ \(4cos^3x-3cosx-4\left(2cos^2x-1\right)+3cosx-4=0\)
\(\Leftrightarrow4cos^3x-8cos^2x=0\)
\(\Leftrightarrow4cos^2x\left(cosx-2\right)=0\)
\(\Leftrightarrow cosx=0\Rightarrow x=\frac{\pi}{2}+k\pi\)
\(0< \frac{\pi}{2}+k\pi< 14\Rightarrow-\frac{1}{2}< k< \frac{14-\frac{\pi}{2}}{\pi}\Rightarrow k=\left\{0;1;2;3\right\}\)
\(\Rightarrow x=\left\{\frac{\pi}{2};\frac{3\pi}{2};\frac{5\pi}{2};\frac{7\pi}{2}\right\}\)
b/ Bạn coi lại đề, cái ngoặc thứ 2 thiếu \(\left(2cos\left(???\right)+cosx\right)\)
c/ Bạn coi lại đề, có 2 số hạng \(cos2x\) xuất hiện ở vế trái, cấp 3 chắc ko ai cho kiểu vậy đâu, nếu đúng thế thì người ta cộng luôn thành \(2cos2x\) cho rồi
Đúng 0
Bình luận (0)