Tìm x biết
a)x3=216
b)3x2=x2
c)x5=x4
H24
Những câu hỏi liên quan
Bài 5: Thu gọn các đa thức và sắp xếp theo lũy hừa tăng (hoặc giảm)của biến:
a) x5 - 3x2 + x4 - 1/2x - x5 + 5x4 + x2 - 1
b) x7 - x4 +2x3 - 3x4 -x2 + x7 - x + 5 - x3
Tính f(x) + g(x) với:
f(x) = x5 – 3x2 + x3 – x2 – 2x + 5
g(x) = x2 – 3x + 1 + x2 – x4 + x5
Thu gọn, sắp xếp đa thức theo lũy thừa giảm của biến:
* Ta có: f(x) = x5 – 3x2 + x3 – x2 – 2x + 5
= x5 – (3x2 + x2 ) + x3 - 2x + 5
= x5 – 4x2 + x3 – 2x + 5
= x5 + x3 – 4x2 – 2x + 5
Và g(x) = x2 – 3x + 1 + x2 – x4 + x5
= (x2 + x2 ) – 3x + 1 – x4 + x5
= 2x2 – 3x + 1 – x4 + x5
= x5 – x4 + 2x2 – 3x + 1
* f(x) + g(x):
Đúng 0
Bình luận (0)
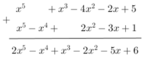
Tính f(x) - g(x) với:
f(x) = x5 – 3x2 + x3 – x2 – 2x + 5
g(x) = x2 – 3x + 1 + x2 – x4 + x5
giúp với ạ
\(f\left(x\right)-g\left(x\right)=\left(x^5-3x^2+x^3-x^2-2x+5\right)-\left(x^2-3x+1+x^2-x^4+x^5\right)\)
\(f\left(x\right)-g\left(x\right)=x^5-3x^2+x^3-x^2-2x+5-x^2+3x-1-x^2+x^4-x^5\)
\(f\left(x\right)-g\left(x\right)=\left(x^5-x^5\right)+\left(-3x^2-x^2-x^2-x^2\right)+x^3+\left(-2x+3x\right)+\left(5-1\right)+x^4\)
\(f\left(x\right)-g\left(x\right)=-6x^2+x^3+x+4+x^4\)
\(f\left(x\right)-g\left(x\right)=x^4+x^3-6x^2+x+4\)
Đúng 0
Bình luận (4)
Cho đa thức
F(x)=x5 - 3x2 -x3 - x2 - 2x + 5
G(x)+x5 - x4 + x2 - 3x + x2 + 1
Tính H(x) = F(x) + G(x)
\(H\left(x\right)=F\left(x\right)+G\left(x\right)=\left(x^5-3x^2-x^3-x^2-2x+5\right)+\left(x^5-x^4+x^2-3x+x^2+1\right)\\ =x^5-3x^2-x^3-x^2-2x+5+x^5-x^4+x^2-3x+x^2+1\\ =\left(x^5+x^5\right)-x^4-x^3-\left(3x^2+x^2-x^2-x^2\right)-\left(2x+3x\right)+5\\ =2x^5-x^4-x^3-2x^2-5x+5\)
Đúng 0
Bình luận (0)
Bài 1: Giải phương trình:
a) ( x+1)2 (x+2) + ( x – 1)2 ( x- 2) = 12
b) x4 + 3x3 + 4x2 + 3x + 1 = 0
c) x5 – x4 + 3x3 + 3x2 –x + 1 = 0
Bài 2: Chứng minh rằng các phương trình sau vô nghiệm
a) x4 – x3 + 2x2 – x + 1 = 0
b) x4 + x3 + x2 + x + 1 = 0
c) x4 – 2x3 +4x2 – 3x +2 = 0
d) x6+ x5+ x4 + x3 + x2 + x + 1 = 0
1.
a/ \(\Leftrightarrow\left(x+1\right)\left(x^2+3x+2\right)+\left(x-1\right)\left(x^2-3x+2\right)-12=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2\right)+3x\left(x+1\right)-3x\left(x-1\right)+\left(x-1\right)\left(x^2+2\right)-12=0\)
\(\Leftrightarrow2x\left(x^2+2\right)+6x^2-12=0\)
\(\Leftrightarrow x^3+3x^2+2x-6=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2+4x+6\right)=0\Rightarrow x=1\)
b/ Nhận thấy \(x=0\) ko phải nghiệm, chia 2 vế cho \(x^2\)
\(x^2+\frac{1}{x^2}+3\left(x+\frac{1}{x}\right)+4=0\)
Đặt \(x+\frac{1}{x}=t\Rightarrow x^2+\frac{1}{x^2}=t^2-2\)
\(t^2-2+3t+4=0\Rightarrow t^2+3t+2=0\Rightarrow\left[{}\begin{matrix}t=-1\\t=-2\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x+\frac{1}{x}=-1\\x+\frac{1}{x}=-2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2+x+1=0\left(vn\right)\\x^2+2x+1=0\end{matrix}\right.\) \(\Rightarrow x=-1\)
1c/
\(\Leftrightarrow x^5+x^4-2x^4-2x^3+5x^3+5x^2-2x^2-2x+x+1=0\)
\(\Leftrightarrow x^4\left(x+1\right)-2x^3\left(x+1\right)+5x^2\left(x+1\right)-2x\left(x+1\right)+x+1=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^4-2x^3+5x^2-2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^4-2x^3+5x^2-2x+1=0\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow x^4-2x^3+x^2+x^2-2x+1+3x^2=0\)
\(\Leftrightarrow\left(x^2-x\right)^2+\left(x-1\right)^2+3x^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-x=0\\x-1=0\\x=0\end{matrix}\right.\) \(\Rightarrow\) không tồn tại x thỏa mãn
Vậy pt có nghiệm duy nhất \(x=-1\)
2.
a. \(x^4-x^3+x^2+x^2-x+1=0\)
\(\Leftrightarrow x^2\left(x^2-x+1\right)+x^2-x+1=0\)
\(\Leftrightarrow\left(x^2+1\right)\left(x^2-x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2+1=0\left(vn\right)\\x^2-x+1=0\Leftrightarrow\left(x-\frac{1}{2}\right)^2+\frac{3}{4}=0\left(vn\right)\end{matrix}\right.\)
Vậy pt vô nghiệm
b.
\(x^4+x^3+x^2+x+1=0\)
\(\Leftrightarrow x\left(x^3+1\right)+x^3+1+x^2=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^3+1\right)+x^2=0\)
\(\Leftrightarrow\left(x+1\right)^2\left(x^2-x+1\right)+x^2=0\)
Mà \(\left\{{}\begin{matrix}\left(x+1\right)^2\left(x^2-x+1\right)\ge0\\x^2\ge0\end{matrix}\right.\)
Nên dấu "=" xảy ra khi và chỉ khi: \(\left\{{}\begin{matrix}x+1=0\\x=0\end{matrix}\right.\) ko tồn tại x thỏa mãn
Xem thêm câu trả lời
Bài 1 : Cho a,b,c là các số hữu tỉ khác 0 sao cho a+b-c/c=a-b+c/b=(-a)+b+c/a
Tính giá trị của biểu thức A=(a+b).(b+c).(c+a)/abc
(LƯU Ý : DẤU / LÀ ...TRÊN.....)
Bài 2 : Cho x,x2,x3,x4,x5,x6 thỏa mãn :
(x2)^2=x1.x3
(x3)^2=x2.x4
(x4)^2=x3.x5
(x5)^2=x4.x6
Chứng minh rằng : x1/x6=(x1+x2+x3+x4+x5/x2+x3+x4+x5+x6)^5
Giusp mk vs nhé các bn !!!
tìm X1, X2,X3,X4,X5,X6,BIẾT:
X1+X2=X3+X4=X5+X6=2,BIẾT :
X1+X2+X3+X4+X5+X6=0
X1+X2=X3+X4=X5+X6=2
nên X1+X2+X3+X4+X5+X6=0
2+2+2=0
6=0(loại)
vậy không có giá trị nào thỏa mãn đề
Đúng 0
Bình luận (0)
Tính f(x) + g(x) với:
f(x) = x5 – 3x2 + x3 – x2 – 2x + 5
g(x) = x2 – 3x + 1 + x2 – x4 + x5
Thu gọn, sắp xếp đa thức theo lũy thừa giảm của biến:
* Ta có: f(x) = x5 – 3x2 + x3 – x2 – 2x + 5 = x5 + x3 – 4x2 – 2x + 5
g(x) = x2 – 3x + 1 + x2 – x4 + x5 = x5 – x4 + 2x2 – 3x + 1
* f(x) + g(x):
Đúng 1
Bình luận (0)
Thu gọn, sắp xếp đa thức theo lũy thừa giảm của biến:
* Ta có: f(x) = x5 – 3x2 + x3 – x2 – 2x + 5 = x5 + x3 – 4x2 – 2x + 5
g(x) = x2 – 3x + 1 + x2 – x4 + x5 = x5 – x4 + 2x2 – 3x + 1
* f(x) + g(x): tự làm nha bạn
Đúng 0
Bình luận (0)
Cho các đa thức f(x) = x5 – 3x2 + x3 – x2 - 2x + 5
g(x) = x5 – x4 + x2 - 3x + x2 + 1
a) Thu gọn và sắp xếp đa thức f(x) và g(x) theo luỹ thừa giảm dần.
b)Tính h(x) = f(x) + g(x)
a: \(F\left(x\right)=x^5-3x^2+x^3-x^2-2x+5\)
\(=x^5+x^3-4x^2-2x+5\)
\(G\left(x\right)=x^5-x^4+x^2-3x+x^2+1\)
\(=x^5-x^4+2x^2-3x+1\)
b: Ta có: \(H\left(x\right)=F\left(x\right)+G\left(x\right)\)
\(=x^5+x^3-4x^2-2x+5+x^5-x^4+2x^2-3x+1\)
\(=2x^5-x^4+x^3-2x^2-5x+6\)
Đúng 1
Bình luận (0)
Giả sử đa thức
P
(
x
)
x
5
-
a
x
4
+
b
có năm nghiệm
x
1
;
x
2
;
x
3
;
x
4
;
x
5
Đặt
f
(
x
)...
Đọc tiếp
Giả sử đa thức P ( x ) = x 5 - a x 4 + b có năm nghiệm x 1 ; x 2 ; x 3 ; x 4 ; x 5 Đặt f ( x ) = x 2 - 4 Tìm giá trị nhỏ nhất của P = f ( x 1 ) f ( x 2 ) f ( x 3 ) f ( x 4 ) f ( x 5 )
A. 512
B. -512
C. 1024
D. -1024
