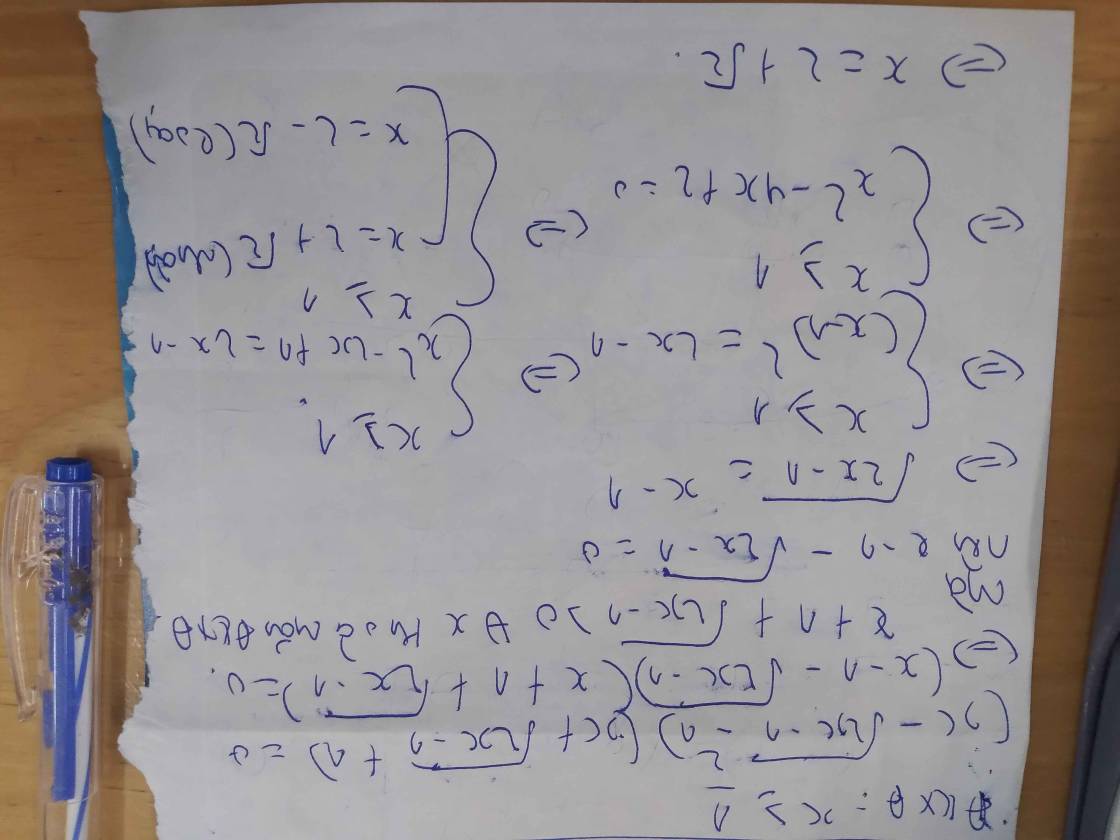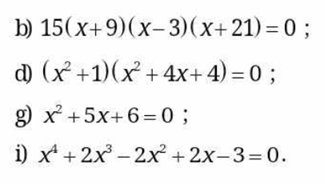cho 3 số a,b,c thỏa mãn : a+b+c=0 ; a2+b2+c2=2009. tính A= a4+b4+c4
Bài 4: Phương trình tích
Ta có: a + b + c = 0
\(\Rightarrow\) (a + b + c)2 = 0
\(\Leftrightarrow\) a2 + b2 + c2 + 2ab + 2bc + 2ac = 0
\(\Leftrightarrow\) 2009 + 2(ab + bc + ac) = 0
\(\Leftrightarrow\) ab + bc + ac = \(\dfrac{-2009}{2}\)
\(\Leftrightarrow\) (ab + bc + ac)2 = \(\left(\dfrac{-2009}{2}\right)^2\)
\(\Leftrightarrow\) a2b2 + b2c2 + a2c2 + 2abc(a + b + c) = \(\left(\dfrac{-2009}{2}\right)^2\)
\(\Leftrightarrow\) a2b2 + b2c2 + c2a2 = \(\left(\dfrac{-2009}{2}\right)^2\) (Vì a + b + c = 0)
Lại có: a2 + b2 + c2 = 2009
\(\Rightarrow\) (a2 + b2 + c2)2 = 20092
\(\Leftrightarrow\) a4 + b4 + c4 + 2(a2b2 + b2c2 + c2a2) = 20092
\(\Leftrightarrow\) a4 + b4 + c4 + 2.\(\dfrac{2009^2}{4}\) = 20092
\(\Leftrightarrow\) a4 + b4 + c4 = 20092 - \(\dfrac{2009^2}{2}\) = 2018040,5
Chúc bn học tốt!
Đúng 1
Bình luận (0)
( x - √2x - 1 -1)( x + √2x -1 + 1) = 0
Giải các phương trình bậc hai sau bằng cách đưa về dạng phương trình tích:
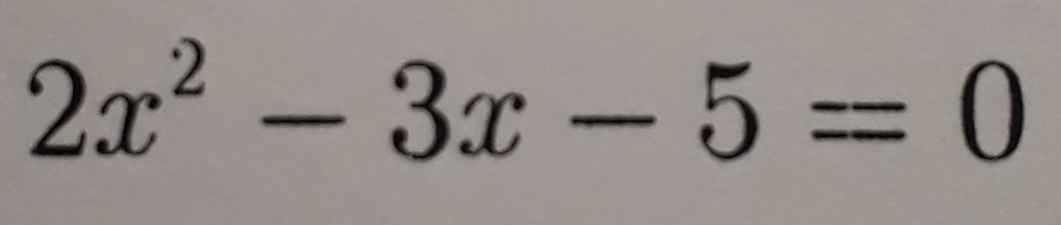
\(2x^2-3x-5=0 \\ \Leftrightarrow2x^2+2x-5x-5=0\\ \Leftrightarrow2x\left(x+1\right)-5\left(x+1\right)=0\\ \Leftrightarrow\left(2x-5\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}2x-5=0\\x+1=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}2x=5\\x=-1\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-1\end{matrix}\right.\\ Vậy.S=\left\{\dfrac{5}{2};-1\right\}\)
Đúng 2
Bình luận (0)
\(2x^2-3x-5=0\)
\(\Leftrightarrow2x^2+2x-5x-5=0\)
\(\Leftrightarrow2x\left(x+1\right)-5\left(x+1\right)=0\)
\(\Leftrightarrow\left(2x-5\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-5=0\\x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-1\end{matrix}\right.\)
Vậy \(x=\dfrac{5}{2};x=-1\) là các nghiệm của phương trình.
#\(Toru\)
Đúng 1
Bình luận (0)
b: =>(x+9)(x-3)(x+21)=0
=>x+9=0; x-3=0; x+21=0
=>x=-9; x=3;x=-21
g: =>(x+2)(x+3)=0
=>x=-2 hoặc x=-3
d: =>x^2+4x+4=0
=>(x+2)^2=0
=>x+2=0
=>x=-2
Đúng 0
Bình luận (0)
giải pt
0=(x-2)^2(x+2)
\(0=\left(x-2\right)^2\left(x+2\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(x-2\right)^2=0\\x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)
Đúng 2
Bình luận (0)
Trong các pt sau, pt tích là
A.(x-2)^2(x+2)=2
B.0=(x-2)^2(x+2)
C.(x-2)^2(x+2)=2(x+2)
D. (x-2)^2(x+2)+(x+2)
Trong các pt sau, pt tích là
A.(x-2)^2(x+2)=2
B.0=(x-2)^2(x+2)
C.(x-2)^2(x+2)=2(x+2)
D. (x-2)^2(x+2)+(x+2)
Đúng 0
Bình luận (1)
B. 0 = (x - 2)²(x + 2)
C. (x - 2)²(x + 2) = 2(x + 2)
Đúng 0
Bình luận (0)
2x^2+8x-10=0
\(2x^2+8x-10=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-5\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Phương trình nào sau đây là ptrinh tích ?
A.2x-(x+3)
B.2x+3=0
C.14x+7=0
D.(x-5)(x-3)=0
Giải pt sau:
2(x2 - x) - x(x + 2) + 4 = 0
Giúp mik vs mọi người ơi
\(2\left(x^2-x\right)-x\left(x+2\right)+4=0\)
\(\Leftrightarrow2x^2-2x-x^2-2x+4=0\)
\(\Leftrightarrow x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
\(\Leftrightarrow x=2\)
Vậy \(S=\left\{2\right\}\)
Đúng 2
Bình luận (0)
Giải phương trình sau:
(x2 - 2) - (x - 2)(3x - 7) = 0
\(\left(x^2-2\right)-\left(x-2\right)\left(3x-7\right)=0\\ \Leftrightarrow x^2-2-\left(3x^2-6x-7x+14\right)=0\\ \Leftrightarrow x^2-2-3x^2+13x-14=0\\ \Leftrightarrow-2x^2+13x-16=0\\\Leftrightarrow \left[{}\begin{matrix}x=\dfrac{13+\sqrt{41}}{4}\\x=\dfrac{13-\sqrt{41}}{4}\end{matrix}\right.\)
Đúng 1
Bình luận (0)