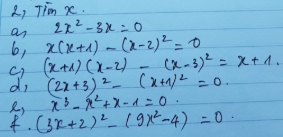16:
a: \(x^2+4x-y^2+4\)
\(=\left(x^2+4x+4\right)-y^2\)
\(=\left(x+2\right)^2-y^2\)
\(=\left(x+2+y\right)\left(x+2-y\right)\)
b: \(9-x^2-2xy-y^2\)
\(=9-\left(x^2+2xy+y^2\right)\)
\(=3^2-\left(x+y\right)^2\)
\(=\left(3-x-y\right)\left(3+x+y\right)\)
c: \(x^2-y^2+4x+4\)
\(=x^2+4x+4-y^2\)
\(=\left(x+2\right)^2-y^2\)
\(=\left(x+2+y\right)\left(x+2-y\right)\)
19:
a: \(8x^3-36x^2+54x-27\)
\(=\left(2x\right)^3-3\left(2x\right)^2\cdot3+3\cdot2x\cdot3^2-3^3\)
\(=\left(2x-3\right)^3\)
b: \(27x^3-27x^2+9x-1\)
\(=\left(3x\right)^3-3\cdot\left(3x\right)^2\cdot1+3\cdot3x\cdot1^2-1^3\)
\(=\left(3x-1\right)^3\)
c: \(x^3+6x^2y+12xy^2+8y^3\)
\(=x^3+3\cdot x^2\cdot2y+3\cdot x\cdot\left(2y\right)^2+\left(2y\right)^3\)
\(=\left(x+2y\right)^3\)
17:
a: \(x^3+9x^2+27x+27\)
\(=x^3+3\cdot x^2\cdot3+3\cdot x\cdot3^2+3^3\)
\(=\left(x+3\right)^3\)
b: \(x^3+3x^2+3x+1\)
\(=x^3+3\cdot x^2\cdot1+3\cdot x\cdot1^2+1^3\)
\(=\left(x+1\right)^3\)
c: \(8-12x+6x^2-x^3\)
\(=2^3-3\cdot2^2\cdot x+3\cdot2\cdot x^2-x^3\)
\(=\left(2-x\right)^3\)
18:
a: \(x^3-9x^2+27x-27\)
\(=x^3-3\cdot x^2\cdot3+3\cdot x\cdot3^2-3^3\)
\(=\left(x-3\right)^3\)
b: \(8x^3+12x^2+6x+1\)
\(=\left(2x\right)^3+3\cdot\left(2x\right)^2\cdot1+3\cdot2x\cdot1^2+1^3\)
\(=\left(2x+1\right)^3\)
c: \(27x^3+54x^2+36x+8\)
\(=\left(3x\right)^3+3\cdot\left(3x\right)^2\cdot2+3\cdot3x\cdot2^2+2^3\)
\(=\left(3x+2\right)^3\)




 Không làm tắt
Không làm tắt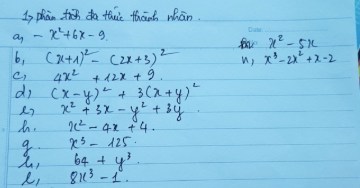 Ko làm tắt
Ko làm tắt