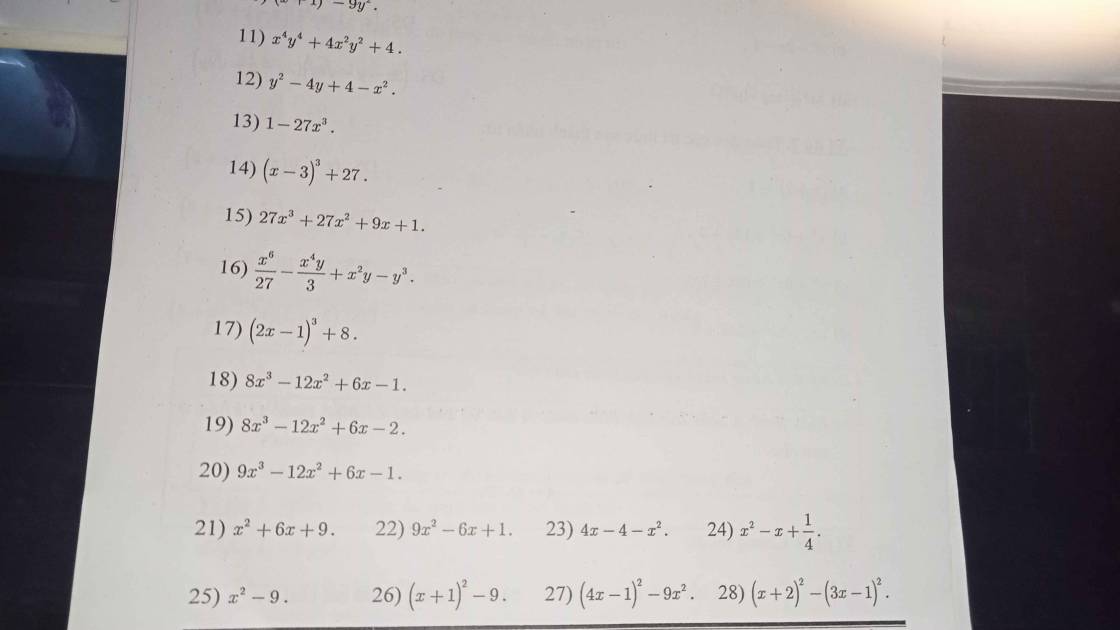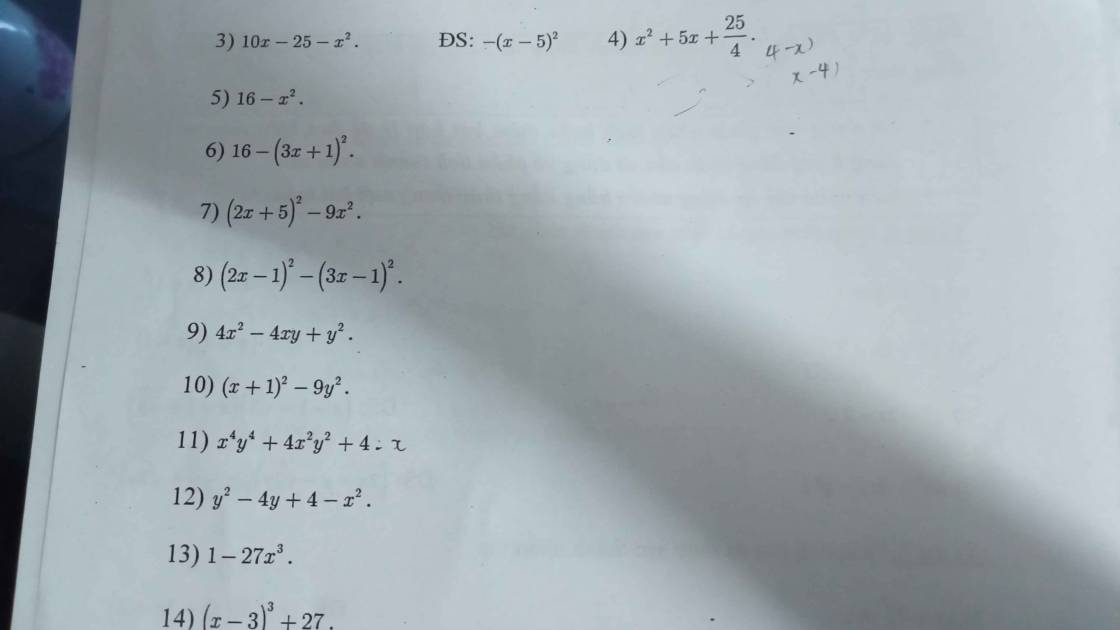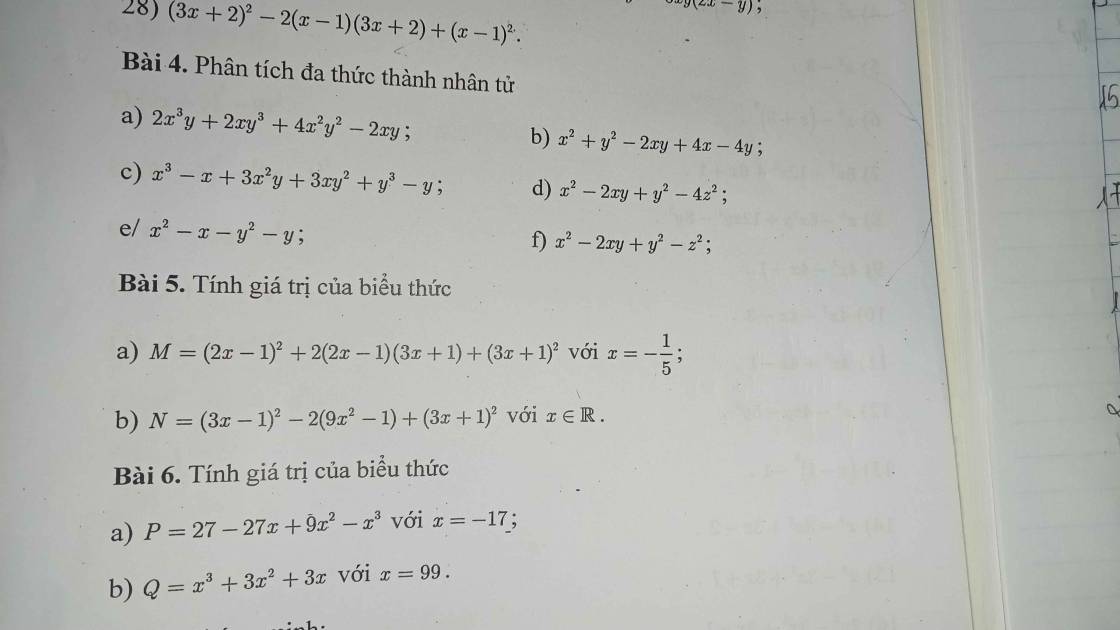Bài 7

Ta có:

VT = a³ + b³ = (a + b)(a² - ab + b²)

= (a + b)(a² - 2ab + b² + ab)

= (a + b)[(a - b)² + ab]

= VP

Vậy a³ + b³ = (a + b)[(a - b)² + ab]

Bài 8

(x + 2)³ + (x - 2)³ + x³ - 3x(x + 2)(x - 2)

= x³ + 6x² + 12x + 8 + x³ - 6x² + 12x - 8 + x³ - 3x(x² - 4)

= 3x³ + 24x - 3x³ + 12x

= 36x

22:

A=9x^2+12x+4+4x^2-28x+49-2(6x^2+15x+4x+10)

=13x^2-16x+53-12x^2-38x-20

=x^2-54x+33

=(-19)^2-54*(-19)+33=1420

Bài 4 : 

\(A=5\left(x+3\right)\left(x-3\right)+\left(2x+3\right)^2+\left(x-6\right)^2\)

\(A=5\left(x^2-9\right)+\left(4x^2+12x+9\right)+\left(x^2-12x+36\right)\)

\(A=5x^2-45+4x^2+12x+9+x^2-12x+36\)

\(A=10x^2\)

Với x = -1/5 => A = \(10.\left(\dfrac{-1}{5}\right)^2=\dfrac{2}{5}\)

  bài đây ạ

Bài 3: 

1) \(x^2-25=x^2-5^2=\left(x+5\right)\left(x-5\right)\)

2) \(9x^2-\dfrac{1}{16}y^2=\left(3x\right)^2-\left(\dfrac{1}{4}y\right)^2=\left(3x+\dfrac{1}{4}y\right)\left(3x-\dfrac{1}{4}y\right)\)

3) \(x^6-y^4=\left(x^3\right)^2-\left(y^2\right)^2=\left(x^3+y^2\right)\left(x^3-y^2\right)\)

4) \(\left(2x-5\right)^2-64=\left(2x-5\right)^2-8^2=\left[\left(2x-5\right)+8\right]\left[\left(2x-5\right)-8\right]=\left(2x+3\right)\left(2x-13\right)\)

5) \(81-\left(3x+2\right)^2=9^2-\left(3x+2\right)=\left[9-\left(3x+2\right)\right]\left[9+\left(3x+2\right)\right]=\left(7-3x\right)\left(11+3x\right)\)

  đây a 

  đây nha

17:x^2-9=(x-3)(x+3)

18: 4x^2-25=(2x-5)(2x+5)

19: =(x^2-y^2)(x^2+y^2)

=(x-y)(x+y)(x^2+y^2)

20: 9x^2+6xy+y^2=(3x+y)^2

21 6x-9-x^2

=-(x^2-6x+9)

=-(x-3)^2

22: x^2+4xy+4y^2

=x^2+2*x*2y+(2y)^2

=(x+2y)^2

23: =(x+y+x-y)(x+y-x+y)

=2x*2y=4xy

25: =(3x+1+x+1)(3x+1-x-1)

=(4x+2)*2x

=4x(2x+1)

27: =(2x-y)(4x^2+2xy+y^2)-6xy(2x-y)

=(2x-y)(2x-y)^2

=(2x-y)^3

  đây a 

Yêu cầu của đề là gì vậy ?

11: =(x^2y^2)^2+2*x^2y^2*2+2

=(x^2y^2+2)^2

12: =(y-2)^2-x^2

=(y-2-x)(y-2+x)

13: =(1-3x)(1+3x+9x^2)

14: =(x-3+3)(x^2-6x+9-3x+9+9)

=x(x^2-3x+9)

15: =(3x+1)^3

16: =(1/3x^2-y)^3

17: =(2x-1+2)(4x^2-4x+1-4x+2+4)

=(2x+1)(4x^2-8x+7)

18: =(2x-1)^3

19: =(2x-1)^3-1

=(2x-1-1)(4x^2-4x+1+2x-1+1)

=(2x-2)(4x^2-2x+1)

=2(x-1)(4x^2-2x+1)

  đây a 

  ạ

14

\(=\left(x-3\right)^3+3^3=\left(x-3+3\right)\left[\left(x-3\right)^2-\left(x-3\right).3+3^2\right]\\ =x\left(x^2-6x+9-3x+9+9\right)=x\left(x^2-9x+27\right)\)

3: =-(x^2-10x+25)

=-(x-5)^2

5: 16-x^2=(4-x)(4+x)

6: 16-(3x+1)^2

=(4-3x-1)(4+3x+1)

=(3x+5)*3(1-x)

7: =(2x+5+3x)(2x+5-3x)

=(5-x)*5*(x+10

8: =(2x-1-3x+1)(2x-1+3x-1)

=-x(5x-2)

9: =(2x-y)^2

10: =(x+1+3y)(x+1-3y)

12: =(y-2)^2-x^2

=(y-2-x)(y-2+x)

  làm 1 bài cx đc hết càng tốt ạ

Bài 5:

a) \(M=\left(2x-1\right)^2+2\left(2x-1\right)\left(3x+1\right)+\left(3x+1\right)^2\)

\(M=\left[\left(2x-1\right)+\left(3x+1\right)\right]^2\)

\(M=\left(5x\right)^2\)

Thay \(x=-\dfrac{1}{5}\) vào biểu thức M ta có:

\(\left(5\cdot-\dfrac{1}{5}\right)^2=\left(-1\right)^2=1\)

Vậy: ...

b) \(N=\left(3x-1\right)^2-2\left(9x^2-1\right)+\left(3x+1\right)^2\)

\(N=\left(3x-1\right)^2-2\left(3x+1\right)\left(3x-1\right)+\left(3x+1\right)^2\)

\(N=\left[\left(3x-1\right)-\left(3x+1\right)\right]^2\)

\(N=\left(-2\right)^2=4\)

Vậy: ...

4

a

\(=2xy\left(x^2+y^2+2xy-1\right)=2xy\left[\left(x+y\right)^2-1^2\right]\\ =2xy\left(x+y-1\right)\left(x+y+1\right)\)

b

\(=\left(x-y\right)^2+4\left(x-y\right)=\left(x-y\right)\left(x-y+4\right)\)

c

\(=\left(x+y\right)^3-\left(x+y\right)\\ =\left(x+y\right)\left[\left(x+y\right)^2-1\right]=\left(x+y\right)\left(x+y-1\right)\left(x+y+1\right)\)

d

\(=\left(x-y\right)^2-\left(2z\right)^2=\left(x-y-2z\right)\left(x-y+2z\right)\)

e

\(=\left(x-y\right)\left(x+y\right)-\left(x+y\right)=\left(x+y\right)\left(x-y-1\right)\)

f

\(=\left(x-y\right)^2-z^2=\left(x-y-z\right)\left(x-y+z\right)\)

a) (x + 2)³ + 1

= (x + 2)³ + 1³

= [(x + 2) + 1][(x + 2)² - (x + 2).1 + 1²]

= (x + 3)(x² + 4x + 4 - x - 2 + 1)

= (x + 3)(x² + 3x + 3)

b) x³ + 6x² + 12x + 9

= x³ + 3x² + 3x² + 9x + 3x + 9

= (x³ + 3x²) + (3x² + 9x) + (3x + 9)

= x²(x + 3) + 3x(x + 3) + 3(x + 3)

= (x + 3)(x² + 3x + 3)

c) x³ + 6x² + 12x + 7

= x³ + x² + 5x² + 5x + 7x + 7

= (x³ + x²) + (5x² + 5x) + (7x + 7)

= x²(x + 1) + 5x(x + 1) + 7(x + 1)

= (x + 1)(x² + 5x + 7)

d) 2x³ + 6x² + 12x + 8

= 2(x³ + 3x² + 6x + 4)

= 2(x³ + x² + 2x² + 2x + 4x + 4)

= 2[(x³ + x²) + (2x² + 2x) + (4x + 4)]

= 2[x²(x + 1) + 2x(x + 1) + 4(x + 1)]

= 2(x + 1)(x² + 2x + 4)

a: =(x+2+1)(x^2+4x+4-x-2+1)

=(x+3)(x^2+3x+3)

b: =x^3+6x^2+12x+8+1

=(x+2)^3+1

=(x+3)(x^2+3x+3)

c: =x^3+x^2+5x^2+5x+7x+7

=(x+1)(x^2+5x+7)