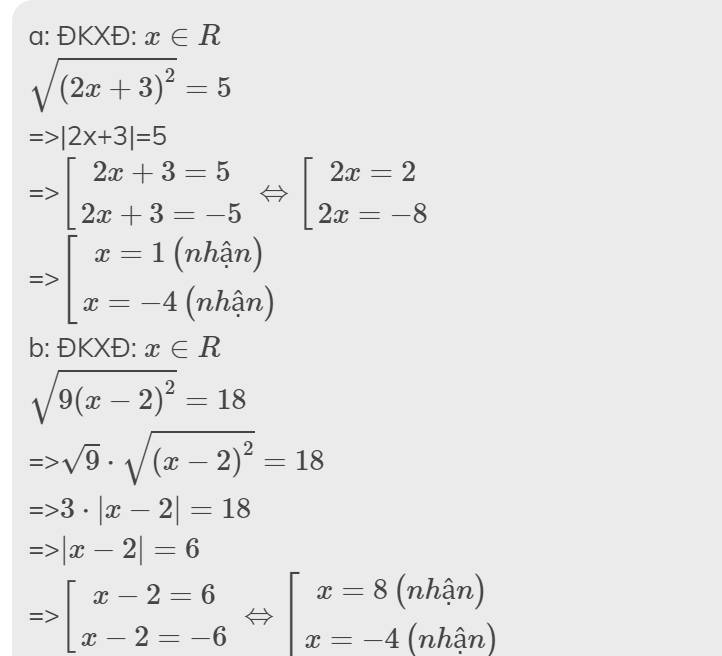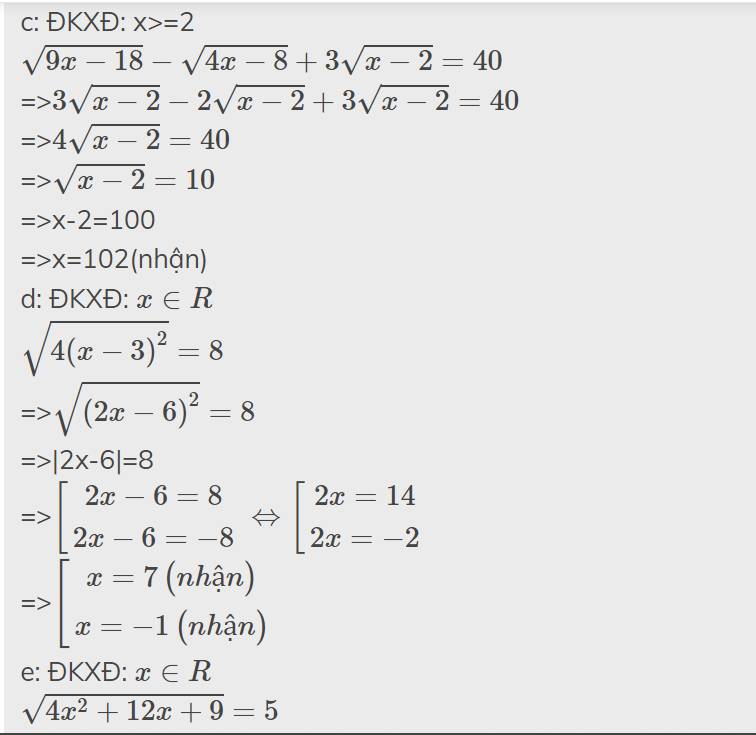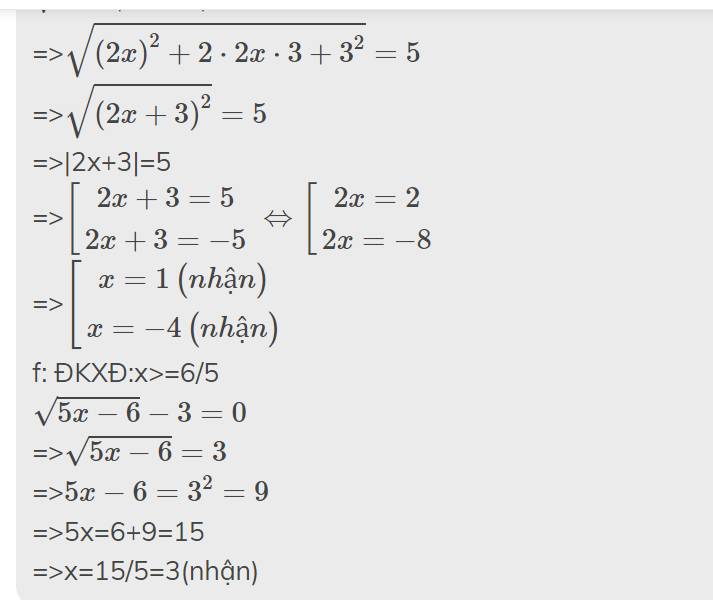giải phương trình x/(x+3)+6/(x-3)=-18/(9-x^2)
H24
Những câu hỏi liên quan
Giải phương trình
a) x-2 phần 4 + 2x-3 phần 3 =x-18 phần 6
b) x+3 phần x-3 + 3-x phần x+3 = 36 phần x2-9
a)
\(\dfrac{x-2}{4}+\dfrac{2x-3}{3}=\dfrac{x-18}{6}\)
`<=> 3x-6+8x-12=2x-36`
`<=> 3x+8x-2x=-36+6+12`
`<=> 9x=-18`
`<=> x=-2`
b)
\(\dfrac{x+3}{x-3}+\dfrac{3-x}{x+3}=\dfrac{36}{x^2-9}\left(x\ne3;x\ne-3\right)\)
suy ra
`(x+3)^2 +(3-x)(x-3)=36`
`<=>x^2 +6x+9+3x-9-x^2 +3x=36`
`<=> x^2 -x^2 +6x+3x+3x+9-9-36=0`
`<=> 12x-36=0`
`<=> 12x=36`
`<=> x=3 (KTMĐK)
Đúng 1
Bình luận (0)
Giải Phương Trình
\(\sqrt{\left(2x+3\right)^2}=5\)
\(\sqrt{9\left(x-2\right)^2}=18\)
\(\sqrt{9x-18}-\sqrt{4x-8}+3\sqrt{x-2}=40\)
\(\sqrt{4.\left(x-3\right)^2}=8\)
\(\sqrt{5x-6}-3=0\)
giải phương trình : (x\(^2\)-2x+3)(2x-x\(^2\)+6)=18
Đặt \(x^2-2x+3=t\Rightarrow2x-x^2+6=9-t\)
Pt trở thành:
\(t\left(9-t\right)=18\Leftrightarrow t^2-9t+18=0\)
\(\Rightarrow\left[{}\begin{matrix}t=3\\t=6\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x^2-2x+3=3\\x^2-2x+3=6\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-2x=0\\x^2-2x-3=0\end{matrix}\right.\) (bấm máy)
Đúng 1
Bình luận (0)
giải phương trình 27*x^3 +18*x^2-9*x+(27*x^2+2*x-1)cawn2x-1 -125=0
Giải phương trình sau : \(x^2-x-18+\left(2x+9\right)\sqrt{x+3}-2\sqrt{5x-1}=0\)
Giải phương trình sau
3/x^2+5x+4+2/x^2+10x+24+4/3+9/x^2+3x-18
Sửa đề:\(\frac{3}{x^2+5x+4}+\frac{2}{x^2+10x+24}=\frac{4}{3}=\frac{9}{x^2+3x-18}\)
\(\Leftrightarrow\frac{3}{\left(x+1\right)\left(x+4\right)}+\frac{2}{\left(x+4\right)\left(x+6\right)}=\frac{9}{\left(x-3\right)\left(x+6\right)}=\frac{4}{3}\)
\(\Leftrightarrow\frac{1}{x+1}-\frac{1}{x+4}+\frac{1}{x+4}-\frac{1}{x+6}=\frac{1}{x-3}-\frac{1}{x+6}=\frac{4}{3}\)
\(\Leftrightarrow\frac{1}{x+1}-\frac{1}{x-6}=\frac{1}{x-3}-\frac{1}{x+6}=\frac{4}{3}\)
\(\Leftrightarrow\frac{1}{x+1}-\frac{1}{x+6}-\frac{1}{x-3}+\frac{1}{x+6}=\frac{4}{3}\)
\(\Leftrightarrow\frac{1}{x+1}-\frac{1}{x+3}=\frac{4}{3}\)
Tự giải tiếp
Quyên sai rồi, tử là 1 mới đc tách kiểu đó, mà 2 pt đó bằng 4/3 thì xét 1 pt thôi được rồi, bước 3 từ dưới lên sai bét
Câu 2: Giải các phương trình sau:
a. \(\sqrt{4x-8}\) - \(\sqrt{x-2}\) - 4 + \(\dfrac{1}{3}\)\(\sqrt{9x-18}\)
b. \(\sqrt{x^2-6x+9}\) - \(\dfrac{\sqrt{6+\sqrt{3}}}{\sqrt{2}+1}\)=0
b: Ta có: \(\sqrt{x^2-6x+9}-\dfrac{\sqrt{6}+\sqrt{3}}{\sqrt{2}+1}=0\)
\(\Leftrightarrow x^2-6x+9=3\)
\(\Leftrightarrow x^2-6x+6=0\)
\(\text{Δ}=\left(-6\right)^2-4\cdot1\cdot6=36-24=12\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{6-2\sqrt{3}}{2}=3-\sqrt{3}\\x_2=3+\sqrt{3}\end{matrix}\right.\)
Đúng 0
Bình luận (0)
giải phương trình
\(\dfrac{2\sqrt{x}+5}{18}\)=2+\(\dfrac{\sqrt{x}-3}{6}\)
Đk:\(x\ge0\)
Pt \(\Leftrightarrow2\sqrt{x}+5=36+3\left(\sqrt{x}-3\right)\)
\(\Leftrightarrow-\sqrt{x}=22\) (vô nghiệm)
Vậy phương trình vô nghiệm
Đúng 2
Bình luận (0)
giải phương trình:\(\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{4x+15}{9-x^2}\)
giải bất phương trình: 2x+3<6-(3-4x)
1) \(\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{4x+15}{9-x^2}\)
ĐKXĐ : \(x\ne\pm3\)
\(\Leftrightarrow\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{-4x-15}{x^2-9}\)
\(\Leftrightarrow\frac{\left(x-1\right)\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}-\frac{x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3}{\left(x-3\right)\left(x+3\right)}-\frac{x^2+3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3-x^2-3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow-7x+3=-4x-15\)
\(\Leftrightarrow-7x+4x=-15-3\)
\(\Leftrightarrow-3x=-18\)
\(\Leftrightarrow x=6\)( tmđk )
Vậy x = 6 là nghiệm của phương trình
2) 2x + 3 < 6 - ( 3 - 4x )
<=> 2x + 3 < 6 - 3 + 4x
<=> 2x - 4x < 6 - 3 - 3
<=> -2x < 0
<=> x > 0
Vậy nghiệm của bất phương trình là x > 0