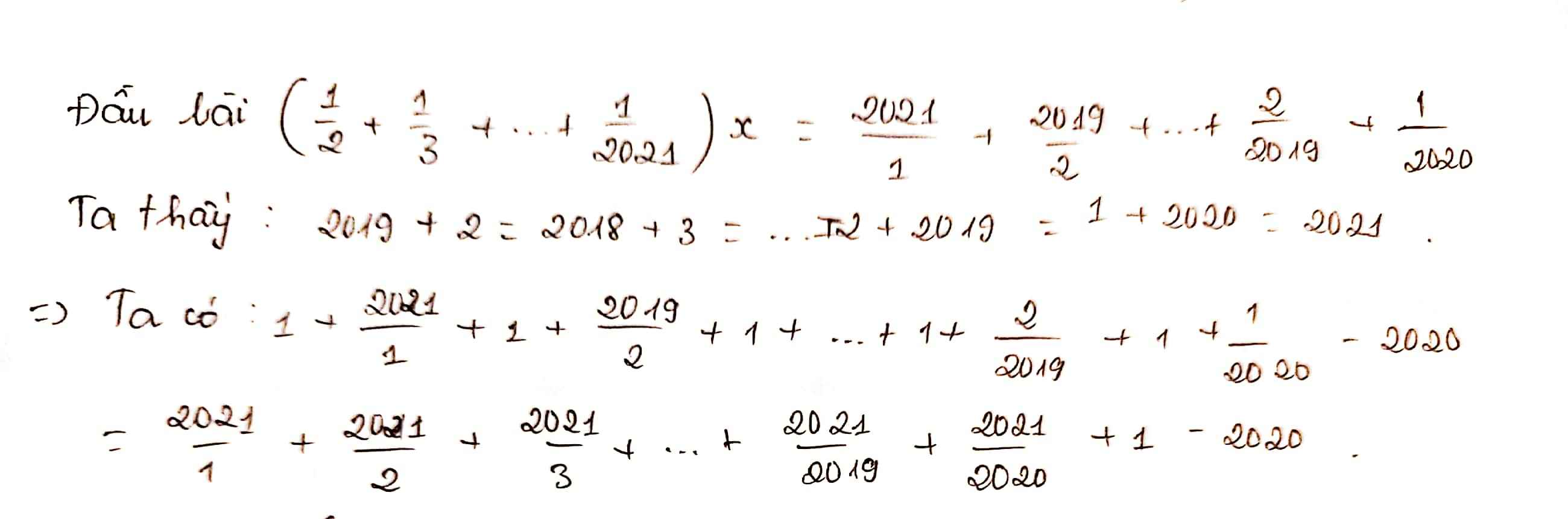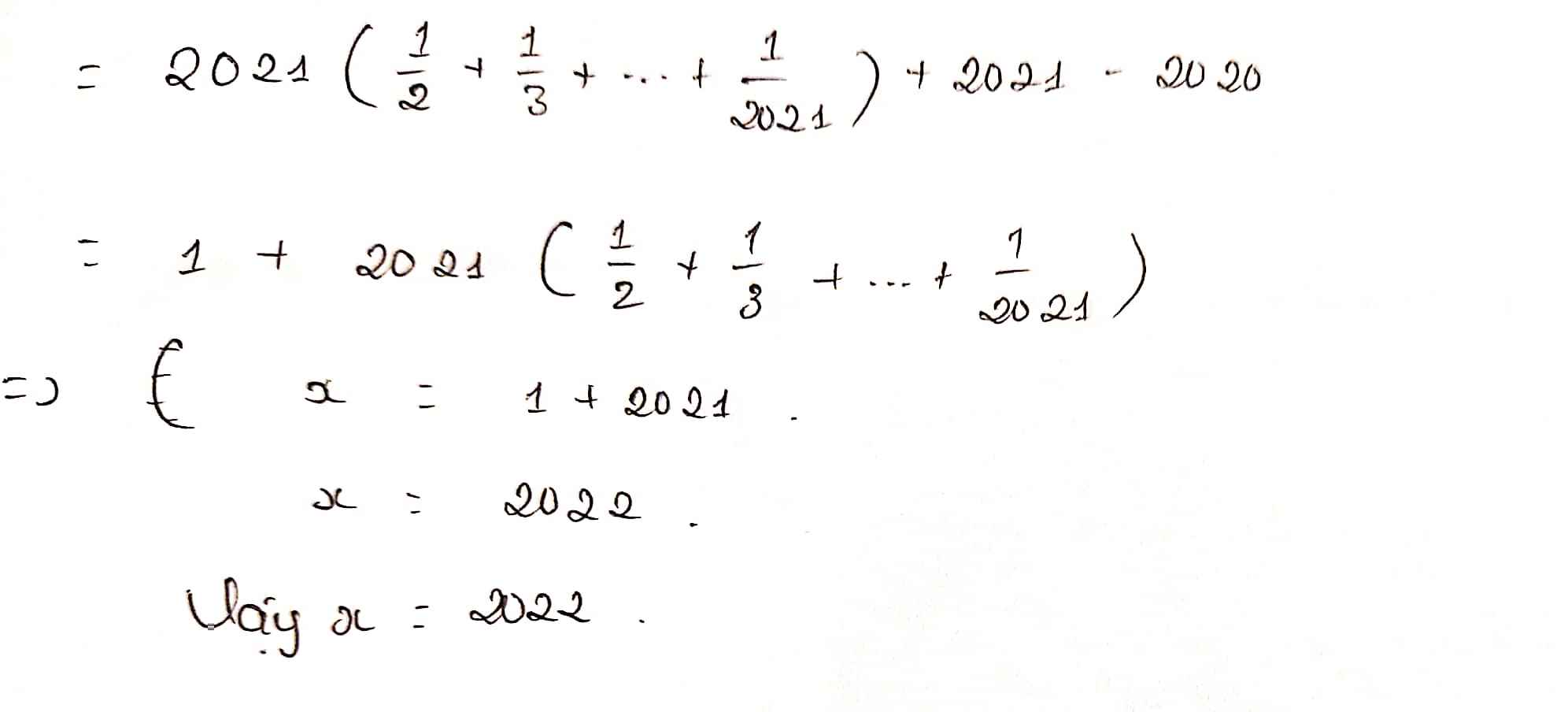(x+1)/2021+(x+2)/2020+(x+3)/2019+(x+2028)/2=0
H24
Những câu hỏi liên quan
Giải phương trình x+1/2021+x+2/2020+x+3/2019+x+2028/2=0
=>\(\left(\dfrac{x+1}{2021}+1\right)+\left(\dfrac{x+2}{2020}+1\right)+\left(\dfrac{x+3}{2019}+1\right)+\left(\dfrac{x+2028}{2}-3\right)=0\)
=>x+2022=0
=>x=-2022
Đúng 0
Bình luận (0)
Bài1:(1,5 điểm)Giải các phương trình sau a)3(2x-3)5x+1b)x+1/2021+x+2/2020+x+3/2019+x+2028/20
Đọc tiếp
Bài1:(1,5 điểm)Giải các phương trình sau
a)3(2x-3)=5x+1
b)x+1/2021+x+2/2020+x+3/2019+x+2028/2=0
a) \(3\left(2x-x\right)=5x+1\)
\(\Leftrightarrow6x-3x=5x+1\)
\(\Leftrightarrow6x-3x-5x=1\)
\(\Leftrightarrow-2x=1\)
\(\Leftrightarrow x=\dfrac{1}{-2}=-\dfrac{1}{2}\)
b) \(\dfrac{x+1}{2021}+\dfrac{x+2}{2020}+\dfrac{x+3}{2019}+\dfrac{x+4}{2018}=0\)
\(\Leftrightarrow\dfrac{x+1}{2021}+1+\dfrac{x+2}{2020}+1=\dfrac{x+3}{2019}+1+\dfrac{x+4}{2018}+1\)
\(\Leftrightarrow\dfrac{x+2022}{2021}+\dfrac{x+2022}{2020}=\dfrac{x+2022}{2019}+\dfrac{x+2022}{2018}\)
\(\Leftrightarrow\left(x+2022\right)\left(\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}+\dfrac{1}{2018}\right)\)
\(\Leftrightarrow x+2022=0\)
\(\Leftrightarrow x=-2022\)
Đúng 1
Bình luận (1)
a)3(2x-3)=5x+1
⇔6x-9=5x+1
⇔6x-5x=1+9
⇔x=10
vậy phương trình có nghiệm là S={10}
b)\(\dfrac{x+1}{2021}\)+\(\dfrac{x+2}{2020}\)+\(\dfrac{x+3}{2019}\)+\(\dfrac{x+2028}{2}\)=0
⇔2020(x+1)+2021(x+2)+2041210(x+2028)=0
⇔2045251x+4139579942=0
⇔2045251x=-4139579942=0
⇔x=-\(\dfrac{4139579942}{2045251}\)
vậy phương trình có tập nghiệm là S={\(-\dfrac{4139579942}{2045251}\)}
Đúng 2
Bình luận (0)
Cho hàm số f(x)= x +1/4 Tính tổng f(0)+f(1/2021)+f(2/2021)+f(3/2021)+...+f(2019/2021)+f(2020/2021)+f(1)
Tìm x biết:
( 1/2 + 1/3 + ... + 1/2021 ).x = 2021/1 +2019/2 + ... + 2/2019 + 1/2020
Tìm x biết:
( 1/2 + 1/3 + ... + 1/2021 ).x = 2021/1 +2019/2 + ... + 2/2019 + 1/2020
Nếu 1/3 + 1/6 +1/10 + ...... + 1/x.(x+1) : 2 = 2019/2021
A.x = 2019/2020 B. x = 2019 C. x = 2020 D. x = 2021
Xem thêm câu trả lời
Giải phương trình
\(\dfrac{1-\sqrt{x-2019}}{x-2019}+\dfrac{1-\sqrt{y-2020}}{y-2020}+\dfrac{1-\sqrt{z-2021}}{z-2021}+\dfrac{3}{4}=0\)
ĐKXĐ : \(\left\{{}\begin{matrix}x>2019\\y>2020\\z>2021\end{matrix}\right.\)
Đặt \(\sqrt{x-2019}=a,......\)
Ta được PT : \(\dfrac{1-a}{a^2}+\dfrac{1-b}{b^2}+\dfrac{1-c}{c^2}+\dfrac{3}{4}=0\)
\(\Leftrightarrow\dfrac{1}{a^2}-\dfrac{1}{a}+\dfrac{1}{4}+\dfrac{1}{b^2}-\dfrac{1}{b}+\dfrac{1}{4}+\dfrac{1}{c^2}-\dfrac{1}{c}+\dfrac{1}{4}=0\)
\(\Leftrightarrow\left(\dfrac{1}{a}-\dfrac{1}{2}\right)^2+\left(\dfrac{1}{b}-\dfrac{1}{2}\right)^2+\left(\dfrac{1}{c}-\dfrac{1}{2}\right)^2=0\)
- Thấy : \(\left(\dfrac{1}{a}-\dfrac{1}{2}\right)^2\ge0,......\)
\(\Rightarrow\left(\dfrac{1}{a}-\dfrac{1}{2}\right)^2+\left(\dfrac{1}{b}-\dfrac{1}{2}\right)^2+\left(\dfrac{1}{c}-\dfrac{1}{2}\right)^2\ge0\)
- Dấu " = " xảy ra <=> \(\left\{{}\begin{matrix}\dfrac{1}{a}=\dfrac{1}{2}\\\dfrac{1}{b}=\dfrac{1}{2}\\\dfrac{1}{c}=\dfrac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}a=2\\b=2\\c=2\end{matrix}\right.\)
- Thay lại a. b. c ta được : \(\left\{{}\begin{matrix}\sqrt{x-2019}=2\\\sqrt{y-2020}=2\\\sqrt{z-2021}=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-2019=4\\y-2020=4\\z-2021=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=2023\\y=2024\\z=2025\end{matrix}\right.\) ( TM )
Vậy ...
Đúng 1
Bình luận (0)
a, \(\left(2x-1\right)\left(x+\dfrac{2}{3}\right)=0\)
b, \(\dfrac{x+4}{2019}+\dfrac{x+3}{2020}=\dfrac{x+2}{2021}+\dfrac{x+1}{2022}\)
a)
`(2x-1)(x+2/3)=0`
\(< =>\left[{}\begin{matrix}2x-1=0\\x+\dfrac{2}{3}=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-\dfrac{2}{3}\end{matrix}\right.\)
b)
\(\dfrac{x+4}{2019}+\dfrac{x+3}{2020}=\dfrac{x+2}{2021}+\dfrac{x+1}{2022}\)
\(< =>\dfrac{x+4}{2019}+1+\dfrac{x+3}{2020}+1=\dfrac{x+2}{2021}+1+\dfrac{x+1}{2022}+1\)
\(< =>\dfrac{x+2023}{2019}+\dfrac{x+2023}{2020}=\dfrac{x+2023}{2021}+\dfrac{x+2023}{2022}\)
\(< =>\left(x+2023\right)\left(\dfrac{1}{2019}+\dfrac{1}{2020}-\dfrac{1}{2021}-\dfrac{1}{2022}\right)=0\)
\(< =>x+2023=0\left(\dfrac{1}{2019}+\dfrac{1}{2020}-\dfrac{1}{2021}-\dfrac{1}{2022}\ne0\right)\\ < =>x=-2023\)
Đúng 3
Bình luận (0)
sai rồi , x không thể có 2 giá trị
Đúng 0
Bình luận (0)
a) + Chia thành 2 trường hợp
- 2x - 1 = 0
2x = 0 + 1
2x = 1
x = 1 : 2
x = 0,5
- x + 2/3 = 0
x = 0 - 2/3
x = -2/3
vậy x = { 0,5 ; -2/3 }
Đúng 1
Bình luận (0)
Xem thêm câu trả lời
Cho x1 + x2 + x3 + ... + x2021 = 0 và x1 + x2 = x3 + x4 = ... = x2019 + x2020 = 2. Tìm x2021.
\(x_1+x_2=x_3+x_4=...=x_{2019}+x_{2020}=2\Rightarrow x_1+x_2+x_3+x_4+...+x_{2019}+x_{2020}=2.1010=2020\)
\(\Rightarrow x_1+x_2+x_3+x_4+...+x_{2019}+x_{2020}+x_{2021}=2020+x_{2021}\)
\(\Rightarrow0=2020+x_{2021}\)
\(\Rightarrow x_{2021}=-2020\)
Vậy \(x_{2021}=-2020\)