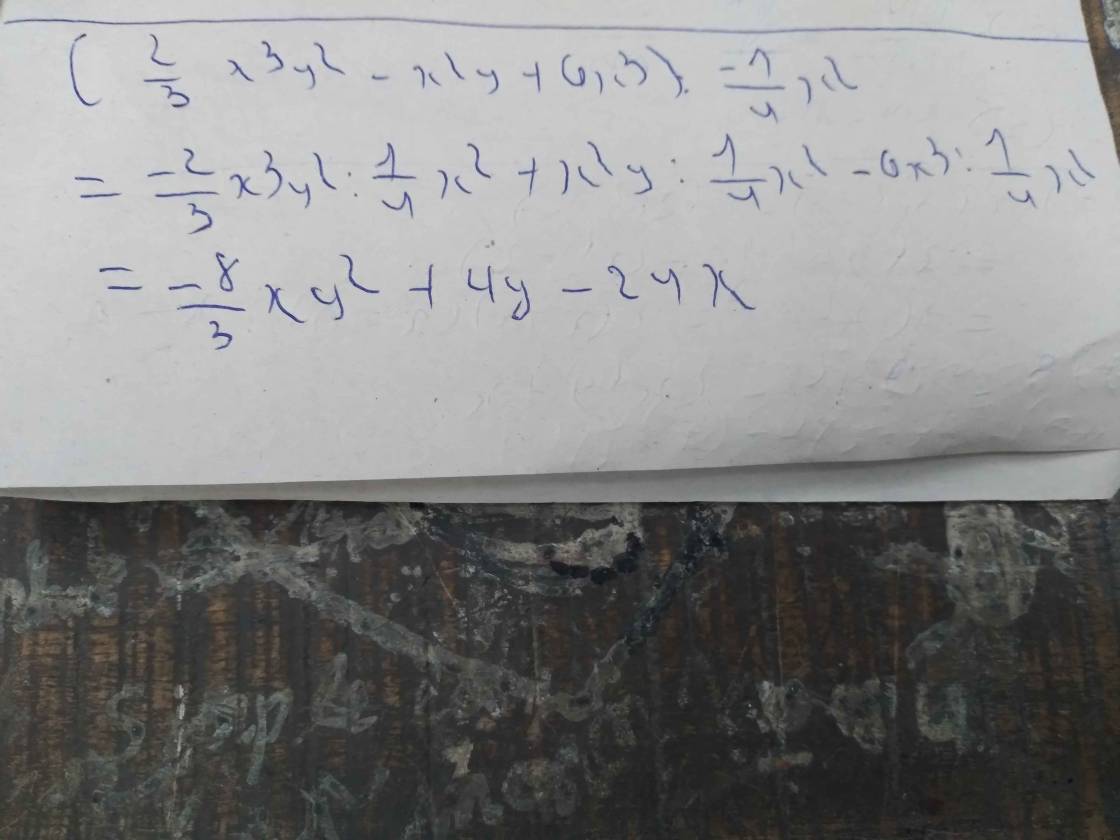Thực hiện phép tính \(\dfrac{x-1}{x-y}+\dfrac{1-y}{x-y}\)
DN
Những câu hỏi liên quan
Thực hiện các phép tính sau:
a,(\(\dfrac{x}{x+1}\)+\(\dfrac{x-1}{x}\)):(\(\dfrac{x}{x+1}\)-\(\dfrac{x-1}{x}\))
b,(1+\(\dfrac{x}{y}\)+\(\dfrac{x^2}{y^2}\)).(1-\(\dfrac{x}{y}\)).\(\dfrac{y^2}{x^3-y^3}\)
\(\left(\dfrac{x}{x+1}+\dfrac{x-1}{x}\right):\left(\dfrac{x}{x+1}-\dfrac{x-1}{x}\right)\) \(\left(đk:x\ne0;-1\right)\)
\(=\dfrac{x^2+\left(x-1\right)\left(x+1\right)}{x\left(x+1\right)}:\left(\dfrac{x^2-\left(x-1\right)\left(x+1\right)}{x\left(x+1\right)}\right)\)
\(=\dfrac{x^2+x^2-1}{x\left(x+1\right)}.\dfrac{x\left(x+1\right)}{x^2-x^2+1}\)
\(=\dfrac{\left(2x^2-1\right)x\left(x+1\right)}{x\left(x+1\right)}=2x^2-1\)
Đúng 1
Bình luận (0)
thực hiện phép tính sau\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
MTC = (x - y)(x2 + xy + y2)
\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)
Đúng 1
Bình luận (0)
1/x-y-3xy/x^3-y^3+x-y/x^2+xy+y^2
=1/x-y+-3xy/(x-y)(x^2+xy+y^2)+x-y/x^2+xy+y^2
=x^2+xy+y^2/(x-y)(x^2+xy+y^2)+-3xy/(x-y)(x^2+xy+y^2)+x^2-2xy+y^2/(x-y)(x^2+xy+y^2)
=x^2+xy+y^2-3xy+x^2-2xy-y^2/(x-y)(x^2+xy+y^2)
=2x^2-5xy/(x-y)(x^2+xy+y^2)
Đúng 0
Bình luận (0)
MTC = (x - y)(x2 + xy + y2)
\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)
Đúng 0
Bình luận (0)
Thực hiện các phép tính sau:
a) \(\dfrac{{x + 2}}{{x - 1}} - \dfrac{{x - 3}}{{x - 1}} - \dfrac{{x - 4}}{{1 - x}}\)
b) \(\dfrac{1}{{x + 5}} - \dfrac{1}{{x - 5}} + \dfrac{{2x}}{{{x^2} - 25}}\)
c) \(x + \dfrac{{2{y^2}}}{{x + y}} - y\)
\(a,\dfrac{x+2}{x-1}-\dfrac{x-3}{x-1}-\dfrac{x-4}{1-x}\\ =\dfrac{x+2}{x-1}-\dfrac{x-3}{x-1}+\dfrac{x-4}{x-1}\\ =\dfrac{x+2-x+3+x-4}{x-1}\\ =\dfrac{x+1}{x-1}\)
\(b,\dfrac{1}{x+5}-\dfrac{1}{x-5}+\dfrac{2x}{x^2-25}\\ =\dfrac{1}{x+5}-\dfrac{1}{x-5}+\dfrac{2x}{\left(x-5\right)\left(x+5\right)}\\ =\dfrac{x-5-x-5+2x}{\left(x-5\right)\left(x+5\right)}\\ =\dfrac{2x-10}{\left(x-5\right)\left(x+5\right)}\\ =\dfrac{2\left(x-5\right)}{\left(x-5\right)\left(x+5\right)}\\ =\dfrac{2}{x+5}\)
\(c,x+\dfrac{2y^2}{x+y}-y\\ =\dfrac{x\left(x+y\right)+2y^2-y\left(x+y\right)}{x+y}\\ =\dfrac{x^2+xy+2y^2-xy-y^2}{x+y}\\ =\dfrac{x^2+y^2}{x+y}\)
Đúng 2
Bình luận (0)
Thực hiện các phép tính sau:a) dfrac{{2{x^2} - 1}}{{x - 2}} + dfrac{{ - {x^2} - 3}}{{x - 2}} b) dfrac{x}{{x + y}} + dfrac{y}{{x - y}} c) dfrac{1}{{x - 1}} - dfrac{2}{{{x^2} - 1}}d) dfrac{{x + 2}}{{{x^2} + xy}} - dfrac{{y - 2}}{{xy + {y^2}}} e) dfrac{1}{{2{x^2} - 3x}} - dfrac{1}{{4{x^2} - 9}} g) dfrac{{2x}}{{9 - {x^2}}} + dfrac{1}{{x - 3}} - dfrac{1}{{x + 3}}
Đọc tiếp
Thực hiện các phép tính sau:
a) \(\dfrac{{2{x^2} - 1}}{{x - 2}} + \dfrac{{ - {x^2} - 3}}{{x - 2}}\)
b) \(\dfrac{x}{{x + y}} + \dfrac{y}{{x - y}}\)
c) \(\dfrac{1}{{x - 1}} - \dfrac{2}{{{x^2} - 1}}\)
d) \(\dfrac{{x + 2}}{{{x^2} + xy}} - \dfrac{{y - 2}}{{xy + {y^2}}}\)
e) \(\dfrac{1}{{2{x^2} - 3x}} - \dfrac{1}{{4{x^2} - 9}}\)
g) \(\dfrac{{2x}}{{9 - {x^2}}} + \dfrac{1}{{x - 3}} - \dfrac{1}{{x + 3}}\)
a: \(=\dfrac{2x^2-1-x^2-3}{x-2}=\dfrac{x^2-4}{x-2}=x+2\)
b: \(=\dfrac{x\left(x-y\right)+y\left(x+y\right)}{\left(x+y\right)\left(x-y\right)}\)
\(=\dfrac{x^2-xy+xy+y^2}{x^2-y^2}=\dfrac{x^2+y^2}{x^2-y^2}\)
c: \(=\dfrac{x+1-2}{\left(x-1\right)\left(x+1\right)}=\dfrac{\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{1}{x+1}\)
d: \(=\dfrac{\left(x+2\right)\cdot y-x\left(y-2\right)}{xy\left(x+y\right)}\)
\(=\dfrac{2y+2x}{xy\left(x+y\right)}=\dfrac{2}{xy}\)
e: \(=\dfrac{1}{x\left(2x-3\right)}-\dfrac{1}{\left(2x-3\right)\left(2x+3\right)}\)
\(=\dfrac{2x+3-x}{x\left(2x-3\right)\left(2x+3\right)}=\dfrac{x+3}{x\left(2x-3\right)\left(2x+3\right)}\)
g: \(=\dfrac{-2x+x+3-x+3}{\left(x-3\right)\left(x+3\right)}=\dfrac{-2x+6}{\left(x-3\right)\left(x+3\right)}=\dfrac{-2}{x+3}\)
Đúng 1
Bình luận (0)
Thực hiện phép tính cộng
\(\dfrac{1}{x-y}\)+\(\dfrac{3xy}{y^3-x^3}\)+\(\dfrac{x-y}{x^2+xy+y^2}\)
GIÚP MÌNH VS Ạ
Ta có:
\(\dfrac{1}{x-y}+\dfrac{3xy}{y^3-x^3}+\dfrac{x-y}{x^2+xy+y^2}\\ =\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{x^3-y^3}\\ =\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\\ =\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)
Đúng 3
Bình luận (0)
\(\dfrac{1}{x-y}+\dfrac{3xy}{y^3-x^3}+\dfrac{x-y}{x^2+xy+y^2}\) \(=\dfrac{x^2+xy+y^2}{x^3-y^3}-\dfrac{3xy}{x^3-y^3}+\dfrac{\left(x-y\right)^2}{x^3-y^3}\)
\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{x^3-y^3}\)
\(=\dfrac{2x^2+2y^2-4xy}{x^3-y^3}\)
\(=\dfrac{2x^2-2xy-2xy+2y^2}{x^3-y^3}\)
\(=\dfrac{2x\left(x-y\right)-2y\left(x-y\right)}{x^3-y^3}\)
\(=\dfrac{\left(2x-2y\right)\left(x-y\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2x-2y}{x^2+xy+y^2}\)
Đúng 1
Bình luận (0)
Tìm mẫu thức chung của các phân thức:
\(\dfrac{1}{x^2+x}\) ; \(\dfrac{x^2-4}{x^2-1}\)
Thực hiện phép tính:
\(\dfrac{1}{y-1}\) - \(\dfrac{1}{y}\)
\(\dfrac{1}{x^2+x}=\dfrac{x-1}{x\left(x-1\right)\left(x+1\right)};\dfrac{x^2-4}{x^2-1}=\dfrac{x\left(x^2-4\right)}{x\left(x-1\right)\left(x+1\right)}\\ \dfrac{1}{y-1}-\dfrac{1}{y}=\dfrac{y-y+1}{y\left(y-1\right)}=\dfrac{1}{y\left(y-1\right)}\)
Đúng 1
Bình luận (0)
1) Thực hiện các phép tính sau ( giả thiết các phân thức đã cho có nghĩa).a)dfrac{x^3}{x-1}-dfrac{x^2}{x+1}-dfrac{1}{x-1}+dfrac{1}{x+1}b)dfrac{x+y}{2.left(x-yright)}-dfrac{x-y}{2.left(x+yright)}+dfrac{2y^2}{x^2-y^2}c)dfrac{x+5}{2x-4}.dfrac{4-2x}{x+2}d) dfrac{8}{x^2+2x-3}+dfrac{2}{x+3}+dfrac{1}{x-1}Mình đang cần gấp ah
Đọc tiếp
1) Thực hiện các phép tính sau ( giả thiết các phân thức đã cho có nghĩa).
a)\(\dfrac{x^3}{x-1}\)-\(\dfrac{x^2}{x+1}\)-\(\dfrac{1}{x-1}\)+\(\dfrac{1}{x+1}\)
b)\(\dfrac{x+y}{2.\left(x-y\right)}\)-\(\dfrac{x-y}{2.\left(x+y\right)}\)+\(\dfrac{2y^2}{x^2-y^2}\)
c)\(\dfrac{x+5}{2x-4}\).\(\dfrac{4-2x}{x+2}\)
d) \(\dfrac{8}{x^2+2x-3}\)+\(\dfrac{2}{x+3}\)+\(\dfrac{1}{x-1}\)
Mình đang cần gấp ah
a.
\(\dfrac{x^3}{x-1}-\dfrac{x^2}{x+1}-\dfrac{1}{x-1}+\dfrac{1}{x+1}=\dfrac{x^3-1}{x-1}-\dfrac{x^2-1}{x+1}\)
\(=\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{x-1}-\dfrac{\left(x-1\right)\left(x+1\right)}{x+1}\)
\(=x^2+x+1-\left(x-1\right)=x^2+2\)
b.
\(\dfrac{x+y}{2\left(x-y\right)}-\dfrac{x-y}{2\left(x+y\right)}+\dfrac{2y^2}{x^2-y^2}\)
\(=\dfrac{\left(x+y\right)^2}{2\left(x-y\right)\left(x+y\right)}-\dfrac{\left(x-y\right)^2}{2\left(x-y\right)\left(x+y\right)}+\dfrac{4y^2}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{\left(x+y\right)^2-\left(x-y\right)^2+4y^2}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{4xy+4y^2}{2\left(x-y\right)\left(x+y\right)}=\dfrac{4y\left(x+y\right)}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{2y}{x-y}\)
c.
\(\dfrac{x+5}{2x-4}.\dfrac{4-2x}{x+2}=\dfrac{x+5}{2x-4}.\dfrac{-\left(2x-4\right)}{x+2}=-\dfrac{x+5}{x+2}\)
d.
\(\dfrac{8}{x^2+2x-3}+\dfrac{2}{x+3}+\dfrac{1}{x-1}=\dfrac{8}{\left(x-1\right)\left(x+3\right)}+\dfrac{2\left(x-1\right)}{\left(x-1\right)\left(x+3\right)}+\dfrac{x+3}{\left(x-1\right)\left(x+3\right)}\)
\(=\dfrac{8+2\left(x-1\right)+x+3}{\left(x-1\right)\left(x+3\right)}=\dfrac{3x+9}{\left(x-1\right)\left(x+3\right)}\)
\(=\dfrac{3\left(x+3\right)}{\left(x-1\right)\left(x+3\right)}=\dfrac{3}{x-1}\)
Đúng 2
Bình luận (0)
Thực hiện phép tính , rút gọn bt
\(\dfrac{2x+y}{2x^2-xy}+\dfrac{16x}{y^2-4x^2}+\dfrac{2x-y}{2x^2+xy}\)
\(\dfrac{x+y}{2\left(x-y\right)}+\dfrac{2}{x^2+3}+\dfrac{1}{x+1}\)
1, Thực hiện phép tính
(\(\dfrac{2}{3}\)x\(^3\)y\(^2\)-x\(^2\)y+6x\(^3\)) : (\(\dfrac{-1}{4}\)x\(^2\))