giải phương trình:
cos2x + cos4x + cos6x = cosx.cos2x.cos3x + 2
CT
Những câu hỏi liên quan
Giải phương trình : cos4x + cos6x = sin7x - sin3x
Xem chi tiết
\(\Leftrightarrow2cos5x.cosx=2cos5x.sin2x\)
\(\Leftrightarrow\left[{}\begin{matrix}cos5x=0\\cosx=sin2x=cos\left(\frac{\pi}{2}-2x\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=\frac{\pi}{2}+k\pi\\x=\frac{\pi}{2}-2x+k2\pi\\x=2x-\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{10}+\frac{k\pi}{5}\\x=\frac{\pi}{6}+\frac{k2\pi}{3}\\x=\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
Sin7x/sinx=sinx+2(cos2x+cos4x+cos6x)
ĐKXĐ: \(x\ne k\pi\)
\(sin7x=sin^2x+2sinx.cos2x+2sinx.cos4x+2sinx.cos6x\)
\(\Leftrightarrow sin7x=sin^2x+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)
\(\Leftrightarrow sin7x=sin^2x-sinx+sin7x\)
\(\Leftrightarrow sinx\left(sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\left(loại\right)\\sinx=1\end{matrix}\right.\)
\(\Rightarrow x=\dfrac{\pi}{2}+k2\pi\)
Đúng 1
Bình luận (0)
2-cos8x-cos6x+cos4x-cos2x=0
cos2x + cos4x + cos6x = 0
1 + cosx + cos2x + cos3x = 0
sin3x - sinx = cos3x - cosx
a. cos2x + cos4x + cos6x = 0
\(\Leftrightarrow\left(cos2x+cos6x\right)+cos4x=0\\ \Leftrightarrow2cos4x.cos2x+cos4x=0\\ \Leftrightarrow cos4x\left(2cos2x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}cos4x=0\\cos2x=\dfrac{-1}{2}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\left(k\in Z\right)}\)
Đúng 0
Bình luận (0)
1.
\(cos2x+cos6x+cos4x=0\)
\(\Leftrightarrow2cos4x.cos2x+cos4x=0\)
\(\Leftrightarrow cos4x\left(2cos2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos4x=0\\cos2x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=\dfrac{\pi}{2}+k\pi\\2x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
2.
\(\Leftrightarrow1+cos2x+cosx+cos3x=0\)
\(\Leftrightarrow1+2cos^2x-1+2cos2x.cosx=0\)
\(\Leftrightarrow cos^2x+cos2x.cosx=0\)
\(\Leftrightarrow cosx\left(cos2x+cosx\right)=0\)
\(\Leftrightarrow cosx\left(2cos^2x+cosx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=-1\\cosx=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k\pi\\x=\pi+k2\pi\\x=\pm\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Giải các phương trình sau: sin6x + cos6x
15
8
cos2x –
1
2
A. x ±
π
6
+ k2π, k ∈ Z B. x ±
5
π
6
+ k2π, k ∈ Z C. x
-
π
4
+ k2π, x
-
π
6
+ k2π, k ∈ Z D. Cả A và B đún...
Đọc tiếp
Giải các phương trình sau: sin6x + cos6x = 15 8 cos2x – 1 2
A. x = ± π 6 + k2π, k ∈ Z
B. x = ± 5 π 6 + k2π, k ∈ Z
C. x = - π 4 + k2π, x = - π 6 + k2π, k ∈ Z
D. Cả A và B đúng
Giải các phương trình sau: sin 2 x - cos 2 x = cos 4 x
sin 2 x - cos 2 x = cos 4 x ⇔ - cos 2 x = cos 4 x ⇔ 2 cos 3 x . cos x = 0
Đúng 0
Bình luận (0)
sin
6
x
+
cos
6
x
cos
4
x
phương trình nào sau đây tương đương với phương trình vừa cho:
Đọc tiếp
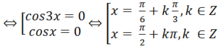
sin 6 x + cos 6 x = cos 4 x phương trình nào sau đây tương đương với phương trình vừa cho:
sin
6
x
+
cos
6
x
cos
4
x
phương trình nào sau đây tương đương với phương trình vừa cho: A.
cos
4
x
2
2
B.
cos
4
x
1
C.
cos
4
x
1
2
D.
cos
4...
Đọc tiếp
sin 6 x + cos 6 x = cos 4 x phương trình nào sau đây tương đương với phương trình vừa cho:
A. cos 4 x = 2 2
B. cos 4 x = 1
C. cos 4 x = 1 2
D. cos 4 x = 3 2
Đạo hàm của hàm số y cos6x + sin4x. cos2x + sin2x. cos4x + sin4x – sin2x bằng biểu thức nào sau đây? A.
-
6
cos
5
x
sin
x
B.
6
cos
5
x
sin
x
C.
6
sin
5
x
cos
x
D.
6
cos
5...
Đọc tiếp
Đạo hàm của hàm số y = cos6x + sin4x. cos2x + sin2x. cos4x + sin4x – sin2x bằng biểu thức nào sau đây?
A. - 6 cos 5 x sin x
B. 6 cos 5 x sin x
C. 6 sin 5 x cos x
D. 6 cos 5 x
Chọn A
y = cos6 x+ sin2xcos2x(sin2x + cos2x) + sin4x - sin2x
= cos6x + sin2x(1 - sin2x) + sin4x - sin2x = cos6x
Do đó : y' = -6cos5xsinx.
Đúng 0
Bình luận (0)