Giải phương trình
x2 bằng CBH( x3-x2) + CBH(x2-x)
Giải phương trình (x3 + x2) + (x2 + x) = 0.
(x3 + x2) + (x2 + x) = 0
⇔x2 (x + 1) + x(x + 1) = 0
⇔(x2 + x)(x + 1) = 0
⇔x(x + 1)(x + 1) = 0
⇔x = 0 hoặc x + 1 = 0
⇔x = 0 hoặc x = -1
Vậy tập nghiệm của phương trình là : S = {0; -1}
Giải phương trình bằng cách đưa về phương trình tích:
a ) 3 x 2 − 7 x − 10 ⋅ 2 x 2 + ( 1 − 5 ) x + 5 − 3 = 0 b ) x 3 + 3 x 2 − 2 x − 6 = 0 c ) x 2 − 1 ( 0 , 6 x + 1 ) = 0 , 6 x 2 + x d ) x 2 + 2 x − 5 2 = x 2 − x + 5 2
a) 3 x 2 − 7 x − 10 ⋅ 2 x 2 + ( 1 − 5 ) x + 5 − 3 = 0
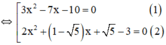
+ Giải (1):
3 x 2 – 7 x – 10 = 0
Có a = 3; b = -7; c = -10
⇒ a – b + c = 0
⇒ (1) có hai nghiệm x 1 = - 1 v à x 2 = - c / a = 10 / 3 .
+ Giải (2):
2 x 2 + ( 1 - √ 5 ) x + √ 5 - 3 = 0
Có a = 2; b = 1 - √5; c = √5 - 3
⇒ a + b + c = 0
⇒ (2) có hai nghiệm:
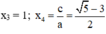
Vậy phương trình có tập nghiệm 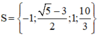
b)
x 3 + 3 x 2 - 2 x - 6 = 0 ⇔ x 3 + 3 x 2 - ( 2 x + 6 ) = 0 ⇔ x 2 ( x + 3 ) - 2 ( x + 3 ) = 0 ⇔ x 2 - 2 ( x + 3 ) = 0
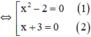
+ Giải (1): x 2 – 2 = 0 ⇔ x 2 = 2 ⇔ x = √2 hoặc x = -√2.
+ Giải (2): x + 3 = 0 ⇔ x = -3.
Vậy phương trình có tập nghiệm S = {-3; -√2; √2}
c)
x 2 − 1 ( 0 , 6 x + 1 ) = 0 , 6 x 2 + x ⇔ x 2 − 1 ( 0 , 6 x + 1 ) = x ⋅ ( 0 , 6 x + 1 ) ⇔ x 2 − 1 ( 0 , 6 x + 1 ) − x ( 0 , 6 x + 1 ) = 0 ⇔ ( 0 , 6 x + 1 ) x 2 − 1 − x = 0
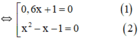
+ Giải (1): 0,6x + 1 = 0 ⇔ 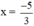
+ Giải (2):
x 2 – x – 1 = 0
Có a = 1; b = -1; c = -1
⇒ Δ = ( - 1 ) 2 – 4 . 1 . ( - 1 ) = 5 > 0
⇒ (2) có hai nghiệm 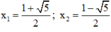
Vậy phương trình có tập nghiệm 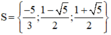
d)
x 2 + 2 x − 5 2 = x 2 − x + 5 2 ⇔ x 2 + 2 x − 5 2 − x 2 − x + 5 2 = 0 ⇔ x 2 + 2 x − 5 − x 2 − x + 5 ⋅ x 2 + 2 x − 5 + x 2 − x + 5 = 0 ⇔ ( 3 x − 10 ) 2 x 2 + x = 0
⇔ (3x-10).x.(2x+1)=0
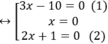
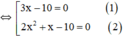
+ Giải (1): 3x – 10 = 0 ⇔ 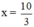
+ Giải (2):
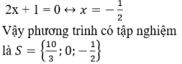
Giải các phương trình sau bằng cách đưa về phương trình tích x 3 – 5 x 2 –x +5 = 0
Ta có: x 3 – 5 x 2 –x +5 = 0 ⇔ x 2 ( x -5) – ( x -5) =0
⇔ (x -5)(x2 -1) =0 ⇔ (x -5)(x -1)(x +1) =0
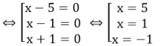
Vậy phương trình đã cho có 3 nghiệm :x1 = 5;x2 =1;x3=-1
Giải phương trình: 2x2 -1/ x3 +1 + 1/x+1 = 2x (1- x2 -x/ x2 -x +1 )
Không dich được đề bài, đề là:
\(\dfrac{2x^2-1}{x^3+1}+\dfrac{1}{x+1}=2x\left(\dfrac{1-x^2-x}{x^2-x+1}\right)\)
Hay: \(...=2\left(1-x^2-\dfrac{x}{x^2-x+1}\right)\)
Giải phương trình x 3 - x 2 = 1 - x
Giải phương trình: x3-x2+x=1/3
giải các phương trình sau:
a) x2+2x=(x-2)3x
b) x3+x2-x-1=0
c) (x+1)(x+2)(x+4)(x+5)=40
a) \(x^2+2x=\left(x-2\right).3x\)
\(\Leftrightarrow x^2+2x=3x^2-6x\)
\(\Leftrightarrow x^2+2x-3x^2+6x=0\)
\(\Leftrightarrow-2x^2+8x=0\)
\(\Leftrightarrow-2x\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}-2x=0\\x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
Vậy S = {0;4}
b) \(x^3+x^2-x-1=0\)
\(\Leftrightarrow x^2\left(x+1\right)-\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\x^2-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^2=1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\mp1\end{matrix}\right.\)
Vậy: S = {-1; 1}
c) \(\left(x+1\right)\left(x+2\right)\left(x+4\right)\left(x+5\right)=40\)
\(\Leftrightarrow\left[\left(x+1\right)\left(x+5\right)\right]\left[\left(x+2\right)\left(x+4\right)\right]=40\)
\(\Leftrightarrow\left(x^2+5x+x+5\right)\left(x^2+4x+2x+8\right)=40\)
\(\Leftrightarrow\left(x^2+6x+5\right)\left(x^2+6x+8\right)=40\)
Đặt x2 + 6x + 5 = t
\(\Leftrightarrow t.\left(t+3\right)=40\)
\(\Leftrightarrow t^2+3t=40\)
\(\Leftrightarrow t^2+2.t.\dfrac{3}{2}+\dfrac{9}{4}=\dfrac{169}{4}\)
\(\Leftrightarrow\left(t+\dfrac{3}{2}\right)^2=\dfrac{169}{4}\)
\(\Leftrightarrow\left[{}\begin{matrix}t+\dfrac{3}{2}=\dfrac{13}{2}\\t+\dfrac{3}{2}=-\dfrac{13}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}t=\dfrac{13}{2}-\dfrac{3}{2}=\dfrac{10}{2}=5\\t=-\dfrac{13}{2}-\dfrac{3}{2}=-\dfrac{16}{2}=-8\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2+6x+5=5\\x^2+6x+5=-8\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2+6x=0\\x^2+6x+13=0\end{matrix}\right.\)
Mà: \(x^2+6x+13=x^2+2.x.3+9+4=\left(x+3\right)^2+4\ne0\)
=> x2 + 6x = 0
<=> x. (x + 6) = 0
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+6=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-6\end{matrix}\right.\)
Vậy S = {0; -6}
a) Ta có: \(x^2+2x=\left(x-2\right)\cdot3x\)
\(\Leftrightarrow x\left(x+2\right)-3x\left(x-2\right)=0\)
\(\Leftrightarrow x\left[\left(x+2\right)-3\left(x-2\right)\right]=0\)
\(\Leftrightarrow x\left(x+2-3x+6\right)=0\)
\(\Leftrightarrow x\left(-2x+8\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\-2x+8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\-2x=-8\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
Vậy: S={0;4}
b) Ta có: \(x^3+x^2-x-1=0\)
\(\Leftrightarrow x^2\left(x+1\right)-\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\cdot\left(x^2-1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\cdot\left(x-1\right)\cdot\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)^2\cdot\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(x+1\right)^2=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x+1=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=1\end{matrix}\right.\)
Vậy: S={-1;1}
c) Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+4\right)\left(x+5\right)=40\)
\(\Leftrightarrow\left(x+1\right)\left(x+5\right)\left(x+2\right)\left(x+4\right)-40=0\)
\(\Leftrightarrow\left(x^2+6x+5\right)\left(x^2+6x+8\right)-40=0\)
\(\Leftrightarrow\left(x^2+6x\right)^2+13\left(x^2+6x\right)+40-40=0\)
\(\Leftrightarrow\left(x^2+6x\right)^2+13\left(x^2+6x\right)=0\)
\(\Leftrightarrow\left(x^2+6x\right)\left(x^2+6x+13\right)=0\)
\(\Leftrightarrow x\left(x+6\right)\left(x^2+6x+13\right)=0\)
mà \(x^2+6x+13>0\forall x\)
nên \(x\left(x+6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-6\end{matrix}\right.\)
Vậy: S={0;-6}
Giải phương trình: x − 2 − x 3 + x 2 = x − 4 3 − 1
A. -2 và 2
B. - 7 2 và -2
C. - 7 2 và 2
D. - 7 2 và 7 2
Đáp án C
x − 2 − x 3 + x 2 = x − 4 3 − 1 ⇔ 2 x − 2 − x 6 + 3 x 6 = 2 x − 4 6 − 6 6 ⇔ − 4 x − 2 x 2 + 3 x = 2 x − 8 − 6 ⇔ − 2 x 2 − 3 x + 14 = 0 t a c ó Δ = − 3 2 − 4. − 2 .14 = 121 ⇒ Δ = 11 ⇒ x 1 = 3 − 11 2. − 2 = 2 ; ⇒ x 2 = 3 + 11 2. − 2 = − 7 2
Cho phương trình 2 x 3 + 2 x 2 - 3x + 10 = 2 x 3 + x 2 – 10. Sau khi biến đổi đưa phương trình trên về dạng a x 2 + bx+ c =0 thì hệ số a bằng ?
A. 2
B. 1
C. 3
D. -1
Đáp án B
Ta có : 2 x 3 + 2 x 2 - 3 x + 10 = 2 x 3 + x 2 – 10
⇔ 2 x 3 + 2 x 2 - 3 x + 10 - 2 x 3 - x 2 + 10 = 0
⇔ x 2 – 3 x + 20 = 0
Phương trình trên là phương trình bậc hai một ẩn với a = 1; b = -3 và c = 20.
Giải phương trình: x 5 = x 4 + x 3 + x 2 + x + 2