Đề : phân tích các đa thức sau thành nhân tử
7x2 - 14xy
Phân tích đa thức thành nhân tử:
a) 3(x-5)-x2+5x
b) 7x2-14xy+7y2
c) (x2+y2)2-4x2y2
d) -25x2+y2+9-6y
\(a,=3\left(x-5\right)-x\left(x-5\right)=\left(3-x\right)\left(x-5\right)\\ b,=7\left(x^2-2xy+y^2\right)=7\left(x-y\right)^2\\ c,=\left(x^2+y^2-2xy\right)\left(x^2+y^2+2xy\right)=\left(x-y\right)^2\left(x+y\right)^2\\ d,=\left(y^2-6y+9\right)-25x^2=\left(y-3\right)^2-25x^2=\left(y-5x-3\right)\left(y+5x-3\right)\)
Bài 1. Phân tích đa thức bằng nhân tử.
a) 2x3 - 3x2
b) 3x4 - 24x
c) x3y + 5x2y
d) 7x2 + 14xy
a. \(2x^3-3x^2=x^2\left(2x-3\right)\)
b. \(3x^4-24x=3x\left(x^3-8\right)=3x\left(x-2\right)\left(x^2+2x+4\right)\)
c. \(x^3y+5x^2y=x^2y\left(x+5\right)\)
d. \(7x^2+14xy=7x\left(x+2y\right)\)
a)2x^3-3x^2=x^2(2x-3)
b)3x^4-24x
=3x(x^3-8x)
=3x(x-2)(x^2+2x+4)
c)x^3y+5x^2y
=x^2y(x+5)
d)7x^2+14xy
=7x(x+2y)
phân tích đa thức thành nhân tử
49x^2-1+14xy+y^2
= ( 7x2 + 14xy + y2 ) - 1
= ( 7x + y )2 - 1
= [(7x + y) + 1] [( 7x + y) - 1]
49.x^2 - 1 + 14xy + y^2
= (49.x^2 + 14xy + y^2) - 1
= (7x + y)^2 - 1
= (7x + y + 1)(7x + y - 1)
Phân tích đa thức thành nhân tử
a) 2x2y3 - 32y3
b) 7x2y - 14xy + 7y
c) 2x3 + 10x2y - xy - 5y2
a) \(=2y^3\left(x^2-16\right)=2y^3\left(x-4\right)\left(x+4\right)\)
b) \(=7y\left(x^2-2x+1\right)=7y\left(x-1\right)^2\)
c) \(=2x^2\left(x+5y\right)-y\left(x+5y\right)=\left(x+5y\right)\left(2x^2-y\right)\)
a: \(2x^2y^3-32y^3=2y^3\left(x-4\right)\left(x+4\right)\)
b: \(7x^2y-14xy+7y=7y\left(x^2-2x+1\right)=7y\left(x-1\right)^2\)
phân tích đa thức thành nhân tử
3x mũ 2 +14xy
3x2 + 14xy = x(3x + 14y)
Phân tích đa thức thành nhân tử
b. 25-x2=14xy-49y2
c. x5+x4+1
Mọi người giúp mình với ạ
b) \(25-x^2+14xy-49y^2\)
\(=25-\left(x^2-14xy+49y^2\right)\)
\(=25-\left[x^2-2\cdot7y\cdot x+\left(7y\right)^2\right]\)
\(=25-\left(x-7y\right)^2\)
\(=5^2-\left(x-7y\right)^2\)
\(=\left[5-\left(x-7y\right)\right]\left[5+\left(x-7y\right)\right]\)
\(=\left(5-x+7y\right)\left(5+x-7y\right)\)
c) \(x^5+x^4+1\)
\(=x^5+x^4+1+x^3-x^3\)
\(=\left(x^5+x^4+x^3\right)+\left(1-x^3\right)\)
\(=x^3\left(x^2+x+1\right)+\left(1-x\right)\left(x^2+x+1\right)\)
\(=\left(x^2+x+1\right)\left[x^3+\left(1-x\right)\right]\)
\(=\left(x^2+x+1\right)\left(x^3+1-x\right)\)
b: 25-x^2+14xy-49y^2
=25-(x-7y)^2
=(5-x+7y)(5+x-7y)
c: =x^5+x^4+x^3+1-x^3
=x^3(x^2+x+1)+(1-x)(x^2+x+1)
=(x^2+x+1)(x^3+1-x)
7x2+15x-5=0
7*(x2+2.5x-5/7)=0
x2+2*x*1.25+1,5625-255/112=0
(x+1.25)2-255/112=0
Phân tích đa thức thành nhân tử:
a) x 4 - 6 x 3 + 12 x 2 - 14x + 3.
b) x 4 + 6 x 3 + 7 x 2 -6x + l.
a) ( x 2 – 4x + 1)( x 2 – 2x + 3).
b) ( x 2 + 5x – 1)( x 2 + x – 1).
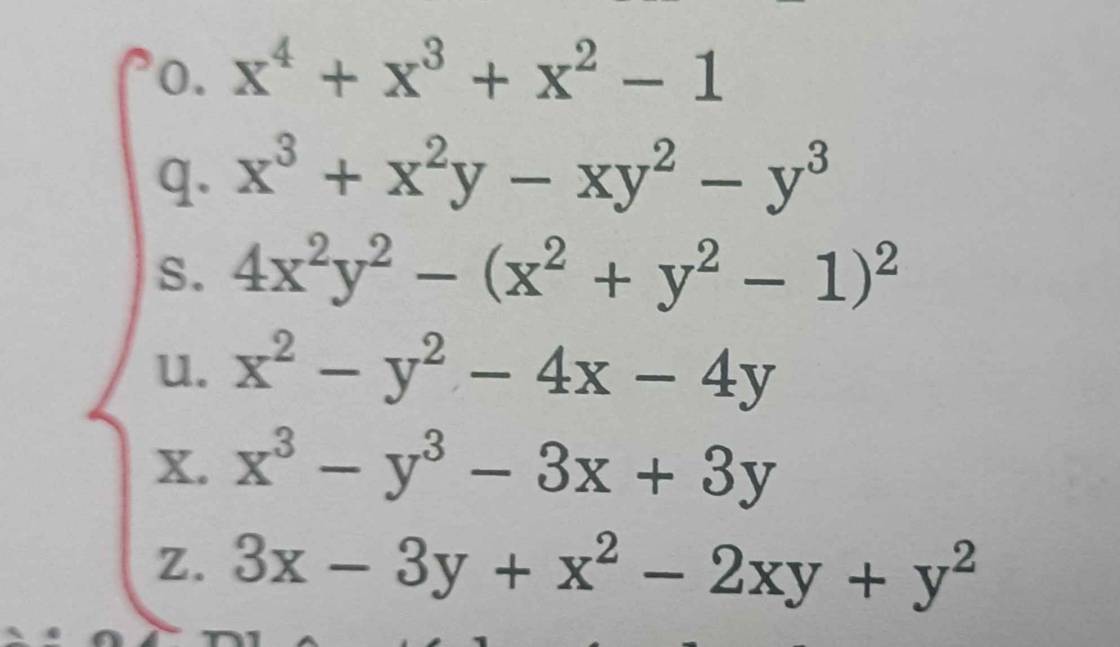 Đề Bài : Phân tích các đa thức sau thành nhân tử. Giúp E Với ạ 🥲
Đề Bài : Phân tích các đa thức sau thành nhân tử. Giúp E Với ạ 🥲
o: x^4+x^3+x^2-1
=x^3(x+1)+(x-1)(x+1)
=(x+1)(x^3+x-1)
q: \(=\left(x^3-y^3\right)+xy\left(x-y\right)\)
=(x-y)(x^2+xy+y^2)+xy(x-y)
=(x-y)(x^2+2xy+y^2)
=(x-y)(x+y)^2
s: =(2xy)^2-(x^2+y^2-1)^2
=(2xy-x^2-y^2+1)(2xy+x^2+y^2-1)
=[1-(x^2-2xy+y^2]+[(x+y)^2-1]
=(1-x+y)(1+x-y)(x+y-1)(x+y+1)
u: =(x^2-y^2)-4(x+y)
=(x+y)(x-y)-4(x+y)
=(x+y)(x-y-4)
x: =(x^3-y^3)-(3x-3y)
=(x-y)(x^2+xy+y^2)-3(x-y)
=(x-y)(x^2+xy+y^2-3)
z: =3(x-y)+(x^2-2xy+y^2)
=3(x-y)+(x-y)^2
=(x-y)(x-y+3)
o) \(x^4+x^3+x^2-1\)
\(=\left(x^4+x^3\right)+\left(x^2-1\right)\)
\(=x^3\left(x+1\right)+\left(x+1\right)\left(x-1\right)\)
\(=\left(x+1\right)\left(x^3+x-1\right)\)
q) \(x^3+x^2y-xy^2-y^3\)
\(=\left(x^3+x^2y\right)-\left(xy^2+y^3\right)\)
\(=x^2\left(x+y\right)-y^2\left(x+y\right)\)
\(=\left(x+y\right)\left(x^2-y^2\right)\)
\(=\left(x+y\right)^2\left(x-y\right)\)
s) \(4x^2y^2-\left(x^2+y^2-1\right)^2\)
\(=\left(2xy\right)^2-\left(x^2+y^2-1\right)^2\)
\(=\left(2xy-x^2-y^2+1\right)\left(2xy+x^2+y^2-1\right)\)
\(=-\left(x^2-2xy+y^2-1\right)\left(x^2+2xy+y^2-1\right)\)
\(=-\left(x-y-1\right)\left(x-y+1\right)\left(x+y+1\right)\left(x+y-1\right)\)
u) \(x^2-y^2-4x-4y\)
\(=\left(x^2-y^2\right)-\left(4x+4y\right)\)
\(=\left(x+y\right)\left(x-y\right)-4\left(x+y\right)\)
\(=\left(x+y\right)\left(x-y-4\right)\)
x) \(x^3-y^3-3x+3y\)
\(=\left(x^3-y^3\right)-\left(3x-3y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2\right)-3\left(x-y\right)\)
\(=\left(x-y\right)\left(x^2+xy+y^2-3\right)\)
z) \(3x-3y+x^2-2xy+y^2\)
\(=\left(3x-3y\right)+\left(x^2-2xy+y^2\right)\)
\(=3\left(x-y\right)+\left(x-y\right)^2\)
\(=\left(x-y\right)\left(3+x-y\right)\)