Giải phương trình
1,sin3x+cos2x=1+2sinx*cos2x
2,cos5x+cos2x+2sin3x*sin2x=0
Giải phương trình
1, cos2x + cos6x + cos3x + cos5x = 0
2, sinx + sin2x + sin3x = 0
3, sinx + sin2x + sin3x + sin4x = 0
\( 2)\sin x + \sin 2x + \sin 3x = 0\\ \Leftrightarrow 2\sin 2x.\cos x + \sin 2x = 0\\ \Leftrightarrow \sin 2x\left( {2\cos x + 1} \right) = 0\\ \Leftrightarrow \left[ \begin{array}{l} \sin 2x = 0\\ 2\cos x + 1 = 0 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} 2x = k\pi \\ \cos x = \dfrac{{ - 1}}{2} \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = \dfrac{{k\pi }}{2}\\ x = \pm \dfrac{{2\pi }}{3} + k2\pi \end{array} \right.\left( {k \in \mathbb{Z} } \right) \)
\( 3)\sin x + \sin 2x + \sin 3x + \sin 4x = 0\\ \Leftrightarrow \left( {\sin x + \sin 4x} \right) + \left( {\sin 2x + \sin 3x} \right) = 0\\ \Leftrightarrow 2\sin \dfrac{{5x}}{2}.\cos \dfrac{{3x}}{2} + 2\sin \dfrac{{5x}}{2}.\cos \dfrac{x}{2} = 0\\ \Leftrightarrow \sin \dfrac{{5x}}{2}.\left( {\cos \dfrac{{3x}}{2} + \cos \dfrac{x}{2}} \right) = 0\\ \Leftrightarrow \sin \dfrac{{5x}}{2}.2\cos x.\cos \dfrac{x}{2} = 0\\ \Leftrightarrow \left[ \begin{array}{l} \sin \dfrac{{5x}}{2} = 0\\ 2\cos x = 0\\ \cos \dfrac{x}{2} = 0 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = \dfrac{{2k\pi }}{5}\\ x = \dfrac{\pi }{2} + k\pi \\ x = \pi + 2k\pi \end{array} \right.\left( {k \in \mathbb{Z}} \right) \)
giải phương trình
1. sin2x+3sinx-cos2x=-2
2. sin2x+sinx-cos2x=0
`1)sin^2 x+3sin x-cos^2 x=-2`
`<=>sin^2 x+3sin x-1+sin^2 x+2=0`
`<=>2sin^2 x+3sin x+1=0`
`<=>[(sin x=-1),(sin x=-1/2):}`
`<=>[(x=-\pi/2 +k2\pi),(x=-\pi/6 +k2\pi),(x=[7\pi]/6+k2\pi):}` `(k in ZZ)`
`2)sin^2 x+sin x-cos^2 x=0`
`<=>sin^2 x+sin x-1+sin^2 x=0`
`<=>2sin^2 x+sin x-1=0`
`<=>[(sin x=-1),(sin x=1/2):}`
`<=>[(x=-\pi/2 +k2\pi),(x=\pi/6 +k2\pi),(x=[5\pi]/6 +k2\pi):}` `(k in ZZ)`
Tìm nghiệm x của phương trình
2 sin 3 x + sin 2 x - sin x + 1 = 3 - 2 sin x - cos 2 x
![]()
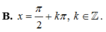
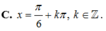

Tìm nghiệm x của phương trình 2 sin 3 x + sin 2 x - s i n x + 1 = 3-2sinx- cos 2 x
thỏa mãn điều kiện sin x < 1 2
![]()
![]()
![]()
![]()
Đáp án A
Phương trình đã cho tương đương với
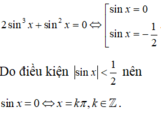
Rút gọn biểu thức
A = sin3x + cos2x - sinx/ cosx + sin2x - cos3x
( sin2x ≠0; 2sinx +1 ≠0)
\(A=\frac{sin3x-sinx+cos2x}{cosx-cos3x+sin2x}=\frac{2cos2x.sinx+cos2x}{2sin2x.sinx+sin2x}=\frac{cos2x\left(2sinx+1\right)}{sin2x\left(2sinx+1\right)}=\frac{cos2x}{sin2x}=cot2x\)
Tìm nghiệm x của phương trình
2 ( sin 3 x + sin 2 x - sin x + 1 ) = 3 - 2 sin x - cos 2 x
thỏa mãn điều kiện sin x < 1 2
A. x = kπ ; k ∈ ℤ
B. x = π 2 + k π ; k ∈ ℤ
C. x = π 6 + k π ; k ∈ ℤ
D. x ∈ ∅
Phương trình đã cho tương đương với
2 sin 3 x + sin 2 x = 0 ⇔ sin x = 0 sin x = - 1 2
Do điều kiện
sin
x
<
1
2
nên sinx = 0 nên
x
=
kπ
;
k
∈
ℤ
Đáp án A
9. Rút gọn các biểu thức sau
A= cos7x - cos8x - cos9x + cos10x / sin7x - sin8x - sin9x + sin10x
B = sin2x + 2sin3x + sin4x / sin3x +2sin4x + sin5x
C= 1+cosx + cos2x + cos3x / cosx + 2cos^2 . x -1
D = sin4x + sin5x + sin6x / cos4x + cos5x + cos6x
\(D=\frac{sin4x+sin5x+sin6x}{cos4x+cos5x+cos6x}\)
\(=\frac{\left(sin4x+sin6x\right)+sin5x}{\left(cos4x+cos6x\right)+cos5x}\)
\(=\frac{2sin\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+sin5x}{2cos\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+cos5x}\)
\(=\frac{2sin5x.cos\left(-x\right)+sin5x}{2cos5x.cos\left(-x\right)+cos5x}=\frac{sin5x\left(2.cos\left(-x\right)+1\right)}{cos5x\left(2.cos\left(-x\right)+1\right)}=\frac{sin5x}{cos5x}=tan5x\)
Rút gọn các biểu thức sau:
D = \(\frac{1+sin2x+cos2x}{1+sin2x-cos2x}\)E = \(\frac{sin2x+2sin3x+sin4x}{cos3x+2cos4x-cos5x}\)F = \(\frac{sinx+sin4x+sin7x}{cosx+cos4x+cos7x}\)G = \(\frac{cos2x-sin4x-cos6x}{cos2x+sin4x-cos6x}\)\(D=\frac{1+sin2x+cos2x}{1+sin2x-cos2x}=\frac{1+2sinxcosx+2cos^2x-1}{1+2sinxcosx-1+2sin^2x}\)
\(D=\frac{cosx\left(sinx+cosx\right)}{sinx\left(sinx+cosx\right)}=cotx\)
\(F=\frac{sinx+sin4x+sin7x}{cosx+cos4x+cos7x}\)
\(F=\frac{2sin4xcos3x+sin4x}{2cos4xcos3x+cos4x}\)
\(F=\frac{2sin4x\left(cos3x+1\right)}{2cos4x\left(cos3x+1\right)}=tan4x\)
\(G=\frac{cos2x-sin4x-cos6x}{cos2x+sin4x-cos6x}=\frac{-2sin4xsin2x-sin4x}{-2sin4xsin2x+sin4x}\)
\(G=\frac{-sin4x\left(2sin2x+1\right)}{-sin4x\left(2sin2x-1\right)}=\frac{2sin2x+1}{2sin2x-1}\)
Giải các pt
a) \(\left(cos2x+1\right)\left(cos2x+2\right)=0\)
b) \(cos5x-sin40^o=0\)
c) \(sin3x+cosx=0\)
d) \(sin2x+\sqrt{3}sinx=0\)
a/
\(\Leftrightarrow\left[{}\begin{matrix}cos2x+1=0\\cos2x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=-1\\cos2x=-2\left(vn\right)\end{matrix}\right.\)
\(\Rightarrow2x=\pi+k2\pi\)
\(\Rightarrow x=\frac{\pi}{2}+k\pi\)
b/
\(\Leftrightarrow cos5x=sin40^0\)
\(\Leftrightarrow cos5x=cos50^0\)
\(\Leftrightarrow\left[{}\begin{matrix}5x=50^0+k360^0\\5x=-50^0+k360^0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=10^0+k72^0\\x=-10^0+k72^0\end{matrix}\right.\)
c/
\(\Leftrightarrow sin3x=-cosx\)
\(\Leftrightarrow sin3x=sin\left(x-\frac{\pi}{2}\right)\)
\(\Rightarrow\left[{}\begin{matrix}3x=x-\frac{\pi}{2}+k2\pi\\3x=\frac{3\pi}{2}-x+k2\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-\frac{\pi}{4}+k\pi\\x=\frac{3\pi}{8}+\frac{k\pi}{2}\end{matrix}\right.\)
d/
\(\Leftrightarrow2sinx.cosx+\sqrt{3}sinx=0\)
\(\Leftrightarrow sinx\left(2cosx+\sqrt{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\cosx=-\frac{\sqrt{3}}{2}=cos\left(\frac{5\pi}{6}\right)\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=k\pi\\x=\frac{5\pi}{6}+k2\pi\\x=-\frac{5\pi}{6}+k2\pi\end{matrix}\right.\)