Giải phương trình :
\(2\log^2_2x-14\log_4x+3=0\)
Giải phương trình : \(\log^2_{\frac{1}{2}}x^2-\log_4x^4-20=0\)
-log22 x2_ log2x2- 20=0
↔ pt này vô ng bạn ơi!!! xem lại đầu bài.
Bài này phương trình có tận 4 nghiệm chứ không phải vô nghiệm đâu bạn Đỗ đại học nhé
Điều kiện \(x\ne0\)
Ta có từ phương trình ban đầu cho \(\Leftrightarrow4\log_2^2\left|x\right|-2\log_2\left|x\right|-20=0\)
\(\Leftrightarrow2\log_2^2\left|x\right|-\log_2\left|x\right|-10=0\)
Đặt \(t=\log_2\left|x\right|\) ta được phương trình \(2t^2-t-10=0\Leftrightarrow\begin{cases}t=-2\\t=\frac{5}{2}\end{cases}\)
Với \(t=2\Rightarrow\log_2\left|x\right|=-2\Leftrightarrow\left|x\right|=\frac{1}{4}\Leftrightarrow x=\pm\frac{1}{4}\)
Với \(t=\frac{5}{2}\Rightarrow\log_2\left|x\right|=\frac{5}{2}\Leftrightarrow\left|x\right|=\sqrt{32}\Leftrightarrow x=\pm\sqrt{32}\)
Vậy phương trình có 4 nghiệm : \(x=\frac{1}{4};x=-\frac{1}{4};x=\sqrt{32};x=-\sqrt{32}\)
giải các phương trình sau
a) \(\log_3\left(2x-5\right)=3\)
b) \(\log_4x^2=2\)
c) \(\log_7\left(3x-1\right)=\log_7\left(2x+5\right)\)
d) \(\ln\left(4x^2+2x-3\right)=\ln\left(3x^2-3\right)\)
e) \(\log\left(2x+3\right)=log\left(1-3x\right)\)
a: ĐKXĐ: \(x\notin\left\{\dfrac{5}{2}\right\}\)
\(\log_32x-5=3\)
=>\(log_3\left(2x-5\right)=log_327\)
=>2x-5=27
=>2x=32
=>x=16(nhận)
b: ĐKXĐ: x<>0
\(\log_4x^2=2\)
=>\(log_4x^2=log_416\)
=>\(x^2=16\)
=>\(\left[{}\begin{matrix}x=4\left(nhận\right)\\x=-4\left(nhận\right)\end{matrix}\right.\)
c: ĐKXĐ: \(x\notin\left\{\dfrac{1}{3};-\dfrac{5}{2}\right\}\)
\(\log_7\left(3x-1\right)=\log_7\left(2x+5\right)\)
=>3x-1=2x+5
=>x=6(nhận)
d: ĐKXĐ: \(x\notin\left\{1;-1;\dfrac{-1+\sqrt{13}}{4};\dfrac{-1-\sqrt{13}}{4}\right\}\)
\(ln\left(4x^2+2x-3\right)=ln\left(3x^2-3\right)\)
=>\(4x^2+2x-3=3x^2-3\)
=>\(x^2+2x=0\)
=>x(x+2)=0
=>\(\left[{}\begin{matrix}x=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(nhận\right)\\x=-2\left(nhận\right)\end{matrix}\right.\)
e: ĐKXĐ: \(x\notin\left\{-\dfrac{3}{2};\dfrac{1}{3}\right\}\)
\(log\left(2x+3\right)=log\left(1-3x\right)\)
=>2x+3=1-3x
=>5x=-2
=>\(x=-\dfrac{2}{5}\left(nhận\right)\)
Giải các bất phương trình sau :
a) \(\left(2x-7\right)\ln\left(x+1\right)>0\)
b) \(\left(x-5\right)\left(\log x+1\right)< 0\)
c) \(2\log^3_2x+5\log^2_2x+\log_2x-2\ge0\)
d) \(\ln\left(3e^x-2\right)\le2x\)
Giải các phương trình sau :
a) \(13^{2x+1}-13^x-12=0\)
b) \(\left(3^x+2^x\right)\left(3^x+3.2^x\right)=8.6^x\)
c) \(\log_{\sqrt{3}}\left(x-2\right).\log_5x=2.\log_3\left(x-2\right)\)
d) \(\log^2_2x-5\log_2x+6=0\)
a) Đặt t = 13x > 0 ta được phương trình:
13t2 – t – 12 = 0 ⇔ (t – 1)(13t + 12) = 0
⇔ t = 1 ⇔ 13x = 1 ⇔ x = 0
b)
Chia cả hai vế phương trình cho 9x ta được phương trình tương đương
(1+(23)x)(1+3.(23)x)=8.(23)x(1+(23)x)(1+3.(23)x)=8.(23)x
Đặt t=(23)xt=(23)x (t > 0) , ta được phương trình:
(1 + t)(1 + 3t) = 8t ⇔ 3t2 – 4t + 1 = 0 ⇔ t∈{13,1}t∈{13,1}
Với t=13t=13 ta được nghiệm x=log2313x=log2313
Với t = 1 ta được nghiệm x = 0
c) Điều kiện: x > 2
Vì nên phương trình đã cho tương đương với:
[log3(x−2)=0log5x=1⇔[x=3x=5[log3(x−2)=0log5x=1⇔[x=3x=5
d) Điều kiện: x > 0
log22x – 5log2x + 6 = 0
⇔(log2x – 2)(log2x – 3) = 0
⇔ x ∈ {4, 8}
Giải các bất phương trình sau :
a) \(\dfrac{2^x}{3^x-2^x}\le2\)
b) \(\left(\dfrac{1}{2}\right)^{\log_2\left(x^2-1\right)}>1\)
c) \(\log^2x+3\log x\ge4\)
d) \(\dfrac{1-\log_4x}{1+\log_2x}\le\dfrac{1}{4}\)
Giải mỗi phương trình sau:
a) \({\log _5}\left( {2x - 4} \right) + {\log _{\frac{1}{5}}}\left( {x - 1} \right) = 0\)
b) \({\log _2}x + {\log _4}x = 3\)
a)
ĐK: \(\left\{{}\begin{matrix}2x-4>0\\x-1>0\end{matrix}\right.\Leftrightarrow x>1\)
\(\log_5\left(2x-4\right)+\log_{\dfrac{1}{5}}\left(x-1\right)=0\\ \Leftrightarrow\log_5\left(2x-4\right)-\log_5\left(x-1\right)=0\\ \Leftrightarrow\log_5\left(\dfrac{2x-4}{x-1}\right)=\log_51\\ \Leftrightarrow\dfrac{2x-4}{x-1}=1\\ \Leftrightarrow2x-4=x-1\\ \Leftrightarrow x=3\left(tm\right)\)
Vậy x = 3.
b) ĐK: x > 0
\(\log_2x+\log_4x=3\\ \Leftrightarrow\log_2x+\dfrac{1}{2}\log_2x=3\\ \Leftrightarrow\left(1+\dfrac{1}{2}\right)\log_2x=3\\ \Leftrightarrow\dfrac{3}{2}\log_2x=3\\ \Leftrightarrow\log_2x=2\\ \Leftrightarrow x=4\left(tm\right)\)
Vậy x= 4
Giải các bất phương trình sau:
a) \(0,{1^{2 - x}} > 0,{1^{4 + 2x}};\)
b) \({2.5^{2x + 1}} \le 3;\)
c) \({\log _3}\left( {x + 7} \right) \ge - 1;\)
d) \({\log _{0,5}}\left( {x + 7} \right) \ge {\log _{0,5}}\left( {2x - 1} \right).\)
\(a,0,1^{2-x}>0,1^{4+2x}\\ \Leftrightarrow2-x>2x+4\\ \Leftrightarrow3x< -2\\ \Leftrightarrow x< -\dfrac{2}{3}\)
\(b,2\cdot5^{2x+1}\le3\\ \Leftrightarrow5^{2x+1}\le\dfrac{3}{2}\\ \Leftrightarrow2x+1\le log_5\left(\dfrac{3}{2}\right)\\ \Leftrightarrow2x\le log_5\left(\dfrac{3}{2}\right)-1\\ \Leftrightarrow x\le\dfrac{1}{2}log_5\left(\dfrac{3}{2}\right)-\dfrac{1}{2}\\ \Leftrightarrow x\le log_5\left(\dfrac{\sqrt{30}}{10}\right)\)
c, ĐK: \(x>-7\)
\(log_3\left(x+7\right)\ge-1\\ \Leftrightarrow x+7\ge\dfrac{1}{3}\\ \Leftrightarrow x\ge-\dfrac{20}{3}\)
Kết hợp với ĐKXĐ, ta có:\(x\ge-\dfrac{20}{3}\)
d, ĐK: \(x>\dfrac{1}{2}\)
\(log_{0,5}\left(x+7\right)\ge log_{0,5}\left(2x-1\right)\\ \Leftrightarrow x+7\le2x-1\\ \Leftrightarrow x\ge8\)
Kết hợp với ĐKXĐ, ta được: \(x\ge8\)
Giải bất phương trình l o g ( x 2 - 2 x - 2 ) ≤ 0
A. [-1; 3]
B. ( 1 - 3 ; 1 + 3 )
C. [ - 1 ; 1 - 3 ) ∪ ( 1 + 3 ; 3 ]
D. (-∞; -1) ∪ (3; +∞)
Chọn C
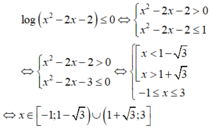
⇒ [ - 1 ; 1 - 3 ) ∪ ( 1 + 3 ; 3 ]
Giải bất phương trình l o g ( x 2 - 2 x - 2 ) ≤ 0
A. [-1; 3]
B. ( 1 - 3 ; 1 + 3 )
C. [ - 1 ; 1 - 3 ) ∪ ( 1 + 3 ; 3 ]
D. (-∞; -1) ∪ (3; +∞)

⇒ [ - 1 ; 1 - 3 ) ∪ ( 1 + 3 ; 3 ]
Chọn C