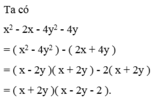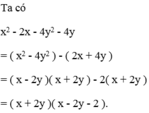$A=x^2+y^2-6x+4y+20=(x^2-6x+9)+(y^2+4y+4)+7$

$=(x-3)^2+(y+2)^2+7\geq 0+0+7=7$
Vậy $A_{\min}=7$. Giá trị này đạt tại $(x-3)^2=(y+2)^2=0$

$\Leftrightarrow x=3; y=-2$

---------------------

$B=9x^2+y^2+2z^2-18x+4z-6y+30$

$=(9x^2-18x+9)+(y^2-6y+9)+(2z^2+4z+2)+10$

$=9(x^2-2x+1)+(y^2-6y+9)+2(z^2+2z+1)+10$

$=9(x-1)^2+(y-3)^2+2(z+1)^2+10\geq 10$
Vậy $B_{\min}=10$. Giá trị này đạt tại $(x-1)^2=(y-3)^2=(z+1)^2$

$\Leftrightarrow x=1; y=3; z=-1$

$C=x^2+y^2+z^2-xy-yz-xz+3$

$2C=2x^2+2y^2+2z^2-2xy-2yz-2xz+6$

$=(x^2-2xy+y^2)+(y^2-2yz+z^2)+(x^2-2xz+z^2)+6$

$=(x-y)^2+(y-z)^2+(z-x)^2+6\geq 6$

$\Rightarrow C\geq 3$

Vậy $C_{\min}=3$. Giá trị này đạt tại $x-y=y-z=z-x=0$

$\Leftrihgtarrow x=y=z$

--------------------------------------

$D=5x^2+2y^2+4xy-2x+4y+2021$

$=2(y^2+2xy+x^2)+3x^2-2x+4y+2021$

$=2(x+y)^2+4(x+y)+3x^2-6x+2021$
$=2(x+y)^2+4(x+y)+2+3(x^2-2x+1)+2016$

$=2[(x+y)^2+2(x+y)+1]+3(x^2-2x+1)+2016$

$=2(x+y+1)^2+3(x-1)^2+2016\geq 2016$

Vậy $D_{\min}=2016$ khi $x+y+1=x-1=0$

$\Leftrightarrow x=1; y=-2$

$E=x^2-2x+4y^2+4y+2014$

$=(x^2-2x+1)+(4y^2+4y+1)+2012$

$=(x-1)^2+(2y+1)^2+2012$

$\geq 2012$

Vậy $E_{\min}=2012$. Giá trị này đạt tại $x-1=2y+1=0$

$\Leftrightarrow x=1; y=\frac{-1}{2}$

----------------------

$F=5x^2+5y^2+8xy+2y-2x+30$

$=4(x^2+2xy+y^2)+x^2+y^2+2y-2x+30$

$=4(x+y)^2+(x^2-2x+1)+(y^2+2y+1)+28$

$=4(x+y)^2+(x-1)^2+(y+1)^2+28\geq 28$

Vậy $F_{\min}=28$. Giá trị này đạt tại $x+y=x-1=y+1=0$

$\Leftrightarrow x=1; y=-1$

\(a,\Leftrightarrow\left(9x^2-18x+9\right)+\left(y^2-6y+9\right)+\left(2z^2+4z+2\right)=0\\ \Leftrightarrow9\left(x-1\right)^2+\left(y-3\right)^2+2\left(z+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=3\\z=-1\end{matrix}\right.\)

\(b,\Leftrightarrow\left(4x^2+8xy+4y^2\right)+\left(x^2-2x+1\right)+\left(y^2+2y+1\right)=0\\ \Leftrightarrow4\left(x+y\right)^2+\left(x-1\right)^2+\left(y+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=-y\\x=1\\y=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)

\(c,\Leftrightarrow\left(4x^2+4xy+y^2\right)+\left(x^2-2x+1\right)+\left(y^2+4y+4\right)=0\\ \Leftrightarrow\left(2x+y\right)^2+\left(x-1\right)^2+\left(y+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x=-y\\x=1\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)

a,9x^2+y^2+2z^2−18x+4z−6y+20=0

⇔9(x−1)^2+(y−3)^2+2(z+1)^2=0

⇔x=1;y=3;z=−1

b,5x^2+5y^2+8xy+2y−2x+2=0

⇔4(x+y)2+(x−1)2+(y+1)2=0

⇔x=−y;x=1y=−1⇔x=1y=−1

c,5x^2+2y^2+4xy−2x+4y+5=0

⇔(2x+y)^2+(x−1)^2+(y+2)^2=0

⇔2x=−y;x=1;y=−2

⇔x=1;y=−2

⇔(x−1)^2+(2y−3)^2+(z+2)^2=0

\(d,\Leftrightarrow\left(x^2-2x+1\right)+\left(4y^2-12y+9\right)+\left(z^2+4z+4\right)=0\\ \Leftrightarrow\left(x-1\right)^2+\left(2y-3\right)^2+\left(z+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\dfrac{3}{2}\\z=-2\end{matrix}\right.\)

\(e,x^2+y^2-6x+4y+2=0\\ \Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=11\)

\(\Rightarrow\)PT vô nghiệm vì 11 không phải là tổng 2 số chính phương

`a)x^2-2x+2+4y^2+4y`

`=x^2-2x+1+4y^2+4y+1`

`=(x-1)^2+(2y+1)^2`

`b)4x^2+y^2+12x+4y+13`

`=4x^2+12x+9+y^2+4y+4`

`=(2x+3)^2+(y+2)^2`

`c)x^2+17+4y^2+8x+4y`

`=x^2+8x+16+4y^2+4y+1`

`=(x+4)^2+(2y+1)^2`

`d)4x^2-12xy+y^2-4y+13`

`=4x^2-12x+9+y^2-4y+4`

`=(2x-3)^2+(y-2)^2`

a) \(x^2-2x+2+4y^2+4y=\left(x-1\right)^2+\left(2y+1\right)^2\)

b) \(4x^2+y^2+12x+4y+13=\left(2x+3\right)^2+\left(y+2\right)^2\)

c) \(x^2+17+4y^2+8x+4y=\left(x+4\right)^2+\left(2y+1\right)^2\)

d) \(4x^2-12x+y^2-4y+13=\left(2x-3\right)^2+\left(y-2\right)^2\)

a: \(x^2-2x+2+4y^2+4y\)

\(=x^2-2x+1+4y^2+4y+1\)

\(=\left(x-1\right)^2+\left(2y+1\right)^2\)

b: \(4x^2+12x+y^2+4y+13\)

\(=4x^2+12x+9+y^2+4y+4\)

\(=\left(2x+3\right)^2+\left(y+2\right)^2\)

c: \(x^2+8x+4y^2+4y+17\)

\(=x^2+8x+16+4y^2+4y+1\)

\(=\left(x+4\right)^2+\left(2y+1\right)^2\)

d: \(4x^2-12x+y^2-4y+13\)

\(=4x^2-12x+9+y^2-4y+4\)

\(=\left(2x-3\right)^2+\left(y-2\right)^2\)

Ta có:

\(3-S=\left(x^2+4y^2+9z^2\right)-\left(2x+4y+6z\right)\)

\(\Leftrightarrow3-S=\left(x^2-2x+1\right)+\left(4y^2-4y+1\right)+\left(9z^2-6z+1\right)-3\)

\(\Leftrightarrow6-S=\left(x-1\right)^2+\left(2y-1\right)^2+\left(3z-1\right)^2\ge0\)

\(\Leftrightarrow S\le6\)

\(S_{max}=6\) khi \(\left\{{}\begin{matrix}x-1=0\\2y-1=0\\3z-1=0\end{matrix}\right.\) \(\Leftrightarrow\left(x;y;z\right)=\left(1;\dfrac{1}{2};\dfrac{1}{3}\right)\)

Phân tích đa thức thành nhân tử

1: \(x^2-x-y^2-y\)

\(=\left(x^2-y^2\right)-\left(x+y\right)\)

\(=\left(x+y\right)\left(x-y\right)-\left(x+y\right)\)

\(=\left(x+y\right)\left(x-y-1\right)\)

2: \(x^2-y^2+x-y\)

\(=\left(x^2-y^2\right)+\left(x-y\right)\)

\(=\left(x-y\right)\left(x+y\right)+\left(x-y\right)\)

\(=\left(x-y\right)\left(x+y+1\right)\)

3: \(3x-3y+x^2-y^2\)

\(=\left(3x-3y\right)+\left(x^2-y^2\right)\)

\(=3\left(x-y\right)+\left(x-y\right)\left(x+y\right)\)

\(=\left(x-y\right)\left(x+y+3\right)\)

4: \(5x-5y+x^2-y^2\)

\(=\left(5x-5y\right)+\left(x^2-y^2\right)\)

\(=5\left(x-y\right)+\left(x-y\right)\left(x+y\right)\)

\(=\left(x-y\right)\left(5+x+y\right)\)

5: \(x^2-5x-y^2-5y\)

\(=\left(x^2-y^2\right)-\left(5x+5y\right)\)

\(=\left(x-y\right)\left(x+y\right)-5\left(x+y\right)\)

\(=\left(x+y\right)\left(x-y-5\right)\)

6: \(x^2-y^2+2x-2y\)

\(=\left(x^2-y^2\right)+\left(2x-2y\right)\)

\(=\left(x-y\right)\left(x+y\right)+2\left(x-y\right)\)

\(=\left(x-y\right)\left(x+y+2\right)\)

7: \(x^2-4y^2+x+2y\)

\(=\left(x^2-4y^2\right)+\left(x+2y\right)\)

\(=\left(x+2y\right)\left(x-2y\right)+\left(x+2y\right)\)

\(=\left(x+2y\right)\left(x-2y+1\right)\)

8: \(x^2-y^2-2x-2y\)

\(=\left(x^2-y^2\right)-\left(2x+2y\right)\)

\(=\left(x-y\right)\left(x+y\right)-2\left(x+y\right)\)

\(=\left(x+y\right)\left(x-y-2\right)\)

9: \(x^2-4y^2+2x+4y\)

\(=\left(x^2-4y^2\right)+\left(2x+4y\right)\)

\(=\left(x-2y\right)\left(x+2y\right)+2\left(x+2y\right)\)

\(=\left(x+2y\right)\left(x-2y+2\right)\)

`a)x^3-8x^2+16x`

`=x(x^2-8x+16)`

`=x(x-4)^2`

`b)x^2+4y^2+2x-4y-4xy-24`

`=(x-2y)^2+2(x-2y)-24`

`=(x-2y)^2-4(x-2y)+6(x-2y)-24`

`=(x-2y-4)(x-2y+6)`

`c)x^4+x^3-x^2-2x-2`

`=x^4-2x^2+x^3-2x+x^2-2`

`=x^2(x^2-2)+x(x^2-2)+x^2-2`

`=(x^2-2)(x^2+x+1)`

\(=\left(x-2y\right)\left(x+2y\right)-2\left(x+2y\right)=\left(x+2y\right)\left(x-2y-2\right)\)

= ( x² - 4y² ) - ( 2x + 4y )

= ( x - 2y ) ( x + 2y ) - 2 ( x - 2y )

= ( x - 2y ) ( x + 2y - 2 )