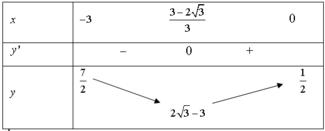
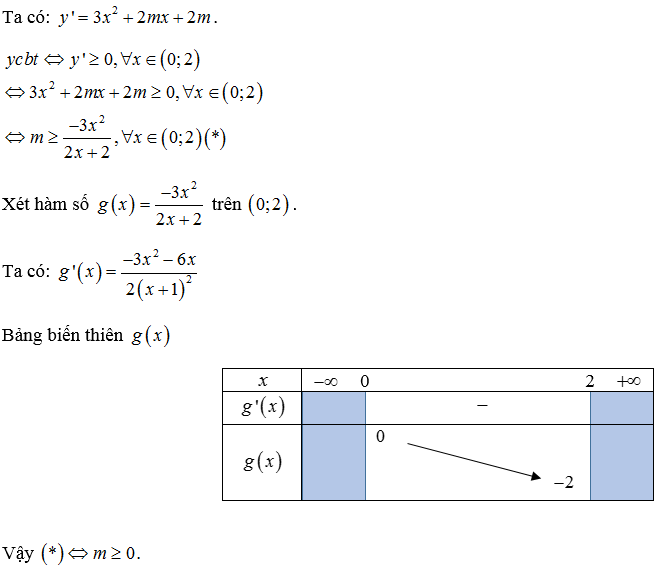
Bài 1 : Cho hàm số y 3(2mx - 1) + m + 2 (d)a. Vẽ đồ thị hàm số với m dfrac{1}{2}b. Tìm m để hàm số nghịch biến trên tập xác định.c. Tìm m để (d) vuông góc với đường thẳng (△) : y 6x + 1d. Tìm điểm cố định luôn nằm trên đường thẳng (d).e. Tìm khoảng cách lớn nhất từ gốc tọa độ O đên (d). Bài 2 : Cho hàm số y 3m - m - 1 (d)a. Vẽ đồ thị hàm số với m -1.b. Tìm m để hàm số vuông góc với đường thẳng (△) : y x + 1.c. Tìm m để đồ thị hàm số cắt trục tung tại điểm có tung độ là 2.d. Tìm điểm cố địn...
Đọc tiếp
Bài 1 : Cho hàm số y = 3(2mx - 1) + m + 2 (d)
a. Vẽ đồ thị hàm số với m = \(\dfrac{1}{2}\)
b. Tìm m để hàm số nghịch biến trên tập xác định.
c. Tìm m để (d) vuông góc với đường thẳng (△) : y = 6x + 1
d. Tìm điểm cố định luôn nằm trên đường thẳng (d).
e. Tìm khoảng cách lớn nhất từ gốc tọa độ O đên (d).
Bài 2 : Cho hàm số y = 3m - m - 1 (d)
a. Vẽ đồ thị hàm số với m = -1.
b. Tìm m để hàm số vuông góc với đường thẳng (△) : y = x + 1.
c. Tìm m để đồ thị hàm số cắt trục tung tại điểm có tung độ là 2.
d. Tìm điểm cố định luôn nằm trên (d).
e. Tìm m để khoảng cách từ gốc tọa độ O đến đường thẳng (d) là lớn nhất.
Bài 3 : Cho hàm số y = (4m - 3)x + m + 3
a. Vẽ đồ thị hàm số với m = 1.
b. Tìm m để hàm số nghịch biên trên tập xác đinh.
c. Tìm m để đồ thị hàm số cắt trục tung tại điểm có tung độ là -4.
d. Tìm điểm cố định luôn nằm trên (d).
e. Tìm m để khoảng cách từ gốc tọa độ O đến đường thẳng (d) là lớn nhất.