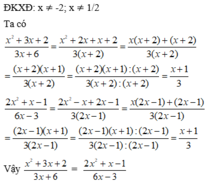x+6/x-5+x-5/x+6=2x2+23x+61/x2+x-30 júp minh voi nha thank
DV
Những câu hỏi liên quan
\(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2=x-30}\)
ĐKXĐ: x<>5; x<>-6
\(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2+x-30}\)
\(\Leftrightarrow x^2+12x+36+x^2-10x+25=2x^2+23x+61\)
=>23x+61=2x+61
=>x=0
Đúng 0
Bình luận (0)
Giải các phương trình sau:
a) \(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2+x-30}\)
b) \(\dfrac{x+5}{x-1}=\dfrac{x+1}{x-3}-\dfrac{8}{x^2-4x+3}\)
a, đk : x khác 5;-6
\(x^2+12x+36+x^2-10x+25=2x^2+23x+61\)
\(\Leftrightarrow2x+61=23x+61\Leftrightarrow21x=0\Leftrightarrow x=0\)(tm)
b, đk : x khác 1;3
\(x^2+2x-15=x^2-1-8\Leftrightarrow2x-15=-9\Leftrightarrow x=3\left(ktmđk\right)\)
pt vô nghiệm
Đúng 1
Bình luận (0)
a, đk : x khác 5;-6
x2+12x+36+x2−10x+25=2x2+23x+61x2+12x+36+x2−10x+25=2x2+23x+61
⇔2x+61=23x+61⇔21x=0⇔x=0⇔2x+61=23x+61⇔21x=0⇔x=0(tm)
b, đk : x khác 1;3
x2+2x−15=x2−1−8⇔2x−15=−9⇔x=3(ktmđk)x2+2x−15=x2−1−8⇔2x−15=−9⇔x=3(ktmđk)
pt vô nghiệm
a: \(\Leftrightarrow\left(x+6\right)^2+\left(x-5\right)^2=2x^2+23x+61\)
\(\Leftrightarrow x^2+12x+36+x^2-10x+25=2x^2+23x+61\)
=>x=0(nhận)
b: \(\Leftrightarrow\left(x+5\right)\left(x-3\right)=\left(x+1\right)\left(x-1\right)-8\)
\(\Leftrightarrow x^2+2x-15=x^2-1-8\)
=>2x-15=-9
=>2x=-6
hay x=-3(nhận)
Xem thêm câu trả lời
giải phương trình chứa ẩn ở mẫu:
1) x+6/x-5 + x-5/x+6 = 2x^2+23x+61/x^2+x-30
2) 6/x-5 + x+2/x-8 = 18/(x-5)(8-x) - 1
3) x^2-x/x+3 - x^2/x-3 = 7x^2 - 3x/9-x^2
1: \(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2+x-30}\)
\(\Leftrightarrow x^2+12x+36+x^2-10x+25=2x^2+23x+61\)
=>23x+61=2x+61
hay x=0
2: \(\dfrac{6}{x-5}+\dfrac{x+2}{x-8}=\dfrac{18}{\left(x-5\right)\left(8-x\right)}-1\)
\(\Leftrightarrow6x-48+x^2-3x-10=-18-x^2+13x-40\)
\(\Leftrightarrow x^2+3x-58+x^2-13x+58=0\)
\(\Leftrightarrow2x^2-10x=0\)
=>2x(x-5)=0
=>x=0
c: \(\dfrac{x^2-x}{x+3}-\dfrac{x^2}{x-3}=\dfrac{7x^2-3x}{9-x^2}\)
\(\Leftrightarrow\left(x^2-x\right)\left(x-3\right)-x^2\left(x+3\right)=-7x^2+3x\)
\(\Leftrightarrow x^3-3x^2-x^2+3x-x^3-3x^2+7x^2-3x=0\)
\(\Leftrightarrow x^2=0\)
hay x=0
Đúng 1
Bình luận (0)
giải phương trình sau
x+6/x-5 + x-5/x+6 = 2x^2+23x+61/x^2+x-30
6/x-5 + x+2/x-8 = 18/(x-5)(8-x) - 1
3/x+1 - 1/x-2 = 9/(x+1)(2-x)
các bạn ơi .mình cần gấp lắm.các bạn giúp mình nhé.mình cảm ơn
giải phương trình :
a) \(\dfrac{x+2}{x+1}+\dfrac{3}{x-2}=\dfrac{3}{x^2-x-2}+1\)
b)\(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2+x-30}\)
a) điều kiện xác định : \(x\ne2;x\ne-1\)
ta có : \(\dfrac{x+2}{x+1}+\dfrac{3}{x-2}=\dfrac{3}{x^2-x-2}+1\)
\(\Leftrightarrow\dfrac{\left(x+2\right)\left(x-2\right)+3\left(x+1\right)}{\left(x+1\right)\left(x-2\right)}=\dfrac{3+x^2-x-2}{\left(x+1\right)\left(x-2\right)}\)
\(\Rightarrow x^2-4+3x+3=x^2-x+1\Leftrightarrow4x=2\Leftrightarrow x=\dfrac{1}{2}\left(tmđk\right)\)
vậy \(x=\dfrac{1}{2}\)
b) điều kiện xác định : \(x\ne5;x\ne-6\)
ta có : \(\dfrac{x+6}{x-5}+\dfrac{x-5}{x+6}=\dfrac{2x^2+23x+61}{x^2+x-30}\)
\(\Leftrightarrow\dfrac{\left(x+6\right)^2+\left(x-5\right)^2}{\left(x-5\right)\left(x+6\right)}=\dfrac{2x^2+23x+61}{\left(x-5\right)\left(x+6\right)}\)
\(\Rightarrow x^2+12x+36+x^2-25x+25=2x^2+23x+61\)
\(\Leftrightarrow-13x=23x\Leftrightarrow x=0\left(tmđk\right)\)
vậy \(x=0\)
Đúng 0
Bình luận (1)
Dùng tính chất cơ bản của phân thức chứng minh cặp phân thức sau bằng nhau
x
2
+
3
x
+
2
3
x
+
6
và
2
x
2
+
x
-
1
6
x...
Đọc tiếp
Dùng tính chất cơ bản của phân thức chứng minh cặp phân thức sau bằng nhau x 2 + 3 x + 2 3 x + 6 và 2 x 2 + x - 1 6 x - 3
Quy đồng mẫu thức a) 74x+1674�+16 và 11x2−1611�2−16b) 6(x+3)36(�+3)3 và x−32x(x+3)2�−32�(�+3)2 c) −61−x−61−� ; 3xx2+x+13��2+�+1 và x2−3x+5x3−1�2−3�+5�3−1 d)175x175� ; 24x−2y24�−2� và x−y8y2−2x2
Đọc tiếp
Quy đồng mẫu thức a) và b) và c) ; và d) ; và
a: ĐKXĐ: \(x\notin\left\{4;-4\right\}\)
\(\dfrac{7}{4x+16}=\dfrac{7}{4\left(x+4\right)}=\dfrac{7\left(x-4\right)}{4\left(x+4\right)\left(x-4\right)}\)
\(\dfrac{11}{x^2-16}=\dfrac{11\cdot4}{4\left(x^2-16\right)}=\dfrac{44}{4\left(x-4\right)\left(x+4\right)}\)
b: \(\dfrac{6}{x\left(x+3\right)^2};\dfrac{x-3}{2x\left(x+3\right)^2}\)
ĐKXĐ: \(x\notin\left\{0;-3\right\}\)
\(\dfrac{6}{x\left(x+3\right)^2}=\dfrac{6\cdot2}{2x\left(x+3\right)^2}=\dfrac{12}{2x\left(x+3\right)^2}\)
\(\dfrac{x-3}{2x\left(x+3\right)^2}=\dfrac{x-3}{2x\left(x+3\right)^2}\)
c: \(\dfrac{-6}{1-x};\dfrac{3x}{x^2+x+1};\dfrac{x^2-3x+5}{x^3-1}\)
ĐKXĐ: \(x\ne1\)
\(-\dfrac{6}{1-x}=\dfrac{6}{x-1}=\dfrac{6\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{6x^2+6x+6}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\dfrac{3x}{x^2+x+1}=\dfrac{3x\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{3x^2-3x}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\dfrac{x^2-3x+5}{x^3-1}=\dfrac{x^2-3x+5}{\left(x-1\right)\left(x^2+x+1\right)}\)
d: \(\dfrac{17}{5x};\dfrac{24}{x-2y};\dfrac{x-y}{8y^2-2x^2}\)
ĐKXĐ: \(x\ne0;x\ne\pm2y\)
\(\dfrac{17}{5x}=\dfrac{17\cdot2\left(x-2y\right)\left(x+2y\right)}{5x\cdot2\cdot\left(x-2y\right)\left(x+2y\right)}=\dfrac{34\left(x^2-4y^2\right)}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\dfrac{24}{x-2y}=\dfrac{24\cdot10x\left(x+2y\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\dfrac{240x\left(x+2y\right)}{10x\left(x-2y\right)\left(x+2y\right)}\)
\(\dfrac{x-y}{8y^2-2x^2}=\dfrac{-\left(x-y\right)}{2x^2-8y^2}=\dfrac{-\left(x-y\right)}{2\left(x-2y\right)\left(x+2y\right)}\)
\(=\dfrac{-5x\left(x-y\right)}{10x\left(x-2y\right)\left(x+2y\right)}=\dfrac{-5x^2+5xy}{10x\left(x-2y\right)\left(x+2y\right)}\)
Đúng 2
Bình luận (0)
Giải các phương trình
1) \(\frac{x+2}{x+1}+\frac{3}{x-2}=\frac{3}{x^2-x-2}+1\)
2) \(\frac{x+6}{x-5}+\frac{x-5}{x+6}=\frac{2x^2+23x+61}{x^2+x-30}\)
3) \(\frac{6}{x-5}+\frac{x+2}{x-8}=\frac{18}{\left(x-5\right)\left(8-x\right)}-1\)
mình cũng giải câu này rồi nhưng ko biết đúng ko
2)x+6/x-5 + x-5/x+6 = 2x2+23x+61/x2+x-30
dkxd:x khắc 5;x khác-6
mc:(x-5)(x+6)
2x2+2x+61 =2x2+23x+61
2x=23x
2x=0 suy ra x=0
23x=0 suyra x=0
s={0}
3)6/x-5 + x+2/x-8 = 18/9(x-5)(8-x) - 1
dkxd: x khác 5 ; x khác -8
mc(x-5)(x-8)
3x+x2-58 =36x-x2+264
3x-58=36x+264
3x-58=0 suy ra x=58/3
36x+264=0 suy ra x=-22/8
s={58/3;-22/3}
Đúng 0
Bình luận (0)
tìm x biết 30 ( x + 2 ) - 6 ( x - 5 ) - 23x = 100