tìm x∈Z để:
a,3x - 2/x+3
b,x^2 - 2x +4/x + 1
NL
Những câu hỏi liên quan
tìm a và b sao cho 2 đa thức f(x)=4x^3-3x^2+2x+2a+3b và g(x)=5x^4-4x^3+3x^2-2x-3a+2b cùng chia hết cho đa thức (x-3)
Áp dụng định lý Bezout ta có:
f(x) chia hết cho x-3 \(\Rightarrow f\left(3\right)=0\)
\(\Leftrightarrow2a+3b=-87\left(1\right)\)
g(x) chia hết cho x-3 \(\Rightarrow g\left(3\right)=0\)
\(\Leftrightarrow-3a+2b=-318\left(2\right)\)
Từ (1) và (2) \(\Rightarrow\hept{\begin{cases}2a+3b=-87\\-3a+2b=-318\end{cases}\Leftrightarrow}\hept{\begin{cases}a=60\\b=-69\end{cases}}\)
Vậy ...
tìm x , y thuộc Z biết :
a, 4.(x +1 ) - (3x-1) = 14
b, (x^2 - 9 ). (x +2 )=0
c,(x^2 + 9 ) . (2x - 4) = 0
d, xy + 2x - y = 2
bấm chữ 0 đúng sẽ ra câu trả lời .
Đúng 0
Bình luận (0)
tìm x , y thuộc Z biết ;
a, 4 .( x+1) - (3x - 1) = 14
b, (x^2 - 9 ). (x+ 2) =0
c, (x^2 +9) . (2x -4) =0
d, xy +2x -y =2
a)4x+4-3x+1=14
x+5=14
x=11
b)trường hợp 1 x2-9=0
x2=9
->x=3;-3
-trường hợp 2: x+2=0
x=-2
c)-th1:x2+9=0
x2=-9
->x rỗng
d)xy+2x-y-2=0
(xy-y)+(2x-2)=0
y(x-1)+2(x-1)=0
(y+2)(x-1)=0
th1: y+2=0
y=-2
th2:x-1=0
x=1
(th1: trường hợp 1)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
1. Cho d: y = (\(^{m^2}\) + 2m)x + m + 1 . Tìm m để:
a, d // d1: y = (m + 6)x - 2
b, d ⊥ d2: y = \(\dfrac{-1}{3}\)x - 3
c, d ≡ d3: y = -\(^{m^2}\).x + 1
2. Tìm d // d1: y = \(\dfrac{-1}{2}\)x + 1 và d đi qua giao điểm của d1: y = 4x - 3 và d2: y = -x + 1
Bài 1:
b: Để (d) vuông góc với (d2) thì \(\left(m^2+2m\right)\cdot\dfrac{-1}{3}=-1\)
\(\Leftrightarrow m^2+2m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=-3\\m=1\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Phân tích đa thức sau thành nhân tử bằng phương pháp nhóm nhiều hạng tử :a) x^4 + 2x³ - 4x - 4b) ( x - 1)( 2x + 1) + 3( x - 1)(x + 2)(2x + 1)c) ( 6x + 3 ) - ( 2x -5 )(2x + 1)d) ( x - 5)² + ( x + 5)( x - 5 ) - (5 - x) ( 2x + 1)e) (3x - 2 )( 4x - 3 ) - ( 2 - 3x )( x - 1 ) - 2( 3x - 2 )( x + 1)g) ( a - b)( a + 2b ) - ( b - a)( 2a - b) - ( a - b)( a + 3b )h) 5xy³ - 2xyz - 15y² + 6zi) ( x + y)( 2x - y) + ( 2x - y)( 3x - y) - ( y - 2x )l) ab³c² - a²b²c² + ab²c³ - a²bc³m) x²( y - z) + y²( z - x) + z² (...
Đọc tiếp
Phân tích đa thức sau thành nhân tử bằng phương pháp nhóm nhiều hạng tử :
a) x^4 + 2x³ - 4x - 4
b) ( x - 1)( 2x + 1) + 3( x - 1)(x + 2)(2x + 1)
c) ( 6x + 3 ) - ( 2x -5 )(2x + 1)
d) ( x - 5)² + ( x + 5)( x - 5 ) - (5 - x) ( 2x + 1)
e) (3x - 2 )( 4x - 3 ) - ( 2 - 3x )( x - 1 ) - 2( 3x - 2 )( x + 1)
g) ( a - b)( a + 2b ) - ( b - a)( 2a - b) - ( a - b)( a + 3b )
h) 5xy³ - 2xyz - 15y² + 6z
i) ( x + y)( 2x - y) + ( 2x - y)( 3x - y) - ( y - 2x )
l) ab³c² - a²b²c² + ab²c³ - a²bc³
m) x²( y - z) + y²( z - x) + z² ( x- y)
Giúp mình nhé mình cần gấp lắm á
chuyển về dạng nguyên thể rồi tính thể chất khối lượng sau đó quay về đang tìm mũ của nhiều số làm ra rồi thì dễ lắm bạn ạ k minh nha
Đúng 0
Bình luận (0)
a)\(\left(x^2-2\right)\left(x^2+2x+2\right)\)
b)\(\left(x-1\right)\left(2x+1\right)\left(3x+7\right)\)
c)\(-2\left(x-4\right)\left(2x+1\right)\)
d)\(\left(x-5\right)\left(4x+1\right)\)
e)\(3\left(x-2\right)\left(3x-2\right)\)
g)\(2\left(a-b\right)^2\)
h)\(\left(xy-3\right)\left(5y^2-2z\right)\)
i)\(\left(4x+1\right)\left(2x-y\right)\)
l)\(abc^2\left(b-a\right)\left(b+c\right)\)
m)\(\left(x-y\right)\left(y-z\right)\left(x-z\right)\)
Đúng 0
Bình luận (0)
Thu gọn và tính giá trị biểu thức
a) A= 3x^4 + 1/3xyz - 3x^4 - 4/3xyz + 2x^2y - 6z khi x=1; y=3 và z=1/3
b) B= 4x^3 - 2/7xyz - 4x^3 - 4/3xyz + 4x^2y khi x=-1; y=2 và z=-1/2
c) C= 4x^2 + 1/2xyz - 2/3xy^2z - 5x^2yz + 3/4xyz khi x=-1; /y/=2 và z=1/2
`#3107`
`a)`
`A=`\(3x^4 + \dfrac{1}3xyz - 3x^4 - \dfrac{4}3xyz + 2x^2y - 6z\)
`= (3x^4 - 3x^4) + (1/3xyz - 4/3xyz) + 2x^2y - 6z`
`= -xyz + 2x^2y - 6z`
Thay `x = 1; y = 3` và `z = 1/3` vào A
`A = -1*3*1/3 + 2*1^2*3 - 6*1/3`
`= -1 + 6 - 2`
`= 6 - 3`
`= 3`
Vậy, `A=3`
`b)`
`B=`\(4x^3 - \dfrac{2}7xyz - 4x^3 - \dfrac{4}3xyz + 4x^2y\)
`= (4x^3 - 4x^3) + (-2/7xyz - 4/3xyz) + 4x^2y`
`= -34/21 xyz + 4x^2y`
Thay `x = -1; y = 2` và `z = -1/2` vào B
`B = -34/21*(-1)*2*(-1/2) + 4*(-1)^2 * 2`
`= -34/21 + 8`
`= 134/21`
Vậy, `B = 134/21`
`c)`
`C=`\(4x^2 + \dfrac{1}2xyz - \dfrac{2}3xy^2z - 5x^2yz + \dfrac{3}4xyz\)
`= 4x^2 + (1/2xyz + 3/4xyz) - 2/3xy^2z - 5x^2yz `
`= 4x^2 + 5/4xyz - 2/3xy^2z - 5x^2yz`
Ta có:
`|y| = 2`
`=> y = +-2`
Thay `x = -1; y = 2` và `z = 1/2` vào C
`4*(-1)^2 + 5/4*(-1)*2*1/2 - 2/3*(-1)*2^2*1/2 - 5*(-1)^2*2*1/2`
`= 4 - 5/4 + 4/3 - 5`
`= -11/12`
Vậy, với `x = -1; y = 2; z = 1/2` thì `B = -11/12`
Thay `x = -1; y = -2; z = 1/2`
`B = 4*(-1)^2 + 5/4*(-1)*(-2)*1/2 - 2/3*(-1)*(-2)^2*1/2 - 5*(-1)^2*(-2)*1/2`
`= 4 + 5/4 + 4/3 + 5`
`= 139/12`
Vậy, với `x = -1; y = -2; z = 1/2` thì `B = 139/12.`
Đúng 3
Bình luận (0)
tìm x:
a)3(2x-3)+2(2-x)=-3
b)2x(x2-2)+x2(1-2x)-x2=-12
c)3x(2x+3)-(2x+5)(3x-2)=8
d)4x(x - 1) - 3(x2-5)-x2=(x-3)-(x+4)
e)2(3x-1)(2x+5)-6(2x-1)(x+2)=-6
a: Ta có: \(3\left(2x-3\right)+2\left(2-x\right)=-3\)
\(\Leftrightarrow6x-9+4-2x=-3\)
\(\Leftrightarrow4x=2\)
hay \(x=\dfrac{1}{2}\)
Đúng 2
Bình luận (1)
a)\(\dfrac{2x^2-3x-2}{2x+1}\)=3
b)\(\dfrac{x-3\sqrt{x}+4}{x-2\sqrt{x}}\)-\(\dfrac{1}{\sqrt{x}-2}\)
a: =>2x^2-3x-2=6x+2
=>2x^2-9x-4=0
=>\(x=\dfrac{9\pm\sqrt{113}}{4}\)
b: \(=\dfrac{x-3\sqrt{x}+4-\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\left(\sqrt{x}-2\right)^2}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}-2}{\sqrt{x}}\)
Đúng 0
Bình luận (0)
a) \(\dfrac{2x^2-3x-2}{2x+1}\)=3
b)B=\(\dfrac{x-3\sqrt{x}+4}{x-2\sqrt{x}}\)=\(\dfrac{1}{\sqrt{x}-2}\)
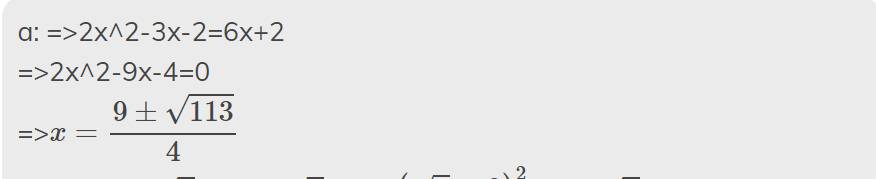
b: =>x-3căn x+4=căn x
=>(căn x-2)^2=0
=>căn x-2=0
=>x=4(loại)
Đúng 0
Bình luận (0)


