Tìm x,y,z biết x2+2x+y2-6y+4z^2-4z+11=0
HN
Những câu hỏi liên quan
Tìm x, y, z biết rằng: x^2 + 2x + y^2 – 6y + 4z^2 – 4z + 11 = 0
\(x^2+2x+y^2-6y+4z^2-4z+11=0\)
\(\Leftrightarrow x^2+2x+1+y^2-6y+9+4z^2-4z+1=0\)
\(\Leftrightarrow\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+1=0\\y-3=0\\2z-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=3\\z=\dfrac{1}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
\(x^2+2x+y^2-6y+4z^2-4z+11=0\\ \Rightarrow\left(x^2+2x+1\right)+\left(y^2-6y+9\right)+\left(4z^2-4z+1\right)=0\\ \Rightarrow\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2=0\)
Vì \(\left(x+1\right)^2\ge0;\left(y-3\right)^2\ge0;\left(2z-1\right)^2\ge0\) mà \(\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2=0\)
\(\Rightarrow\left\{{}\begin{matrix}\left(x+1\right)^2=0\\\left(y-3\right)^2=0\\\left(2z-1\right)^2=0\end{matrix}\right.\\ \Rightarrow\left\{{}\begin{matrix}x=-1\\y=3\\z=\dfrac{1}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
Tìm x,y, z biết
x2 + 2x +y2 - 6y + 4z2-4z + 11 =0
Tìm x, y,z
x^2 + 2x + y^2 - 6y + 4z^2- 4z + 11 = 0
Bài làm:
Ta có: \(x^2+2x+y^2-6y+4z^2-4z+11=0\)
\(\Leftrightarrow\left(x^2+2x+1\right)+\left(y^2-6y+9\right)+\left(4z^2-4z+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2=0\)
Dấu "=" xảy ra khi: \(\hept{\begin{cases}\left(x+1\right)^2=0\\\left(y-3\right)^2=0\\\left(2z-1\right)^2=0\end{cases}}\Rightarrow\hept{\begin{cases}x=-1\\y=3\\x=\frac{1}{2}\end{cases}}\)
Xin lỗi mk nhầm đoạn cuối là: \(\Rightarrow\hept{\begin{cases}x=-1\\y=3\\z=\frac{1}{2}\end{cases}}\) nhé:)
<=>x2+2x+1+y2-6y+9+4z2-4z+1=0
<=>(x2+2x+1)+(y2-6y+9)+(4z2-4z+1)=0
<=>(x+1)2+(y-3)2+(2z+1)2=0 (1)
Từ (1) <=> (x+1)2=0 <=> x+1=0 <=>x=-1
(y-3)2=0 <=>y-3=0 <=>y=3
(2z+1)2=0 <=>2z+1=0 <=> z=-1/2
Xem thêm câu trả lời
1) Tìm x, y, za) 9x2 +y2 + 2z2 – 18x +4z – 6y +20 0b) 5x2 +5y2 +8xy+2y – 2x+2 0c) 5x2 +2y2 + 4xy – 2x + 4y +5 0d) x2 + 4y2 + z2 2x + 12y – 4z – 14e) x2 +y2 – 6x + 4y +2 0 2) Phân tích đa thức thành nhân tửa) 3xy2 – 3x3 – 6xy +3xb) 3x2 + 11x + 6c) –x3 – 4xy2 + 4x2y +16xd) xz – x2 – yz +2xy – y2e) 4x2 – y2 – 6x + 3yf) X4 – x3 – 10x2 + 2x +4g) (x3 – x2 + x)(121 – 25y2 – 10y) – (x3 – x2 + x) – (121 – 25y2 – 10y) +1h) X4 – 14x3 + 71x2 – 154x + 120Giúp mik vs cần gấp!!!
Đọc tiếp
1) Tìm x, y, z
a) 9x2 +y2 + 2z2 – 18x +4z – 6y +20 = 0
b) 5x2 +5y2 +8xy+2y – 2x+2 = 0
c) 5x2 +2y2 + 4xy – 2x + 4y +5 = 0
d) x2 + 4y2 + z2 =2x + 12y – 4z – 14
e) x2 +y2 – 6x + 4y +2= 0
2) Phân tích đa thức thành nhân tử
a) 3xy2 – 3x3 – 6xy +3x
b) 3x2 + 11x + 6
c) –x3 – 4xy2 + 4x2y +16x
d) xz – x2 – yz +2xy – y2
e) 4x2 – y2 – 6x + 3y
f) X4 – x3 – 10x2 + 2x +4
g) (x3 – x2 + x)(121 – 25y2 – 10y) – (x3 – x2 + x) – (121 – 25y2 – 10y) +1
h) X4 – 14x3 + 71x2 – 154x + 120
Giúp mik vs cần gấp!!!
\(a,9x^2+y^2+2z^2-18x+4z-6y+20=0\\ \Leftrightarrow9\left(x-1\right)^2+\left(y-3\right)^2+2\left(z+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=3\\z=-1\end{matrix}\right.\)
\(b,5x^2+5y^2+8xy+2y-2x+2=0\\ \Leftrightarrow4\left(x+y\right)^2+\left(x-1\right)^2+\left(y+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=-y\\x=1\\y=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)
\(c,5x^2+2y^2+4xy-2x+4y+5=0\\ \Leftrightarrow\left(2x+y\right)^2+\left(x-1\right)^2+\left(y+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x=-y\\x=1\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)
\(d,x^2+4y^2+z^2=2x+12y-4z-14\\ \Leftrightarrow\left(x-1\right)^2+\left(2y-3\right)^2+\left(z+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\dfrac{3}{2}\\z=-2\end{matrix}\right.\)
\(e,x^2+y^2-6x+4y+2=0\\ \Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=11\)
Pt vô nghiệm do ko có 2 bình phương số nguyên có tổng là 11
Đúng 3
Bình luận (0)
e: Ta có: \(x^2-6x+y^2+4y+2=0\)
\(\Leftrightarrow x^2-6x+9+y^2+4y+4-11=0\)
\(\Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=11\)
Dấu '=' xảy ra khi x=3 và y=-2
Đúng 1
Bình luận (0)
1) Tìm x, y, za) 9x2 +y2 + 2z2 – 18x +4z – 6y +20 0b) 5x2 +5y2 +8xy+2y – 2x+2 0c) 5x2 +2y2 + 4xy – 2x + 4y +5 0d) x2 + 4y2 + z2 2x + 12y – 4z – 14e) x2 +y2 – 6x + 4y +2 0 2) Phân tích đa thức thành nhân tửa) 3xy2 – 3x3 – 6xy +3xb) 3x2 + 11x + 6c) –x3 – 4xy2 + 4x2y +16xd) xz – x2 – yz +2xy – y2e) 4x2 – y2 – 6x + 3yf) X4 – x3 – 10x2 + 2x +4g) (x3 – x2 + x)(121 – 25y2 – 10y) – (x3 – x2 + x) – (121 – 25y2 – 10y) +1h) X4 – 14x3 + 71x2 – 154x + 120Giúp mik với mik đang cần rất gấp ạ!!!
Đọc tiếp
1) Tìm x, y, z
a) 9x2 +y2 + 2z2 – 18x +4z – 6y +20 = 0
b) 5x2 +5y2 +8xy+2y – 2x+2 = 0
c) 5x2 +2y2 + 4xy – 2x + 4y +5 = 0
d) x2 + 4y2 + z2 =2x + 12y – 4z – 14
e) x2 +y2 – 6x + 4y +2= 0
2) Phân tích đa thức thành nhân tử
a) 3xy2 – 3x3 – 6xy +3x
b) 3x2 + 11x + 6
c) –x3 – 4xy2 + 4x2y +16x
d) xz – x2 – yz +2xy – y2
e) 4x2 – y2 – 6x + 3y
f) X4 – x3 – 10x2 + 2x +4
g) (x3 – x2 + x)(121 – 25y2 – 10y) – (x3 – x2 + x) – (121 – 25y2 – 10y) +1
h) X4 – 14x3 + 71x2 – 154x + 120
Giúp mik với mik đang cần rất gấp ạ!!!
Tìm x, y, z:
X2 + 2x + y2 - 6y + 4z2 - 4z + 11 = 0
\(\Leftrightarrow x^2+2x+1+y^2-6x+9+4z^2-4z+1=0\)
\(\Leftrightarrow\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2=0\)(1)
VT(1) >= 0 với mọi x;y;z nên để đẳng thức (1) xảy ra thì: x = -1; y = 3; z = 1/2.
Đúng 0
Bình luận (0)
1) Tìm x, y, z
a) 9x2 +y2 + 2z2 – 18x +4z – 6y +20 = 0
b) 5x2 +5y2 +8xy+2y – 2x+2 = 0
c) 5x2 +2y2 + 4xy – 2x + 4y +5 = 0
d) x2 + 4y2 + z2 =2x + 12y – 4z – 14
e) x2 +y2 – 6x + 4y +2= 0
Giúp mik vs cần gấp!!!
\(a,\Leftrightarrow\left(9x^2-18x+9\right)+\left(y^2-6y+9\right)+\left(2z^2+4z+2\right)=0\\ \Leftrightarrow9\left(x-1\right)^2+\left(y-3\right)^2+2\left(z+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=3\\z=-1\end{matrix}\right.\)
\(b,\Leftrightarrow\left(4x^2+8xy+4y^2\right)+\left(x^2-2x+1\right)+\left(y^2+2y+1\right)=0\\ \Leftrightarrow4\left(x+y\right)^2+\left(x-1\right)^2+\left(y+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=-y\\x=1\\y=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)
\(c,\Leftrightarrow\left(4x^2+4xy+y^2\right)+\left(x^2-2x+1\right)+\left(y^2+4y+4\right)=0\\ \Leftrightarrow\left(2x+y\right)^2+\left(x-1\right)^2+\left(y+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x=-y\\x=1\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)
Đúng 4
Bình luận (0)
a,9x^2+y^2+2z^2−18x+4z−6y+20=0
⇔9(x−1)^2+(y−3)^2+2(z+1)^2=0
⇔x=1;y=3;z=−1
b,5x^2+5y^2+8xy+2y−2x+2=0
⇔4(x+y)2+(x−1)2+(y+1)2=0
⇔x=−y;x=1y=−1⇔x=1y=−1
c,5x^2+2y^2+4xy−2x+4y+5=0
⇔(2x+y)^2+(x−1)^2+(y+2)^2=0
⇔2x=−y;x=1;y=−2
⇔x=1;y=−2
⇔(x−1)^2+(2y−3)^2+(z+2)^2=0
Đúng 2
Bình luận (0)
\(d,\Leftrightarrow\left(x^2-2x+1\right)+\left(4y^2-12y+9\right)+\left(z^2+4z+4\right)=0\\ \Leftrightarrow\left(x-1\right)^2+\left(2y-3\right)^2+\left(z+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\dfrac{3}{2}\\z=-2\end{matrix}\right.\)
\(e,x^2+y^2-6x+4y+2=0\\ \Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=11\)
\(\Rightarrow\)PT vô nghiệm vì 11 không phải là tổng 2 số chính phương
Đúng 2
Bình luận (0)
tìm x,y,z biết:
x2+2x+y2-6x+4z2-4z+11=0
x2 + 2x + y2 - 6y + 4z2 - 4z + 11 = 0
<=> ( x2 + 2x + 1 ) + ( y2 - 6y + 9 ) + ( 4z2 - 4z + 1 ) = 0
<=> ( x + 1 )2 + ( y - 3 )2 + ( 2z - 1 )2 = 0 (*)
Ta có : \(\hept{\begin{cases}\left(x+1\right)^2\ge0\forall x\\\left(y-3\right)^2\ge0\forall y\\\left(2z-1\right)^2\ge0\forall z\end{cases}}\Rightarrow\left(x+1\right)^2+\left(y-3\right)^2+\left(2z-1\right)^2\ge0\forall x,y,z\)
Dấu "=" xảy ra tức (*) <=> \(\hept{\begin{cases}x+1=0\\y-3=0\\2z-1=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=-1\\y=3\\z=\frac{1}{2}\end{cases}}\)
Vậy ...
Cho đường thẳng
∆
:
x
2
y
-
1
1
z
-
2
-
1
và và mặt cầu
(
S
)
:
x
2
+
y
2
+
z
2
-...
Đọc tiếp
Cho đường thẳng ∆ : x 2 = y - 1 1 = z - 2 - 1 và và mặt cầu ( S ) : x 2 + y 2 + z 2 - 2 x + 4 z + 1 = 0 . Số điểm chung của (Δ) và (S) là :
A. 0
B. 1
C. 2
D. 3
Chọn A.
Đường thẳng (Δ) đi qua M(0; 1; 2) và có một vectơ chỉ phương là 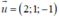
Mặt cầu (S) có tâm I(1; 0; -2) và bán kính ![]()
Ta có![]()
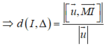
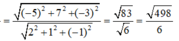
![]() không cắt mặt cầu (S)
không cắt mặt cầu (S)
Đúng 0
Bình luận (0)







