giải phương trình (x-2)2(x-9) = 0
1. giải phương trình tích:
a) \(\left(x+3\right)\left(x^2+2021\right)=0\)
\(\)2. giải các phương trình sau bằng cách đưa về phương trình tích:
b) \(x\left(x-3\right)+3\left(x-3\right)=0\)
c) \(\left(x^2-9\right)+\left(x+3\right)\left(3-2x\right)=0\)
d) \(3x^2+3x=0\)
e) \(x^2-4x+4=4\)
`a,(x+3)(x^2+2021)=0`
`x^2+2021>=2021>0`
`=>x+3=0`
`=>x=-3`
`2,x(x-3)+3(x-3)=0`
`=>(x-3)(x+3)=0`
`=>x=+-3`
`b,x^2-9+(x+3)(3-2x)=0`
`=>(x-3)(x+3)+(x+3)(3-2x)=0`
`=>(x+3)(-x)=0`
`=>` $\left[ \begin{array}{l}x=0\\x=-3\end{array} \right.$
`d,3x^2+3x=0`
`=>3x(x+1)=0`
`=>` $\left[ \begin{array}{l}x=0\\x=-1\end{array} \right.$
`e,x^2-4x+4=4`
`=>x^2-4x=0`
`=>x(x-4)=0`
`=>` $\left[ \begin{array}{l}x=0\\x=4\end{array} \right.$
1) a) \(\left(x+3\right).\left(x^2+2021\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+3=0\\x^2+2021=0\end{matrix}\right.\\\left[{}\begin{matrix}x=-3\left(nhận\right)\\x^2=-2021\left(loại\right)\end{matrix}\right. \)
=> S={-3}
Bài 1:
a) Ta có: \(\left(x+3\right)\left(x^2+2021\right)=0\)
mà \(x^2+2021>0\forall x\)
nên x+3=0
hay x=-3
Vậy: S={-3}
Bài 2:
b) Ta có: \(x\left(x-3\right)+3\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x+3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\)
Vậy: S={3;-3}
bài 9 các cặp phương trình sau có tương đương hay không?
d, x+2=0 và \(\dfrac{x}{x+2}=0\)
bài 8 cho phương trình (m\(^2\)-9)x-3=m. Giải phương trình trong các trường hợp sau:
a,m=2 b,m=3 c,m=-3
Bài 9:
Không, vì $x+2=0$ có nghiệm duy nhất $x=-2$ còn $\frac{x}{x+2}=0$ ngay từ đầu đkxđ đã là $x\neq -2$ (cả 2 pt không có cùng tập nghiệm)
Bài 8:
a. Khi $m=2$ thì pt trở thành:
$(2^2-9)x-3=2$
$\Leftrightarrow -5x-3=2$
$\Leftrightarrow -5x=5$
$\Leftrightarrow x=-1$
b.
Khi $m=3$ thì pt trở thành:
$(3^2-9)x-3=3$
$\Leftrightarrow 0x-3=3$
$\Leftrightarrow 0=6$ (vô lý)
c. Khi $m=3$ thì pt trở thành:
$[(-3)^2-9]x-3=-3$
$\Leftrightarrow 0x-3=-3$ (luôn đúng với mọi $x\in\mathbb{R}$)
Vậy pt vô số nghiệm thực.
a) \(2\left(x^2-2x\right)+\sqrt{x^2-2x-3}-9=0\)
b) \(3\sqrt{2+x}-6\sqrt{2-x}+4\sqrt{4-x^2}=10-3x\)
c) Cho phương trình: \(\sqrt{x}+\sqrt{9-x}=\sqrt{-x^2+9x+m}\)
+) Giải phương trình khi m=9
+) Tìm m để phương trình có nghiệm
a, ĐK: \(x\le-1,x\ge3\)
\(pt\Leftrightarrow2\left(x^2-2x-3\right)+\sqrt{x^2-2x-3}-3=0\)
\(\Leftrightarrow\left(2\sqrt{x^2-2x-3}+3\right).\left(\sqrt{x^2-2x-3}-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x^2-2x-3}=-\dfrac{3}{2}\left(l\right)\\\sqrt{x^2-2x-3}=1\end{matrix}\right.\)
\(\Leftrightarrow x^2-2x-3=1\)
\(\Leftrightarrow x^2-2x-4=0\)
\(\Leftrightarrow x=1\pm\sqrt{5}\left(tm\right)\)
b, ĐK: \(-2\le x\le2\)
Đặt \(\sqrt{2+x}-2\sqrt{2-x}=t\Rightarrow t^2=10-3x-4\sqrt{4-x^2}\)
Khi đó phương trình tương đương:
\(3t-t^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=0\\t=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{2+x}-2\sqrt{2-x}=0\\\sqrt{2+x}-2\sqrt{2-x}=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2+x=8-4x\\2+x=17-4x+12\sqrt{2-x}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{6}{5}\left(tm\right)\\5x-15=12\sqrt{2-x}\left(1\right)\end{matrix}\right.\)
Vì \(-2\le x\le2\Rightarrow5x-15< 0\Rightarrow\left(1\right)\) vô nghiệm
Vậy phương trình đã cho có nghiệm \(x=\dfrac{6}{5}\)
c, ĐK: \(0\le x\le9\)
Đặt \(\sqrt{9x-x^2}=t\left(0\le t\le\dfrac{9}{2}\right)\)
\(pt\Leftrightarrow9+2\sqrt{9x-x^2}=-x^2+9x+m\)
\(\Leftrightarrow-\left(-x^2+9x\right)+2\sqrt{9x-x^2}+9=m\)
\(\Leftrightarrow-t^2+2t+9=m\)
Khi \(m=9,pt\Leftrightarrow-t^2+2t=0\Leftrightarrow\left[{}\begin{matrix}t=0\\t=2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}9x-x^2=0\\9x-x^2=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=9\left(tm\right)\\x=\dfrac{9\pm\sqrt{65}}{2}\left(tm\right)\end{matrix}\right.\)
Phương trình đã cho có nghiệm khi phương trình \(m=f\left(t\right)=-t^2+2t+9\) có nghiệm
\(\Leftrightarrow minf\left(t\right)\le m\le maxf\left(t\right)\)
\(\Leftrightarrow-\dfrac{9}{4}\le m\le10\)
Giải phương trình sau
\(x^2-2\sqrt{5}x+5=0
\)
\(4x^4-5x^2-9=0\)
a: \(\Leftrightarrow\left(x-\sqrt{5}\right)^2=0\)
\(\Leftrightarrow x-\sqrt{5}=0\)
hay \(x=\sqrt{5}\)
b: \(\Leftrightarrow4x^4-9x^2+4x^2-9=0\)
\(\Leftrightarrow4x^2-9=0\)
=>x=3/2hoặc x=-3/2
Giải Phương trình
\(x-\dfrac{x+2}{3}< 3x+\dfrac{x}{2}+5
\)
\(\dfrac{x}{2}+\dfrac{1-x}{3}>0\)
\(\left(x-9\right)^2-x\left(x+9\right)< 0\)
a. \(x-\dfrac{x+2}{3}< 3x+\dfrac{x}{2}+5\)
\(\Leftrightarrow\dfrac{6x}{6}-\dfrac{2\left(x+2\right)}{6}< \dfrac{18x}{6}+\dfrac{3x}{6}+\dfrac{30}{6}\)
\(\Rightarrow6x-2x-4-18x-3x-30< 0\)
\(\Leftrightarrow-17x< 34\)
\(\Leftrightarrow x>-2\)
b. \(\dfrac{x}{2}+\dfrac{1-x}{3}>0\)
\(\Leftrightarrow3x+2-2x>0\)
\(\Leftrightarrow x>-2\)
c. \(\left(x-9\right)^2-x\left(x+9\right)< 0\)
\(\Leftrightarrow x^2-18x+81-x^2-9x< 0\)
\(\Leftrightarrow-27x< -81\)
\(\Leftrightarrow x>3\)
Bài tập 3: Giải phương trình.
a, \(\log_52x-\log_5-x-2=0\)
b, \(9^x-3.3^x+2=0\)
Bạn coi lại đề câu a, chỗ \(\log_5-x\) đó
b.
\(\Leftrightarrow9^x-3^x-2.3^x-2=0\)
\(\Leftrightarrow3^x\left(3^x-1\right)-2\left(3^x-1\right)=0\)
\(\Leftrightarrow\left(3^x-2\right)\left(3^x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}3^x=2\\3^x=1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\log_32\\x=0\end{matrix}\right.\)
Giải phương trình 9(x+2)-3x(x+2) = 0
\(\left(x+2\right)\left(9-3x\right)=0\)
\(3\left(x+2\right)\left(3-x\right)=0\)
\(\left(x+2\right)\left(3-x\right)=0\)
=> x+2 =0 hoặc 3-x=0
=> x=-2 hoặc x=3
Bài 1: Giải phương trình và bất phương trình sau: 1. 5.(2-3x). (x-2) = 3.( 1-3x) 2. 4x^2 + 4x + 1= 0 3. 4x^2 - 9= 0 4. 5x^2 - 10=0 5. x^2 - 3x= -2 6. |x-5| - 3= 0
Giúp e với ạ Giải bất phương trình: (x ^ 2 - 3x + 2)/(9 - x ^ 2) >0
\(\Leftrightarrow\dfrac{\left(x-1\right)\left(x-2\right)}{\left(3-x\right)\left(3+x\right)}>0\)
Bảng xét dấu:
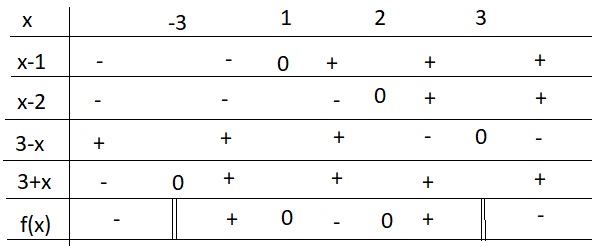
Từ bảng xét dấu ta có nghiệm của BPT là: \(x\in\left(-3;1\right)\cup\left(2;3\right)\)