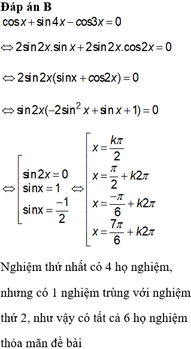Đáp án B

Nghiệm thứ nhất có 4 họ nghiệm , nhưng có 1 nghiệm trùng với nghiệm thứ 2, như vậy

có tất cả 6 họ nghiệm thỏa mãn đề bài

\(VT=\dfrac{2\cdot sin2x+2\cdot sin2x\cdot cos2x}{2\cdot\left(cosx+cos3x\right)}\)

\(=\dfrac{2\cdot sin2x\left(1+cos2x\right)}{2\cdot\left(cosx+cos3x\right)}\)

\(=\dfrac{sin2x\cdot\left(1+2cos^2x-1\right)}{cosx+cos3x}\)

\(=\dfrac{sin2x\cdot2\cdot cos^2x}{2\cdot cos\left(\dfrac{3x+x}{2}\right)\cdot cos\left(\dfrac{3x-x}{2}\right)}\)

\(=\dfrac{sin2x\cdot cos^2x}{cosx\cdot cos2x}=\dfrac{sin2x}{cos2x}\cdot cosx=tan2x\cdot cosx\)

\(sin4x+1-2sinx-sin2x-cos3x=0\)

\(\Leftrightarrow2cos3x.sinx-cos3x+1-2sinx=0\)

\(\Leftrightarrow cos3x\left(2sinx-1\right)-\left(2sinx-1\right)=0\)

\(\Leftrightarrow\left(cos3x-1\right)\left(2sinx-1\right)=0\Rightarrow\left[{}\begin{matrix}cos3x=1\\sinx=\dfrac{1}{2}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{k2\pi}{3}\\x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)

\(sin4x-2cos2x.cosx=0\)

\(\Leftrightarrow2sin2x.cos2x-2cos2x.cosx=0\)

\(\Leftrightarrow cos2x\left(sin2x-cosx\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\Rightarrow x=\frac{\pi}{4}+\frac{k\pi}{2}\\sin2x-cosx=0\left(1\right)\end{matrix}\right.\)

Xét (1)

\(\Leftrightarrow sin2x=cosx=sin\left(\frac{\pi}{2}-x\right)\)

\(\Rightarrow\left[{}\begin{matrix}2x=\frac{\pi}{2}-x+k2\pi\\2x=x-\frac{\pi}{2}+k2\pi\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{6}+\frac{k2\pi}{3}\\x=-\frac{\pi}{2}+k2\pi\end{matrix}\right.\)

\(\left(cosx+sin2x\right).sin2x=0\)

\(\Leftrightarrow\left(cosx+2sinx.cosx\right).2sinx.cosx=0\)

\(\Leftrightarrow\left(1+2sinx\right)sinx.cos^2x=0\)

\(\Leftrightarrow\left[{}\begin{matrix}1+2sinx=0\\sinx.cosx=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}sinx=-\frac{1}{2}\\sin2x=0\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=-\frac{\pi}{6}+k2\pi\\x=\frac{7\pi}{6}+k2\pi\\2x=k\pi\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=-\frac{\pi}{6}+k2\pi\\x=\frac{7\pi}{6}+k2\pi\\x=\frac{k\pi}{2}\end{matrix}\right.\)

\(cosx+cos3x+cos2x+cos4x=0\)

\(\Leftrightarrow2cos2x.cosx+2cos3x.cosx=0\)

\(\Leftrightarrow cosx\left(cos2x+cos3x\right)=0\)

\(\Leftrightarrow2cosx.cos\frac{5x}{2}.cos\frac{x}{2}=0\)

\(\Rightarrow\left[{}\begin{matrix}cosx=0\\cos\frac{x}{2}=0\\cos\frac{5x}{2}=0\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\\frac{x}{2}=\frac{\pi}{2}+k\pi\\\frac{5x}{2}=\frac{\pi}{2}+k\pi\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\x=\pi+k2\pi\\x=\frac{\pi}{5}+\frac{k2\pi}{5}\end{matrix}\right.\)

\(D=\frac{sin4x+sin5x+sin6x}{cos4x+cos5x+cos6x}\)

\(=\frac{\left(sin4x+sin6x\right)+sin5x}{\left(cos4x+cos6x\right)+cos5x}\)

\(=\frac{2sin\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+sin5x}{2cos\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+cos5x}\)

\(=\frac{2sin5x.cos\left(-x\right)+sin5x}{2cos5x.cos\left(-x\right)+cos5x}=\frac{sin5x\left(2.cos\left(-x\right)+1\right)}{cos5x\left(2.cos\left(-x\right)+1\right)}=\frac{sin5x}{cos5x}=tan5x\)

\(A=cosx+cos3x+cos2x=2cos2x.cosx+cos2x\)

\(=cos2x\left(2cosx+1\right)\)

\(B=sin3x+sin5x+sin4x=2sin4x.cosx+sin4x\)

\(=sin4x\left(2cosx+1\right)\)

\(A=\frac{cosx-cos3x+cos4x-cos2x}{sinx-sin3x+sin4x-sin2x}=\frac{2sin2x.sinx-2sin3x.sinx}{-2cos2x.sinx+2cos3x.sinx}\)

\(=\frac{sin2x-sin3x}{cos3x-cos2x}=\frac{-2cos\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}{-2sin\left(\frac{5x}{2}\right)sin\left(\frac{x}{2}\right)}=cot\left(\frac{5x}{2}\right)\)

\(B=sinx+2cos2x.sinx+2cos4x.sinx+2cos6x.sinx\)

\(=sinx+sin3x-sinx+sin5x-sin3x+sin7x-sin5x\)

\(=sin7x\)