Tập nghiệm của bất phương trình -x2 + 4x - 3 < 0 là:
A. \(\left(-\infty;1\right)\cup\left(3;+\infty\right)\) B. (1; 3) C. \(\text{∀}\text{x}\in\text{R}\) D. \(\left(-1;1\right)\)
FA
Những câu hỏi liên quan
Tập nghiệm của bất phương trình \(\dfrac{\text{x}^2-1}{x^2+x+1}>0\) là:
A. \(\left(1;+\infty\right)\) B. \(\left(-\infty;1\right)\) C. \(\left(-\infty;-1\right)\cup\left(1;+\infty\right)\) D. (-1; 1)
Xem thêm câu trả lời
Biểu diễn hình học miền nghiệm của bất phương trình, hệ bất phương trình sau
1) \(3x-y+1>0\) 2) \(2\left(x-1\right)+y-2\le x-3y+1\)
MS
8 tháng 3 2022 lúc 14:10
Biểu diễn hình học miền nghiệm của bất phương trình, hệ bất phương trình sau
1) 3x − y + 1 > 0 2) 2(x − 1) + y − 2 ≤ x − 3y + 1
Điều kiện xác định của bất phương trình \(\dfrac{\sqrt{\text{x}-2}}{x+1}-\sqrt{4-x}\ge0\) là:
A. \((-\infty;4]\backslash\left\{-1\right\}\) B. [2; +∞) C. \(\left[2;4\right]\) D. \([-1;4)\)
Xem thêm câu trả lời
Tìm tất cả giá trị của tham số m để bất phương trình -x2 + mx + m < 0 đúng với mọi x \(\in\) R
A. m < 0 B. \(\text{m}>-4\) C. \(-4< m< 0\) D. \(\text{m}< -4\cup m>0\)
Giải các bất phương trình sau
1) \(\sqrt{\text{x}^2+1}< 3\) 2) \(\dfrac{\text{x^2}-4x+3}{x^2-4}< 0\)
\(1)\sqrt{x^2+1}< 3.\\ \Leftrightarrow x^2+1< 9.\\ \Leftrightarrow x^2< 8.\\ \Leftrightarrow\left[{}\begin{matrix}x< 2\sqrt{2}.\\x>-2\sqrt{2}.\end{matrix}\right.\)
\(\Leftrightarrow-2\sqrt{2}< x< 2\sqrt{2}.\)
\(2)\dfrac{x^2-4x+3}{x^2-4}< 0.\)
Đặt \(f\left(x\right)=\dfrac{x^2-4x+3}{x^2-4}.\)
\(x^2-4=0.\Leftrightarrow\left[{}\begin{matrix}x=2.\\x=-2.\end{matrix}\right.\\ x^2-4x+3=0.\Leftrightarrow\left[{}\begin{matrix}x=3.\\x=1.\end{matrix}\right.\)
Bảng xét dấu:
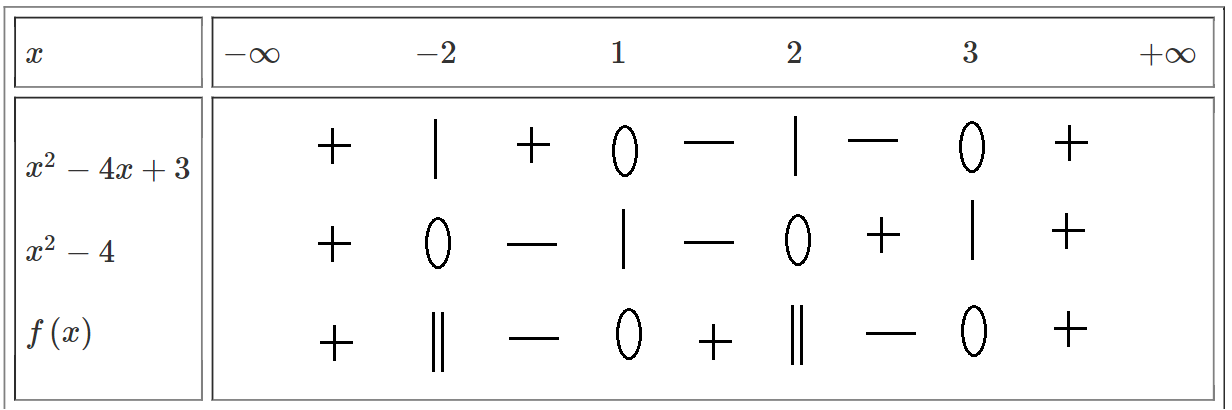
\(\Rightarrow f\left(x\right)< 0\Leftrightarrow x\in\left(-2;1\right)\cup\left(2;3\right).\)
Đúng 1
Bình luận (0)
Lời giải:
1.
$\sqrt{x^2+1}<3$
$\Leftrightarrow 0\leq x^2+1<9$
$\Leftrightarrow x^2+1<9$
$\Leftrightarrow x^2<8$
$\Leftrightarrow -2\sqrt{2}< x< 2\sqrt{2}$
2.
Xét 2 TH:
TH1: \(\left\{\begin{matrix} x^2-4x+3<0\\ x^2-4>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} (x-1)(x-3)<0\\ (x-2)(x+2)>0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} 1< x< 3\\ x>2 \text{hoặc} x<-2\end{matrix}\right.\)
\(\Leftrightarrow 2< x<3\)
TH2: \(\left\{\begin{matrix} x^2-4x+3>0\\ x^2-4<0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} (x-1)(x-3)>0\\ (x-2)(x+2)<0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x>3 \text{hoặc} x<1\\ -2< x< 2\end{matrix}\right.\)
\(\Leftrightarrow -2< x< 1\)
Kết hợp 2 TH suy ra tập nghiệm \(S=(2;3)\cup (-2;1)\)
Đúng 0
Bình luận (0)
Với giá trị nào của tham số m thì phương trình mx2 - 2mx + 1 = 0 có hai nghiệm dương phân biệt?
A. m > 0 B. 0 < m < 1 C. \(\text{m}\in\left(-\infty;2\right)\backslash\left\{0\right\}\) D. m > 1
\(\left\{{}\begin{matrix},m\ne0\\\Delta'>0\Leftrightarrow m^2-m>0\\x1+x2>0\Leftrightarrow2>0\\x1.x2>0\Leftrightarrow\dfrac{1}{m}>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m\ne0\\\left[{}\begin{matrix}m< 0\\m>1\end{matrix}\right.\\m>0\\\end{matrix}\right.\)\(\Leftrightarrow m>1\)
Đúng 0
Bình luận (0)
Bất phương trình: \(\sqrt{\text{-x^2 + 6x - 5}}>8-2x\) có nghiệm là:
A. 3 < x ≤ 5 B. 2 < x ≤ 3 C. -5 < x ≤ -3 D. -3 < x ≤ -2
Tìm tất cả các giá trị của tham số m để phương trình text{x}^2-left(2m-3right)x+m^2-3m0 có hai nghiệm x1, x2 phân biệt thoả mãn x1 x2 6A. text{m} 6 B. text{m}9 C. 6 m dfrac{15}{2} D. dfrac{15}{2} m 9
Đọc tiếp
Tìm tất cả các giá trị của tham số m để phương trình \(\text{x}^2-\left(2m-3\right)x+m^2-3m=0\) có hai nghiệm x1, x2 phân biệt thoả mãn x1 < x2 < 6
A. \(\text{m}< 6\) B. \(\text{m}>9\) C. \(6< m< \dfrac{15}{2}\) D. \(\dfrac{15}{2}< m< 9\)