Biết ∫ 0 π 2 x + x cos x - sin 3 x 1 + cos x d x = π 2 3 - b c Trong đó a, b, c là các số nguyên dương, phân số b/c tối giản. Tính T = a 2 + b 2 + c 2
A. T =16
B. T = 59
C. T =69
D. T = 50
Dựa vào đồ thị y = cos x trên [-π,π] hãy chỉ ra các khoảng giá trị x mà cos x >0 , cos x < 0
Tính:F=Cos(π/4+α) x cos(π/4-α)
G=Sin(π/3+α) x cos(π/3-α)
H=cos(π/2-α) x sin(π/2+α)
I=sin(π/4+α) - cos(π/4-α)
K=cos(π/6-x) - sin(π/3+x)
Tìm x biết ba số cos(x-π/4); sinx; cos(x+π/4) là 3 số hạng liên tiếp của cấp số nhân
Để \(cos\left(x-\dfrac{\Omega}{4}\right);sinx;cos\left(x+\dfrac{\Omega}{4}\right)\) là ba số hạng liên tiếp của cấp số nhân thì \(sin^2x=cos\left(x-\dfrac{\Omega}{4}\right)\cdot cos\left(x+\dfrac{\Omega}{4}\right)\)
=>\(sin^2x=\sqrt{2}\left(cosx-sinx\right)\cdot\sqrt{2}\left(cosx+sinx\right)\)
=>\(sin^2x=2cos^2x-2sin^2x\)
=>\(3\cdot sin^2x=2\cdot cos^2x\)
=>\(\dfrac{sin^2x}{cos^2x}=\dfrac{2}{3}\)
=>\(tan^2x=\dfrac{2}{3}\)
=>\(\left[{}\begin{matrix}tanx=\dfrac{\sqrt{6}}{3}\\tanx=-\dfrac{\sqrt{6}}{3}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=arctan\left(\dfrac{\sqrt{6}}{3}\right)+k\Omega\\x=arctan\left(-\dfrac{\sqrt{6}}{3}\right)+k\Omega\end{matrix}\right.\)
Trong các khoảng sau, m thuộc khoảng nào để phương trình sin^2 x-(2m+1) sin x.cos x + 2m cos^2 x = 0 có nghiệm thuộc khoảng (π/4 ; π/3)?
\(sin^2x-2m.sinx.cosx-sinx.cosx+2mcos^2x=0\)
\(\Leftrightarrow sinx\left(sinx-cosx\right)-2mcosx\left(sinx-cosx\right)=0\)
\(\Leftrightarrow\left(sinx-cosx\right)\left(sinx-2m.cosx\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}sinx=cosx\\sinx=2m.cosx\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}tanx=1\\tanx=2m\end{matrix}\right.\)
Do \(tanx=1\) ko có nghiệm đã cho nên \(tanx=2m\) phải có nghiệm trên khoảng đã cho
\(\Rightarrow tan\left(\dfrac{\pi}{4}\right)< 2m< tan\left(\dfrac{\pi}{3}\right)\)
\(\Rightarrow1< 2m< \sqrt[]{3}\)
\(\Rightarrow m\in\left(\dfrac{1}{2};\dfrac{\sqrt{3}}{2}\right)\) (hoặc có thể 1 đáp án là tập con của tập này cũng được)
\(\Leftrightarrow1-2sin^2x+\left(2m-3\right)sinx+m-2=0\)
\(\Leftrightarrow2sin^2x-\left(2m-3\right)sinx-m+1=0\)
\(\Leftrightarrow2sin^2x+sinx-2\left(m-1\right)sinx-\left(m-1\right)=0\)
\(\Leftrightarrow sinx\left(2sinx+1\right)-\left(m-1\right)\left(2sinx+1\right)=0\)
\(\Leftrightarrow\left(2sinx+1\right)\left(sinx-m+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=-\dfrac{1}{2}\\sinx=m-1\end{matrix}\right.\)
Pt có đúng 2 nghiệm thuộc khoảng đã cho khi và chỉ khi:
\(\left\{{}\begin{matrix}m-1\ne-\dfrac{1}{2}\\-1\le m-1\le1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m\ne\dfrac{1}{2}\\0\le m\le2\end{matrix}\right.\)
Số nghiệm của phương trình sin x . sin 2 x + 2 . sin x . cos 2 x + sin x + cos x sin x + cos x = 3 . cos 2 x trong khoảng - π , π là:
A. 2
B. 4
C. 3
D. 5
Sin(x-π/2)+cos(x-π)+tan(5π/2-x)+tan(x-π/2)=-2cosx
\(sin\left(x-\dfrac{\pi}{2}\right)+cos\left(x-\pi\right)+tan\left(\dfrac{5\pi}{2}-x\right)+tan\left(x-\dfrac{\pi}{2}\right)\)
\(=-sin\left(\dfrac{\pi}{2}-x\right)+cos\left(\pi-x\right)+tan\left(2\pi+\dfrac{\pi}{2}-x\right)-tan\left(\dfrac{\pi}{2}-x\right)\)
\(=-cosx-cosx+tan\left(\dfrac{\pi}{2}-x\right)-cotx\)
\(=-2cosx+cotx-cotx=-2cosx\)
Nếu \(cot1,25.tan\left(4\text{ }Π+1,25\right)-sin\left(x+\frac{Π}{2}\right).cos\left(6Π-x\right)=0\) thì tanx bằng
\(cot1,25.tan\left(4\pi+1,25\right)-sin\left(x+\frac{\pi}{2}\right).cos\left(6\pi-x\right)=0\)
\(\Leftrightarrow cot1,25.tan1,25-cosx.cos\left(-x\right)=0\)
\(\Leftrightarrow1-cos^2x=0\)
\(\Leftrightarrow sin^2x=0\Rightarrow sinx=0\Rightarrow tanx=0\)
Tìm nghiệm của phương trình lượng giác cos 2 x - cos x = 0 thỏa mãn điều kiện 0 < x < π
A. x = π 2
B. x=0
C. x= π
D. x=2
Đáp án A
Giải phương trình lượng giác sau đó kết hợp vào điều kiện của đầu bài để tìm ra nghiệm thỏa mãn.
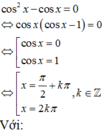
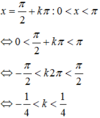
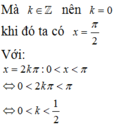
Mà k ∈ ℤ nên không có giá trị k nào thỏa mãn.
Sai lầm và chú ý: Đối với những bài toán giải phương trình lượng giác thỏa mãn điều kiện cho trước, ta cần tìm được x sau đó cho x thỏa mãn điều kiện đầu bài và cô lập được k khi đó ta sẽ tìm được giá trị nguyên k thỏa mãn và sẽ tìm đc x.