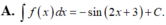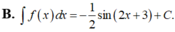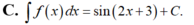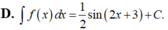Tìm họ nguyên hàm của hàm số sau: I = ∫ x + 1 3 - 2 x 3 d x
A. 3 4 3 - 2 x 7 3 7 - 5 3 + 2 x 4 3 4 + C
B. 3 4 3 - 2 x 7 3 - 7 - 5 3 - 2 x 4 3 4 + C
C. 3 4 3 - 2 x 7 3 7 - 5 3 - 2 x 4 3 - 4 + C
D. 3 4 3 - 2 x 7 3 7 - 5 3 - 2 x 4 3 4 + C
PB
Những câu hỏi liên quan
Tìm họ nguyên hàm của hàm số sau
∫
(
x
-
1
)
e
x
2
-
2
x
+
3
d
x
A.
1
2
e
x
2
-...
Đọc tiếp
Tìm họ nguyên hàm của hàm số sau ∫ ( x - 1 ) e x 2 - 2 x + 3 d x
A. 1 2 e x 2 - 2 x + 3 + C
B. - e x 2 - 2 x + 3 + C
C. 2 e x 2 - 2 x + 3 + C
D. x e x 2 - 2 x + 3 + C
Chọn A
Đặt u = x 2 - 2 x + 3 ⇒ d u = 2 ( x - 1 ) d x ⇒ ( x - 1 ) d x = d u 2
⇒ ∫ ( x - 1 ) e x 2 - 2 x + 3 d x = ∫ 1 2 e u d u = 1 2 e u + C = 1 2 e x 2 - 2 x + 3 + C
Đúng 0
Bình luận (0)
Tìm họ nguyên hàm của hàm số sau
I
∫
sin
3
x
cos
5
x
d
x
A.
sin
8
x
8
-
sin
6
x...
Đọc tiếp
Tìm họ nguyên hàm của hàm số sau I = ∫ sin 3 x cos 5 x d x
A. sin 8 x 8 - sin 6 x 6 + C
B. sin 8 x 7 - sin 6 x 5 + C
C. sin 8 x 9 - sin 6 x 7 + C
D. sin 8 x 8 + sin 6 x 6 + C
Chọn A
Đặt t = cos x ⇒ d t = - sin x d x
Ta có
I = ∫ 1 - cos 2 x cos 5 x sin x d x = - ∫ ( 1 - t 2 ) t 5 = ∫ ( t 7 - t 5 ) d t = t 8 8 - t 6 6 + C = sin 8 x 8 - sin 6 x 6 + C
Đúng 0
Bình luận (0)
Tìm 1 họ nguyên hàm của hàm số sau
K
∫
x
d
x
x
+
3
+
5
x
+
3
A.
1
-
6...
Đọc tiếp
Tìm 1 họ nguyên hàm của hàm số sau K = ∫ x d x x + 3 + 5 x + 3
A. 1 - 6 1 5 ( 5 x + 3 ) 3 - ( x + 3 ) 3
B. 1 6 1 5 ( 5 x + 3 ) 3 + ( x + 3 ) 3 + 1
C. 1 6 1 5 ( 5 x + 3 ) 3 - ( x + 3 ) 3 - 10
D. 1 6 1 - 5 ( 5 x + 3 ) 3 - ( x + 3 ) 3 + 8
Chọn C
Ta có
K = ∫ x ( 5 x + 3 - x + 3 ) d x 5 x + 3 - x - 3 = 1 4 ∫ 5 x + 3 - x + 3 d x = 1 6 1 5 ( 5 x + 3 ) 3 - ( x + 3 ) 3 + C
Cho C = -10.
Đúng 0
Bình luận (0)
Tìm họ nguyên hàm của hàm số sau
∫
x
2
+
1
.
x
d
x
A.
1
3
(
x
2
+
1
)
3
B. ...
Đọc tiếp
Tìm họ nguyên hàm của hàm số sau ∫ x 2 + 1 . x d x
A. 1 3 ( x 2 + 1 ) 3
B. 1 2 ( x 2 + 1 ) 3 + C
C. 1 3 ( x 2 + 1 ) 3 + C
D. 1 3 ( - x 2 + 1 ) 3 + C
Chọn C
Đặt u = x 2 + 1 ⇒ d u = 2 x d x ⇒ x d x = 1 2 d u
⇒ ∫ x 2 + 1 . x d x = ∫ u 1 2 . 1 2 d u = 1 2 ∫ u 1 2 d u = 1 2 u 3 2 . 2 3 = u 3 2 3 + C = 1 3 x 2 + 1 3 + C
Đúng 0
Bình luận (0)
Tìm họ nguyên hàm của hàm số sau
J
∫
cos
x
d
x
(
sin
x
+
2
cos
x
)
3
A.
J...
Đọc tiếp
Tìm họ nguyên hàm của hàm số sau J = ∫ cos x d x ( sin x + 2 cos x ) 3
A. J = 1 2 1 ( tan x + 2 ) 2 + C
B. J = - 1 2 1 ( tan x + 2 ) 2 + C
C. J = - 1 ( tan x + 2 ) 2 + C
D. J = - 1 2 1 ( tan 2 x + 2 ) 2 + C
Chọn B
I = ∫ cos x d x cos 3 x ( tan x + 2 ) 3 = ∫ d x cos 2 x ( tan x + 2 ) 3
Đặt t = tan x ⇒ d t = 1 cos 2 x d x
Do đó J = - 1 2 1 ( tan x + 2 ) 2 + C
Đúng 0
Bình luận (0)
Tìm họ nguyên hàm của hàm số lượng giác sau :
\(I=\int\tan x.\tan\left(x+\frac{\pi}{4}\right)dx\)
Ta biến đổi f(x) về dạng :
\(f\left(x\right)=\frac{\sin x.\sin\left(x+\frac{\pi}{4}\right)+\cos x.\cos\left(x+\frac{\pi}{4}\right)}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}-1=\frac{\cos\frac{\pi}{4}}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}-1\)
\(\Rightarrow F\left(x\right)=\frac{\sqrt{2}}{2}\int\frac{dx}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}dx-\int dx=\frac{\sqrt{2}}{2}\int\frac{dx}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}dx-x\left(1\right)\)
Để tính \(J=\int\frac{dx}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}dx\)
Ta có \(\int\frac{dx}{\cos x.\cos\left(x+\frac{\pi}{4}\right)}dx=\sqrt{2}\int\frac{1}{\cos x.\left(\cos x-\sin x\right)}dx=\sqrt{2}\int\frac{1}{\left(1-\tan x\right)}.\frac{1}{\cos^2x}dx\)
\(=-\sqrt{2}\int\frac{d\left(1-\tan x\right)}{1-\tan x}=\sqrt{2}\ln\left|1-\tan x\right|+C\)
Đúng 0
Bình luận (0)
H24
11 tháng 3 2022 lúc 21:18
Cho hàm số f(x) liên tục trên R. Biết x.ex là 1 nguyên hàm của f(x).e2x, tìm họ tất cả nguyên hàm của hàm số f'(x).e2x
Từ giả thiết: \(\int f\left(x\right).e^{2x}dx=x.e^x+C\)
Đạo hàm 2 vế:
\(\Rightarrow f\left(x\right).e^{2x}=e^x+x.e^x\)
\(\Rightarrow f\left(x\right)=\dfrac{e^x+x.e^x}{e^{2x}}=\dfrac{x+1}{e^x}\)
Xét \(I=\int f'\left(x\right)e^{2x}dx\)
Đặt \(\left\{{}\begin{matrix}u=e^{2x}\\dv=f'\left(x\right)\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=2.e^{2x}dx\\v=f\left(x\right)\end{matrix}\right.\)
\(\Rightarrow I=f\left(x\right).e^{2x}-2\int f\left(x\right).e^{2x}dx=\left(\dfrac{x+1}{e^x}\right)e^{2x}-2.x.e^x+C\)
\(=\left(1-x\right)e^x+C\)
Đúng 1
Bình luận (0)
Tìm họ nguyên hàm của hàm số
y
1
(
x
+
1
)
2
A.
∫
1
(...
Đọc tiếp
Tìm họ nguyên hàm của hàm số y = = 1 ( x + 1 ) 2
A. ∫ 1 ( x + 1 ) 2 d x = - 2 ( x + 1 ) 3 + C
B. ∫ 1 ( x + 1 ) 2 d x = - 1 x + 1 + C
C. ∫ 1 ( x + 1 ) 2 d x = 1 x + 1 + C
D. ∫ 1 ( x + 1 ) 2 d x = 2 ( x + 1 ) 3 + C
Đáp án B
Phương pháp: Sử dụng công thức ∫ 1 ( a x + b ) 2 = - 1 a ( a x + b ) + C
Cách giải: ∫ 1 ( x + 1 ) 2 d x = - 1 x + 1 + C
Đúng 0
Bình luận (0)
Tìm họ nguyên hàm của hàm số: f(x)=cos(2x+3)
