ĐKXĐ:\(x\ne\pm\dfrac{1}{2}\)

\(\dfrac{1+8x}{4+8x}-\dfrac{4x}{12x-6}+\dfrac{32x^2}{3\left(4-16x^2\right)}=0\)

\(\Leftrightarrow\dfrac{1+8x}{4\left(2x+1\right)}-\dfrac{4x}{6\left(2x-1\right)}+\dfrac{32x^2}{-6\cdot\left(2x-1\right)\left(2x+1\right)}=0\)

\(\Leftrightarrow\dfrac{6\cdot\left(1+8x\right)\left(2x-1\right)}{24\left(2x-1\right)\left(2x+1\right)}-\dfrac{4\cdot4x\left(2x+1\right)}{24\left(2x-1\right)\left(2x+1\right)}-\dfrac{32x^2\cdot4}{24\left(2x-1\right)\left(2x+1\right)}=0\)

\(\Leftrightarrow96x^2-36x-6-36x^2-16x-144x^2=0\)

\(\Leftrightarrow-84x^2-52x-6=0\)

\(\Leftrightarrow\Delta=688\)

\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{52-\sqrt{688}}{-168}=\dfrac{-13+\sqrt{43}}{42}\\x_2=\dfrac{52+\sqrt{688}}{-168}=\dfrac{-13-\sqrt{43}}{43}\end{matrix}\right.\)

Vậy pt có 2 nghiệm phân biệt............

Đáp án B

\(dat:\sqrt{x-5}=a\Rightarrow\sqrt{4\left(x-5\right)}+\sqrt{x-5}-\frac{1}{3}=\sqrt{9\left(x-5\right)}\Rightarrow\sqrt{4}.a+a-\frac{1}{3}=\sqrt{9}.a\Rightarrow3a-\frac{1}{3}=3a\left(voli\right)\Rightarrow vonghiem\)

câu a chắc đề như zầy pk bạn???

\(\sqrt{4x-20}+\sqrt{x-5}-\frac{1}{3}+\sqrt{9x-45}=4\)

\(pt\Leftrightarrow2\sqrt{x-5}+\sqrt{x-5}+3\sqrt{x-5}=\frac{13}{3}\)

\(\Leftrightarrow6\sqrt{x-5}=\frac{13}{3}\Rightarrow\sqrt{x-5}=\frac{13}{18}\Leftrightarrow x=\frac{1789}{324}\)

b)đề như này đúng ko bạn??

\(\sqrt{16-32x}-\sqrt{12x}=\sqrt{3x}+\sqrt{9-18x}\)

\(\Leftrightarrow4\sqrt{1-2x}-2\sqrt{3x}=\sqrt{3x}+3\sqrt{1-2x}\)

\(\Leftrightarrow\sqrt{1-2x}-3\sqrt{3x}=0\Leftrightarrow\sqrt{1-2x}=3\sqrt{3x}\)

\(\Leftrightarrow1-2x=27x\Leftrightarrow x=\frac{1}{29}\)

câu c\(\sqrt{4x-20}-3\sqrt{\frac{x-5}{9}}=\sqrt{1-x}\)

Xét điều kiện \(\left\{{}\begin{matrix}x\le1\\x\ge5\end{matrix}\right.\)không tồn tại số nào nằm trong khoảng này

Vậy pt trên vô nghiệm

\(\sqrt{16.\left(1-2x\right)}\) -\(\sqrt{4.3}\) =\(\sqrt{3x}\) +\(\sqrt{9.\left(1-2x\right)}\) 

(=)4\(\sqrt{1-2x}\) -2\(\sqrt{3x}\) =\(\sqrt{3x}\) +3\(\sqrt{1-2x}\) 

(=)\(\sqrt{1-2x}\) -3\(\sqrt{3x}\) =0

(=)1-2x=3\(\sqrt{3x}\) (=)1-2x=9.3x(=)1-2x=27x(=)29x=1(=)x=\(\frac{1}{29}\)

Ta có: Giá trị của biểu thức xác định khi mỗi giá trị của phân thức trong biểu thức đều được xác định.

Khi đó điều kiện xác định là: 2x - 2 ≠ 0; x 2 - 1 ≠ 0 ; 2x + 2 ≠ 0 hay x ≠ ± 1

Vậy với x ≠ ± 1 thì giá trị của biểu thức xác định.

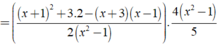
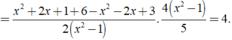
⇒ Giá trị của biểu thức A không phụ thuộc vào biến x

\(\Leftrightarrow A=\left(x^2-32x+240\right)^2+\left(x-12\right)^2+1870\)

\(\Leftrightarrow A\ge1870\)

\(\Rightarrow A_{min}=1870\) khi \(x^2-32x+240=0\) và \(x-12=0\)

\(\Leftrightarrow x=12\)

\(\left(12x-5\right)\left(4x-1\right)+\left(3x-7\right)\left(1-16x\right)=81\)

\(\text{⇔}48x^2-32x+5-48x^2-7+115x=81\)

\(\text{⇔}83x-2=81\)

\(\text{⇔}83x=83\)

\(\text{⇔}x=1\)

Vậy: x=1

Ta có: \(\left(12x-5\right)\left(4x-1\right)+\left(3x-7\right)\left(1-16x\right)=81\)

\(\Leftrightarrow48x^2-12x-20x+5+3x-48x^2-7+112x=81\)

\(\Leftrightarrow83x=83\)

hay x=1