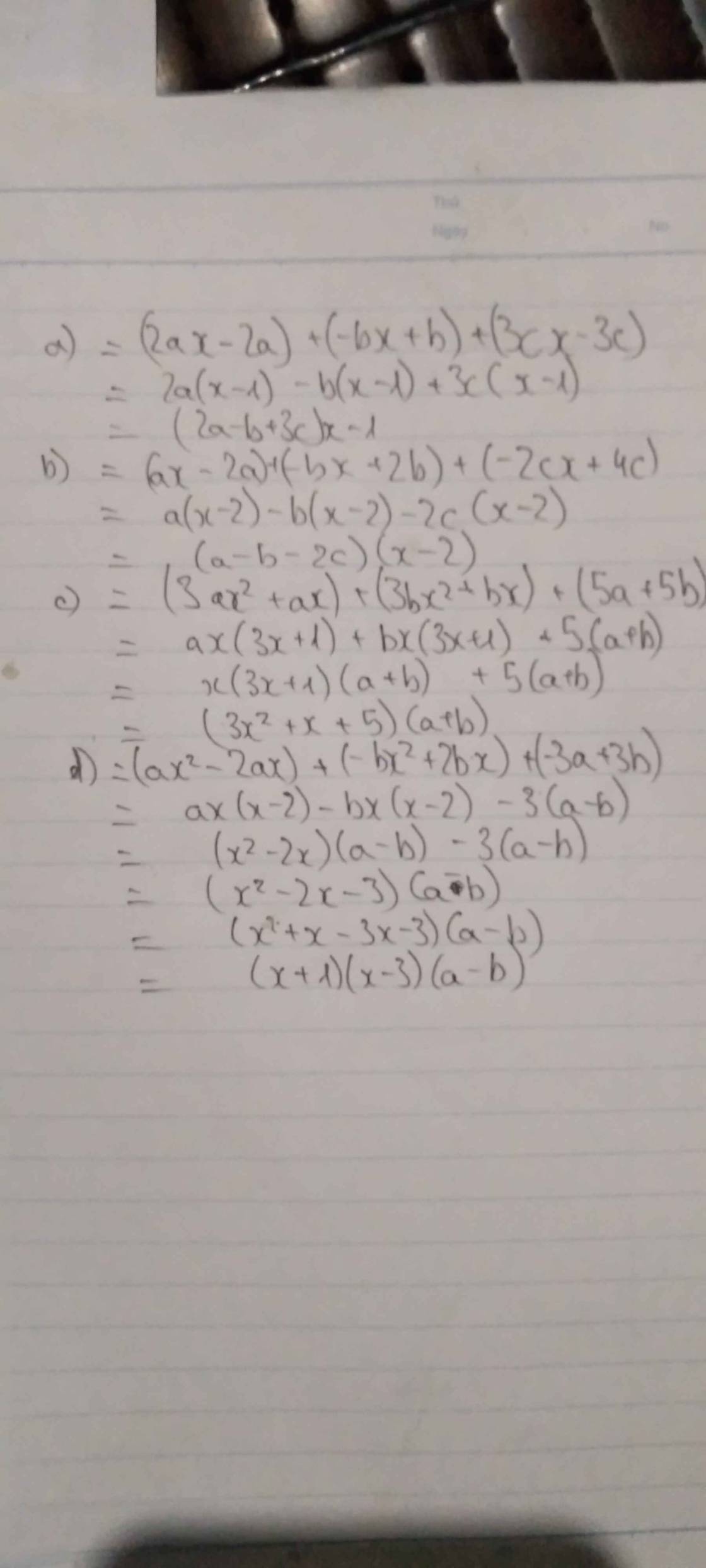\(A=\frac{ac+bx+ax+bc}{ay+2bx+2ax+by}CM\)ko phụ thuộc AB
BL
Những câu hỏi liên quan
Cho \(a\ne-b\) và biểu thức \(A=\frac{ac+bx+ax+bc}{ay+2bx+2ax+by}\)
CMR: Giá trị của biểu thức A không phụ thuộc vào a,b
\(A=\frac{ac+bx+ax+bc}{ay+2bx+2ax+by}=\frac{a\left(c+x\right)+b\left(c+x\right)}{a\left(y+2x\right)+b\left(y+2x\right)}=\frac{\left(c+x\right)\left(a+b\right)}{\left(y+2x\right)\left(a+b\right)}\)
Do \(a\ne-b\Rightarrow a+b\ne0\Rightarrow\)\(A=\frac{c+x}{y+2x}\), giá trị không phụ thuộc vào a; b (đpcm)
Đúng 0
Bình luận (0)
ac+bx+ax+bc
ay+2bx+2ax+by
Tính
a) A= ax-ay-bx+by biết a-b= 1: x-y= -2
b) B= 2ax+2ay-2bx-2by biết a-b= -2 : x+y= -5
Mk đang cần gấp, giúp mk nha
a) A = ax - ay - bx + by
A = a(x - y) - b(x - y)
A = (a - b)(x - y)
A = 1. (-2) = -2
B = 2ax + 2ay - 2bx - 2by
B = 2a(x + y) - 2b(x + y)
B = 2(x + y)(a - b)
B = 2. (-5).(-2) = 20
ab+ac
ab-ac+ad
ax-bx-cx-dx
a(b+c)-d(b+c)
ac-ad+bc-bd
ax+by+bx+ay
a) \(ab+ac=a.\left(b+c\right)\)
b) \(ab-ac+ad=a.\left(b-c+d\right)\)
c) \(ax-bx-cx-dx=x.\left(a-b-c-d\right)\)
d) \(a.\left(b+c\right)-d.\left(b+c\right)=ab+ac-db-dc=b.\left(a-d\right)+c.\left(a-d\right)=\left(a-d\right).\left(b+c\right)\)
e) \(ac-ad+bc-bd=a.\left(c-d\right)+b.\left(c-d\right)=\left(c-d\right).\left(a+b\right)\)
f) \(ax+by+bx+ay=a.\left(x+y\right)+b.\left(y+x\right)=\left(x+y\right).\left(a+b\right)\)
CHÚC BN HỌC TỐT!!!!!
Đúng 0
Bình luận (0)
Viết dưới dạng tích các tổng sau : ab+ac ; ab -ac+ad; ax -bx-cx+dx; a(b+c) - d (b+c); ac-ad+bc-bd; ax+by+bx+ay
ab + ac = a(b + c)
ab - ac + ad = a(b - c + d)
ax - bx - cx + dx
=x(a - b - c + d)
Đúng 0
Bình luận (0)
ab+ac=a(b+c)
ab-ac+ad=a(b-c+d)
ax-bx-cx+dx=x(a-b-c+d)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
ab+ac
ab-ac+ad
ax-bx-cx +dx
a(b+c) - d(b+c)
ac-ad+bc-bd
ax+by+bx+ay
ai đúng mình tick cho
,bố thằng nào làm đc
bạn đừng vào bình luận linh tinh
kh trả lời thì thôi
Xem thêm câu trả lời
Viết dưới dạng tích
a) ab + ac
b) ab - ac + ad
c) ax - bx - cx + dx
d) a(b + c) - d(b + c)
e) ac - ad + bc - bd
g) ax + by + bx +ay
a, \(ab+ac\)
\(=a\left(b+c\right)\)
b, \(ab-ac+ad\)
\(=a\left(b-c+d\right)\)
Đúng 0
Bình luận (0)
c, \(ax-bx-cx+dx\)
\(=x\left(a-b-c+d\right)\)
d, \(a\left(b+c\right)-d\left(b+c\right)\)
\(=\left(b+c\right)\left(a-d\right)\)
Đúng 0
Bình luận (0)
\(a)ab+ac=a\left(b+c\right)\)
\(b)ab-ac+ad=a\left(b-c+d\right)\)
\(c)ax-bx-cx+dx=x\left(a-b-c+d\right)\)
\(d)a\left(b+c\right)-d\left(b+c\right)=\left(b+c\right)\left(a-d\right)\)
\(e)ac-ad+bc-bd=a\left(c-d\right)+b\left(c-d\right)=\left(a+b\right)\left(c-d\right)\)
\(g)ax+by+bx+ay=\left(ax+bx\right)+\left(ay+by\right)=x\left(a+b\right)+y\left(a+b\right)=\left(x+y\right)\left(a+b\right)\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
a) ay+by+ax+bx
b)3xy-6y+2ax-4a
c)x^2
a) \(ay+by+ax+bx\)
\(=y\left(a+b\right)+x\left(a+b\right)\)
\(=\left(a+b\right)\left(x+y\right)\)
b) \(3xy-6y+2ax-4a\)
\(=3y\left(x-2\right)+2a\left(x-2\right)\)
\(=\left(x-2\right)\left(3y+2a\right)\)
c) sai đề ?
p/s: Trần Quỳnh Phương tên đẹp đấy nhưng lần sau nhớ viết đề cẩn thân hơn nhé!
Đúng 0
Bình luận (0)
Phân tích thành nhân tử (mọi người làm chi tiết ạ)
\(2ax-bx+3cx-2a+b-3c\)
\(ax-bx-2cx-2a+2b+4c\)
\(3ax^2 +3bx^2 +ax+bx+5a+5b\)
\(ax^2 -bx^2 -2ax+2bx-3a+3b\)
\(2ax-bx+3cx-2a+b-3c\\ =x\left(2a-b+3c\right)-\left(2a-b+3c\right)\\ =\left(x-1\right)\left(2a-b+3c\right)\)
\(ax-bx-2cx-2a+2b+4c\\ =x\left(a-b-2c\right)-2\left(a-b-2c\right)\\ =\left(x-2\right)\left(a-b-2c\right)\)
\(3ax^2+3bx^2+ax+bx+5a+5b\\ =3x^2\left(a+b\right)+x\left(a+b\right)+5\left(a+b\right)\\ =\left(3x^2+x+5\right)\left(a+b\right)\)
\(ax^2-bx^2-2ax+2bx-3a+3b\\ =x^2\left(a-b\right)-2x\left(a-b\right)-3\left(a+b\right)\\ =\left(x^2-2x-3\right)\left(a+b\right)\\ =\left(x+1\right)\left(x-3\right)\left(a+b\right)\)
Đúng 1
Bình luận (0)