Phương trình đối xứng với sinx và cosx
2cos2x + (sinxcosx-1) (sinx + cosx) = 0
2cos2x+ 4 cosx =1 +sinx- 2 sinxcosx
Giải phương trình hộ mình với
Giải phương trình đối xứng: cosx + sinx = 2sinx.cosx.(sinx + cosx)
=>(cosx+sinx)-2*sinx*cosx*(sinx+cosx)=0
=>\(\left(sinx+cosx\right)\left(2\cdot sinx\cdot cosx-1\right)=0\)
=>\(\sqrt{2}\cdot sin\left(x+\dfrac{pi}{4}\right)\cdot\left(sin2x-1\right)=0\)
=>\(\left[{}\begin{matrix}sin\left(x+\dfrac{pi}{4}\right)=0\\sin2x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{pi}{4}=kpi\\sin2x=1\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=kpi-\dfrac{pi}{4}\\2x=\dfrac{pi}{2}+k2pi\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=kpi-\dfrac{pi}{4}\\x=\dfrac{pi}{4}+kpi\end{matrix}\right.\)
Giải các phương trình sau: 1 + sin x - cos x - sin 2 x + 2 cos 2 x = 0
1 + sin x - cos x - sin 2 x + 2 cos 2 x = 0 ( 1 ) T a c ó : 1 - sin 2 x = sin x - cos x 2 ⇔ 2 cos 2 x = 2 ( cos 2 x - sin 2 x ) = - 2 ( sin x - cos x ) ( sin x + cos x ) V ậ y ( 1 ) ⇔ ( sin x - cos x ) ( 1 + sin x - cos x - 2 sin x - 2 cos x ) = 0 ⇔ ( sin x - cos x ) ( 1 - sin x - 3 cos x ) = 0
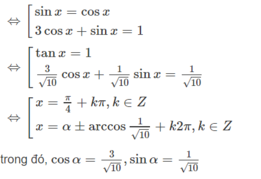
III. Phương trình bậc nhất đối với sinx và cosx:
*Giải các phương trình bậc nhất đối với sinx và cosx sau đây:
(2.1)
1) \(2sinx-2cosx=\sqrt{2}\)
2) \(cosx-\sqrt{3}sinx=1\)
3) \(\sqrt{3}sin\dfrac{x}{3}+cos\dfrac{x}{2}=\sqrt{2}\)
4) \(cosx-sinx=1\)
5) \(2cosx+2sinx=\sqrt{6}\)
6) \(sin3x+\sqrt{3}cosx=\sqrt{2}\)
7) \(3sinx-2cosx=2\)
(2.3)
1) \(\left(sinx-1\right)\left(1+cosx\right)=cos^2x\)
2) \(sin\left(\dfrac{\pi}{2}+2x\right)+\sqrt{3}sin\left(\pi-2x\right)=1\)
3) \(\sqrt{2}\left(cos^4x-sin^4x\right)=cosx+sinx\)
4) \(sin2x+cos2x=\sqrt{2}sin3x\)
5) \(sinx=\sqrt{2}sin5x-cosx\)
6) \(sin8x-cos6x=\sqrt{3}\left(sin6x+cos8x\right)\)
7) \(cos3x-sinx=\sqrt{3}\left(cosx-sin3x\right)\)
8) \(2sin^2x+\sqrt{3}sin2x=3\)
9) \(sin^4x+cos^4\left(x+\dfrac{\pi}{4}\right)=\dfrac{1}{4}\)
(2.3)
1) \(\dfrac{\sqrt{3}\left(1-cos2x\right)}{2sinx}=cosx\)
2) \(cotx-tanx=\dfrac{cosx-sinx}{sinx.cosx}\)
3) \(\dfrac{\sqrt{3}}{cosx}+\dfrac{1}{sinx}=4\)
4) \(\dfrac{1+sinx}{1+cosx}=\dfrac{1}{2}\)
5) \(3cosx+4sinx+\dfrac{6}{3cosx+4sinx+1}=6\)
(2.4)
a) Tìm nghiệm \(x\in\left(\dfrac{2\pi}{5};\dfrac{6\pi}{7}\right)\) của phương trình \(cos7x-\sqrt{3}sin7x+\sqrt{2}=0\)
b) Tìm nghiệm \(x\in\left(0;\pi\right)\) của phương trình \(4sin^2\dfrac{x}{2}-\sqrt{3}cos2x=1+2cos^2\left(x-\dfrac{3\pi}{4}\right)\)
(2.5) Xác định tham số m để các phương trình sau đây có nghiệm:
a) \(mcosx-\left(m+1\right)sinx=m\)
b) \(\left(2m-1\right)sinx+\left(m-1\right)cosx=m-3\)
(2.6) Tìm GTLN, GTNN (nếu có) của các hàm số sau đây:
a) \(y=3sinx-4cosx+5\)
b) \(y=cos2x+sin2x-1\)
2.1
a.
\(\Leftrightarrow sinx-cosx=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\sqrt{2}sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{4}=\dfrac{\pi}{6}+k2\pi\\x-\dfrac{\pi}{4}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5\pi}{12}+k2\pi\\x=\dfrac{13\pi}{12}+k2\pi\end{matrix}\right.\)
b.
\(cosx-\sqrt{3}sinx=1\)
\(\Leftrightarrow\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\x+\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
c.
\(\sqrt{3}sin\dfrac{x}{3}+cos\dfrac{x}{2}=\sqrt{2}\)
Câu này đề đúng không nhỉ? Nhìn thấy có vẻ không đúng lắm
d.
\(cosx-sinx=1\)
\(\Leftrightarrow\sqrt{2}cos\left(x+\dfrac{\pi}{4}\right)=1\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{4}=\dfrac{\pi}{4}+k2\pi\\x+\dfrac{\pi}{4}=-\dfrac{\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)
\(1.\left(sinx+cosx\right)^3+sinxcosx-1=0\)
\(2.\left(sinx+cosx\right)^4-3sin2x-1=0\)
\(3.sin^3x+cos^3x+2\left(sinx+cosx\right)-3sin2x=0\)
\(4.\left(sinx-cosx\right)^3=1+sinxcosx\)
5.\(sinx+cosx+2+tanx+cotx+\frac{1}{sinx}+\frac{1}{cosx}=0\)
1.
Đặt \(sinx+cosx=t\Rightarrow\left\{{}\begin{matrix}\left|t\right|\le\sqrt{2}\\sinx.cosx=\frac{t^2-1}{2}\end{matrix}\right.\)
Pt trở thành:
\(t^3+\frac{t^2-1}{2}-1=0\)
\(\Leftrightarrow2t^3+t^2-3=0\)
\(\Leftrightarrow\left(t-1\right)\left(2t^2+3t+3\right)=0\)
\(\Leftrightarrow t=1\)
\(\Leftrightarrow sin\left(x+\frac{\pi}{4}\right)=\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\frac{\pi}{4}=\frac{\pi}{4}+k2\pi\\x+\frac{\pi}{4}=\frac{3\pi}{4}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow...\)
b.
Đặt \(sinx+cosx=t\Rightarrow\left\{{}\begin{matrix}\left|t\right|\le\sqrt{2}\\sin2x=2sinx.cosx=t^2-1\end{matrix}\right.\)
Pt trở thành:
\(t^4-3\left(t^2-1\right)-1=0\)
\(\Leftrightarrow t^4-3t^2+2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t^2=1\\t^2=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}1+sin2x=1\\1+sin2x=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sin2x=0\\sin2x=1\end{matrix}\right.\)
\(\Leftrightarrow...\)
3.
\(\Leftrightarrow\left(sinx+cosx\right)\left(1-sinx.cosx\right)+2\left(sinx+cosx\right)-6sinx.cosx=0\)
Đặt \(sinx+cosx=t\Rightarrow\left\{{}\begin{matrix}\left|t\right|\le\sqrt{2}\\sinx.cosx=\frac{t^2-1}{2}\end{matrix}\right.\)
Pt trở thành:
\(t\left(1-\frac{t^2-1}{2}\right)+2t-3\left(t^2-1\right)=0\)
\(\Leftrightarrow-t^3-6t^2+7t+6=0\)
Nghiệm của pt bậc 3 này rất xấu, chắc bạn ghi ko đúng đề bài
Nghiệm của phương trình 2 ( sin x + cos x ) + sin x cos x = 2 là:
A. π 2 + k 2 π , k ∈ Z hoặc k 2 π , k ∈ Z
B. π 2 + k π , k ∈ Z hoặc kπ , k ∈ Z
C. - π 2 + k 2 π , k ∈ Z hoặc k 2 π , k ∈ Z
D. - π 2 + k π , k ∈ Z hoặc kπ , k ∈ Z
Số điểm biểu diễn nghiệm của phương trình cos 2 x + sinxcosx + cosx − sinx = 0 trên đường tròn lượng giác là
A. 4
B. 2
C. 1
D. 3
Đáp án C
Nhận thấy cos x = 0 không phải là nghiệm của phương trình. Chia cả hai vế của phương trình cho cosx ta được
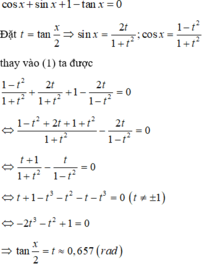
Vậy có 1 điểm biểu diễn nghiệm của phương trình trên đường tròn lượng giác.
Số điểm biểu diễn nghiệm của phương trình cos 2 x + sin x cos x + cos x - sin x = 0 trên đường tròn lượng giác là
A. 4
B. 2
C. 1
D. 3
Số điểm biểu diễn nghiệm của phương trình cos 2 x + sin x cos x + cos x - sin x = 0 trên đường tròn lượng giác là
A. 4
B. 2
C. 1
D. 3
Đáp án C
Nhận thấy cosx = 0 không phải là nghiệm của phương trình. Chia cả hai vế của phương trình cho cosx ta được
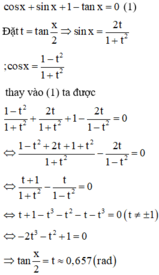
Vậy có 1 điểm biểu diễn nghiệm của phương trình trên đường tròn lượng giác.