Giải phương trình :
\(\sqrt{3x+1}\)= -4x2 +13x -5
`4x=2+xx+1x<=>4x=2+3x<=>4x-3x=2<=>1x=2<=>x=2`
Giải phương trình : \(\sqrt{x+1}\) - 4x2 = \(\sqrt{3x}\) -1
\(\sqrt{x+1}-4x^2=\sqrt{3x}-1\left(x\ge0\right)\left(1\right)\)
\(\Leftrightarrow-4x^2+1+\sqrt{x+1}-\dfrac{\sqrt{6}}{2}=\sqrt{3x}-\dfrac{\sqrt{6}}{2}\)
\(\Leftrightarrow-\left(2x-1\right)\left(2x+1\right)+\dfrac{x+1-\dfrac{3}{2}}{\sqrt{x+1}+\dfrac{\sqrt{6}}{2}}=\dfrac{3x-\dfrac{3}{2}}{\sqrt{3x}+\dfrac{\sqrt{6}}{2}}\)
\(\Leftrightarrow-4\left(x-\dfrac{1}{2}\right)\left(x+\dfrac{1}{2}\right)+\dfrac{x-\dfrac{1}{2}}{\sqrt{x+1}+\dfrac{\sqrt{6}}{2}}-\dfrac{3\left(x-\dfrac{1}{2}\right)}{\sqrt{3x}+\dfrac{\sqrt{6}}{2}}=0\)
\(\Leftrightarrow\left(x-\dfrac{1}{2}\right)\left[-4\left(x+\dfrac{1}{2}\right)+\dfrac{1}{\sqrt{x+1}+\dfrac{\sqrt{6}}{2}}-\dfrac{3}{\sqrt{3x}+\dfrac{\sqrt{6}}{2}}\right]=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\left(tm\right)\\-4\left(x+\dfrac{1}{2}\right)+\dfrac{1}{\sqrt{x+1}+\dfrac{\sqrt{6}}{2}}-\dfrac{3}{\sqrt{3x}+\dfrac{\sqrt{6}}{2}}=0\left(2\right)\end{matrix}\right.\)
\(\left(x\ge0\right)\Rightarrow\left(2\right)< 0\Rightarrow\left(2\right)vô\) \(nghiệm\)
\(\Rightarrow S=\left\{\dfrac{1}{2}\right\}\)
\(\)
giải phương trình :
a,\(\sqrt{2x^2+13x+5}+\sqrt{2x^2-3x+5}=8\sqrt{x}\)
b, \(\sqrt{x^2-\dfrac{4}{3}}+2\sqrt{x^2-1}=x\)
a.
ĐKXĐ: \(x\ge0\)
\(\sqrt{2x^2+13x+5}-5\sqrt{x}+\sqrt{2x^2-3x+5}-3\sqrt{x}=0\)
\(\Leftrightarrow\dfrac{2x^2-12x+5}{\sqrt{2x^2+13x+5}+5\sqrt{x}}+\dfrac{2x^2-12x+5}{\sqrt{2x^2-3x+5}+3\sqrt{x}}=0\)
\(\Leftrightarrow\left(2x^2-12x+5\right)\left(\dfrac{1}{\sqrt{2x^2+13x+5}+5\sqrt{x}}+\dfrac{1}{\sqrt{2x^2-3x+5}+3\sqrt{x}}\right)=0\)
\(\Leftrightarrow2x^2-12x+5=0\)
\(\Leftrightarrow...\)
b.
ĐKXĐ: \(x^2\ge\dfrac{4}{3}\)
\(\sqrt{x^2-\dfrac{4}{3}}+\sqrt{4x^2-4}-x=0\)
\(\Leftrightarrow\sqrt{\dfrac{3x^2-4}{3}}+\dfrac{3x^2-4}{\sqrt{4x^2-4}+x}=0\)
\(\Leftrightarrow\sqrt{3x^2-4}\left(\dfrac{1}{\sqrt{3}}+\dfrac{\sqrt{3x^2-4}}{\sqrt{4x^2-4}+x}\right)=0\)
\(\Leftrightarrow3x^2-4=0\)
\(\Leftrightarrow...\)
giải phương trình vô tỉ sau
\(4x^2+\sqrt{3x+1}+5=13x\)
\(\sqrt[3]{3x-5}=8x^3-36x^2+53x-25\)
đặt \(\sqrt{3x+1}=a\)
=> pt <=> 4x^2 +a +6=a^2 +12x
chuyển hết nt sang vế phải để vt =0 ptđttnt có ntc=a+2x-3
câu 2 đặt \(\sqrt[3]{3x-5}=2y-3\) rồi làm tt như bài trên lớp
sau khi chuyển cậu có pt a62-4x^2-a+12x-6=0
=> a^2+2ax-3a-2ax-4x^2+6x+2a+4x-6=0
<=> (a+2x-3)(a-2x+2)=0
c2 đăt...
=>3x-5=(2y-3)^3
mặt khác từ pt =>\(\sqrt[3]{3x-5}=\left(2x-3\right)^3-x+2\)
=>2y-3=(2x-3)^3-x+2
=>2y+x-5=(2x-3)^3 rồi cậu giải tt bài trên lớp
Giải phương trình sau bằng cách đưa về phương trình tích: 4x2 – 1 = (2x + 1)(3x – 5)
4x2 – 1 = (2x + 1)(3x – 5)
⇔ 4x2 – 1 – (2x + 1)(3x – 5) = 0
⇔ (2x – 1)(2x + 1) – (2x + 1)(3x – 5) = 0
⇔ (2x + 1)[(2x – 1) – (3x – 5)] = 0
⇔ (2x + 1)(2x – 1 – 3x + 5) = 0
⇔ (2x + 1)(4 – x) = 0
⇔ 2x + 1= 0 hoặc 4 – x = 0
+ 2x + 1 = 0 ⇔ 2x = -1 ⇔ x = -1/2.
+ 4 – x = 0 ⇔ x = 4.
Vậy phương trình có tập nghiệm 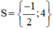
Giải phương trình √(3x+1) = -4x^2 +13x - 5
Giải phương trình: \(8x^3-13x+7=\left(x+1\right)\sqrt[3]{3x^2-2}\)
Ta viết lại phương trình thành:
\(\left(2x-1\right)^3-\left(x^2-x-1\right)=\left(x+1\right)\sqrt[3]{\left(x+1\right)\left(2x-1\right)+x^2-x-1}\)
Đặt: \(a=2x-1;b=\sqrt[3]{\left(x+1\right)\left(2x-1\right)+x^2-x-1}=\sqrt[3]{3x^2-2}\) ta thu được hệ phương trình:
\(\hept{\begin{cases}a^3-\left(x^2-x+1\right)=\left(x+1\right)b\\b^3-\left(x^2-x+1\right)=\left(x+1\right)a\end{cases}}\)
Trừ 2 pt của hệ cho nhau ta được: \(\left(a-b\right)\left(a^2+ab+b^2+x+1\right)=0\)
Trường hợp 1: \(a=b\) ta có:
\(2x-1=\sqrt[3]{3x^2-2}\Leftrightarrow8x^3-15x^2+6x+1=0\Leftrightarrow\orbr{\begin{cases}x=1\\x=-\frac{1}{8}\end{cases}}\)
Trường hợp 2: \(a^2+ab+b^2+x+1=0\Leftrightarrow\left(a+\frac{b}{2}\right)^2+\frac{3}{4}\left(2x-1\right)^2+x+1=0\)
\(\Leftrightarrow4\left(a+\frac{b}{2}\right)^2+4x^2+2\left(2x-1\right)^2+5=0\left(vn\right)\)
Vậy pt có 2 nghiệm là: \(x=1;x=-\frac{1}{8}\)
Chỗ nào z bn?
giải pt:
a, \(2x^2-6x-1=\sqrt{4x+5}\)
b, \(18x^2+6x-29=\sqrt{12x+61}\)
c, \(4x^2-13x+5+\sqrt{3x+1}=0\)
c, \(4x^2-13x+5+\sqrt{3x+1}=0\)
c.
ĐLXĐ: \(x\ge-\dfrac{1}{3}\)
\(-\left(3x+1\right)+\sqrt{3x+1}+4x^2-10x+6=0\)
Đặt \(\sqrt{3x+1}=t\ge0\)
\(\Rightarrow-t^2+t+4x^2-10x+6=0\)
\(\Delta=1+4\left(4x^2-10x+6\right)=\left(4x-5\right)^2\)
\(\Rightarrow\left[{}\begin{matrix}t=\dfrac{-1+4x-5}{-2}=3-2x\\t=\dfrac{-1-4x+5}{-2}=2x-2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{3x+1}=3-2x\left(x\le\dfrac{3}{2}\right)\\\sqrt{3x-1}=2x-2\left(x\ge1\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x+1=4x^2-12x+9\left(x\le\dfrac{3}{2}\right)\\3x-1=4x^2-8x+4\left(x\ge1\right)\end{matrix}\right.\)
\(\Leftrightarrow...\)
b.
ĐKXĐ: \(x\ge-\dfrac{61}{12}\)
\(\Leftrightarrow36x^2+12x-58-2\sqrt{12x+61}=0\)
\(\Leftrightarrow\left(36x^2+24x+4\right)-\left(12x+61+2\sqrt{12x+61}+1\right)=0\)
\(\Leftrightarrow\left(6x+2\right)^2-\left(\sqrt{12x+61}+1\right)^2=0\)
\(\Leftrightarrow\left(6x+1-\sqrt{12x+61}\right)\left(6x+3+\sqrt{12x+61}\right)=0\)
\(\Leftrightarrow...\) tương tự câu a
a.
ĐKXĐ: \(x\ge-\dfrac{5}{4}\)
\(\Leftrightarrow4x^2-12x-2-2\sqrt{4x+5}=0\)
\(\Leftrightarrow\left(4x^2-8x+4\right)-\left(4x+5+2\sqrt{4x+5}+1\right)=0\)
\(\Leftrightarrow\left(2x-2\right)^2-\left(\sqrt{4x+5}+1\right)^2=0\)
\(\Leftrightarrow\left(2x-2-\sqrt{4x+5}-1\right)\left(2x-2+\sqrt{4x+5}+1\right)=0\)
\(\Leftrightarrow\left(2x-3-\sqrt{4x+5}\right)\left(2x-1+\sqrt{4x+5}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{4x+5}=2x-3\left(x\ge\dfrac{3}{2}\right)\\\sqrt{4x+5}=1-2x\left(x\le\dfrac{1}{2}\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x+5=4x^2-12x+9\left(x\ge\dfrac{3}{2}\right)\\4x+5=4x^2-4x+1\left(x\le\dfrac{1}{2}\right)\end{matrix}\right.\)
\(\Leftrightarrow...\)
Giải các bất phương trình sau:
a) 2 x − 8 > 0 ; b) 9 − 3 x ≤ 0 ;
c) 5 − 1 3 x < 1 ; d) 3 x + 5 2 − x ≥ 1 + x + 2 3
Giải phương trình
a) x2-\(4\sqrt{15}\)x+19=0
b) 4x2+4\(\sqrt{5}\)x+5=0
a)
\(x^2-4\sqrt{15}x+19=0\\ < =>x^2-4\sqrt{15}x+60-41=0\\ < =>\left(x-2\sqrt{15}\right)^2-41=0\\ < =>\left(x-2\sqrt{15}-\sqrt{41}\right)\left(x-2\sqrt{15}+\sqrt{41}\right)=0\\ < =>\left[{}\begin{matrix}x-2\sqrt{15}-\sqrt{41}=0\\x-2\sqrt{15}+\sqrt{41}=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=2\sqrt{15}+\sqrt{41}\\x=2\sqrt{15}-\sqrt{41}\end{matrix}\right.\)
b)
\(4x^2+4\sqrt{5}x+5=0\\ < =>\left(2x+\sqrt{5}\right)^2=0\\ < =>2x+\sqrt{5}=0\\ < =>2x=-\sqrt{5}\\ < =>-\dfrac{\sqrt{5}}{2}\)
a: Δ=(4căn 15)^2-4*1*19=164>0
Phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x=\dfrac{4\sqrt{5}-2\sqrt{41}}{2}=2\sqrt{5}-\sqrt{41}\\x_2=2\sqrt{5}+\sqrt{41}\end{matrix}\right.\)
b: \(\Leftrightarrow\left(2x\right)^2+2\cdot2x\cdot\sqrt{5}+5=0\)
=>(2x+căn 5)^2=0
=>2x+căn 5=0
=>x=-1/2*căn 5