cho x, y, z \(\in\)R thỏa mãn x+y+z=6. tìm GTLN của biểu thức A=xy+2yz+3zx
MÌNH CẦN GẤP Ạ!!!
Cho các số thực x, y, z thỏa mãn: x + y + z = 6. Tìm GTLN của
A= xy +2yz +3xz
Đúng thì like giúp mik nha. Thx bạn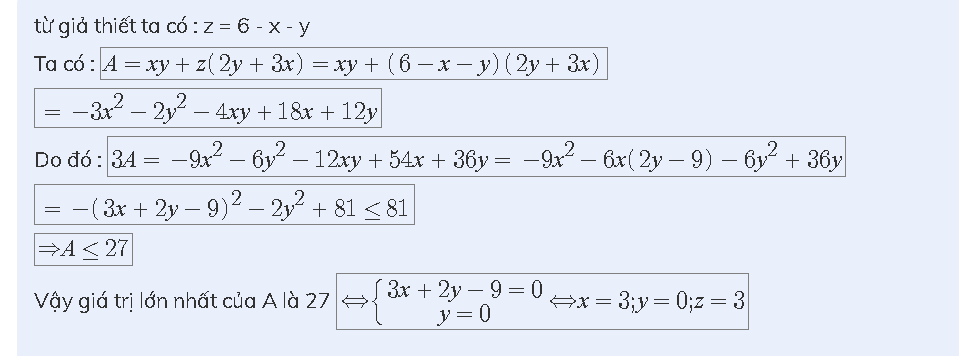
\(A=xy+xz+2yz+2xz=x\left(y+z\right)+2z\left(x+y\right)\)
\(=x\left(6-x\right)+2z\left(6-z\right)=-x^2+6x+2\left(-z^2+6z\right)\)
\(=-\left(x-3\right)^2-2\left(z-3\right)^2+27\le27\)
\(A_{max}=27\) khi \(\left(x;y;z\right)=\left(3;0;3\right)\)
Cho x,y,z là các số thực không âm thỏa mãn x+y+z=1. Tìm GTLN của biểu thức A = -z^2+z(y+1)+xy
cho x,y,z thỏa mãn x+y+z=6. tìm giá trị lớn nhất của A=xy+2yz+3zx
A = xy + 2yz + 3xz = xy + xz + 2yz + 2xz = x(y + z) + 2z(y + z)
Áp dụng BĐT: (a+b)^2/4 ≥ ab dấu = khi a = b
ta có:
(x + y + z)^2/4 ≥ x(y + z)
(x+ y +z)^2/4 ≥ z(y + z)
=> A ≤ 3(x + y + z)^2/4 = 3.36/4 = 27
=>Giá trị lớn nhất của = 27 sẽ xảy ra khi có các trường hợp:
{x = y + z
{z = y + z
Vậy y = 0 và x = z = 3
\(A=xy+2yz+3zx=x\left(6-x-z\right)+2\left(6-x-z\right)+3zx\)
\(=-x^2+6x-2z^2+12z=\left(-x^2+6x-9\right)+\left(-2z^2+12z-18\right)+27\)
\(=27-\left(x-3\right)^2-2\left(z-3\right)^2\le27\)
\(A=x\left(6-x-z\right)+2y\left(6-x-z\right)+3zx=-x^2+6x-2z^2+12z\)
\(=\left(-x^2+6x-9\right)+\left(-2z^2+12z-18\right)+27=27-\left(x-3\right)^2-2\left(z-3\right)^2\le27\)
PS: Cai trên ghi thiêu chữ y. Mà thôi coi cai này nè nha.
cho \(x,y,z\ge0\) thỏa mãn \(x+y+z=6\). tìm GTLN và GTNN của biểu thức \(A=x^2+y^2+z^2\)
Lời giải:
Tìm min:
Áp dụng BĐT AM-GM:
$x^2+y^2+z^2\geq \frac{(x+y+z)^2}{3}=\frac{6^2}{3}=12$
Vậy $A_{\min}=12$. Giá trị này đạt tại $x=y=z=2$
--------------
Tìm max:
$A=x^2+y^2+z^2=(x+y+z)^2-2(xy+yz+xz)=36-2(xy+yz+xz)$
Vì $x,y,z\geq 0\Rightarrow xy+yz+xz\geq 0$
$\Rightarrow A=36-2(xy+yz+xz)\leq 36$
Vậy $A_{\max}=36$. Giá trị này đạt tại $(x,y,z)=(0,0,6)$ và hoán vị.
Tìm GTLN của biểu thức P=xy+2yz=xz trong đó x,y,z là các số thực thỏa mãn \(x\ge y\ge z>0\)và \(1+4\sqrt{2}-2\sqrt{2}x^2=z^2=5-4y^2\)
Cho các số thực x,y,z thỏa mãn: x+y+z=6.Tìm giá trị lớn nhất của A=xy+2yz+3zx
từ giả thiết ta có : z = 6 - x - y
Ta có : \(A=xy+z\left(2y+3x\right)=xy+\left(6-x-y\right)\left(2y+3x\right)\)
\(=-3x^2-2y^2-4xy+18x+12y\)
Do đó : \(3A=-9x^2-6y^2-12xy+54x+36y=-9x^2-6x\left(2y-9\right)-6y^2+36y\)
\(=-\left(3x+2y-9\right)^2-2y^2+81\le81\)
\(\Rightarrow A\le27\)
Vậy giá trị lớn nhất của A là 27 \(\Leftrightarrow\hept{\begin{cases}3x+2y-9=0\\y=0\end{cases}\Leftrightarrow x=3;y=0;z=3}\)
Cho x,y,z >0 thỏa mãn x+y+z = 2. Tìm GTLN của biểu thức
\(P=\sqrt{2x+yz}+\sqrt{2y+xz}+\sqrt{2z+xy}\)
\(\sqrt{2x+yz}=\sqrt{x\left(x+y+z\right)+yz}=\sqrt{\left(x+y\right)\left(x+z\right)}\le\dfrac{1}{2}\left(x+y+x+z\right)=\dfrac{1}{2}\left(2x+y+z\right)\)
Tương tự: \(\sqrt{2y+xz}\le\dfrac{1}{2}\left(x+2y+z\right)\) ; \(\sqrt{2z+xy}\le\dfrac{1}{2}\left(x+y+2z\right)\)
Cộng vế:
\(P\le\dfrac{1}{2}\left(4x+4y+4z\right)=4\)
\(P_{max}=4\) khi \(x=y=z=\dfrac{2}{3}\)
P = \(1.\sqrt{2x+yz}+1.\sqrt{2y+xz}+1.\sqrt{2z+xy}\)
\(\le\sqrt{\left(1^2+1^2+1^2\right)\left(2x+yz+2y+xz+2z+xy\right)}\)
\(=\sqrt{3.\left(4+xy+yz+zx\right)}\)
Đã biết x2 + y2 + z2 \(\ge\)xy + yz + zx
=> xy + yz + zx \(\le\dfrac{\left(x+y+z\right)^2}{3}\)
Khi đó \(P\le\sqrt{3\left(4+xy+yz+zx\right)}\le\sqrt{3\left[4+\dfrac{\left(x+y+z\right)^2}{3}\right]}\)
= 4
Dấu "=" xảy ra <=> x = 2/3
Cho x,y,z >0 thỏa mãn \(x+y+z=2\) . Tìm GTLN của biểu thức \(P=\sqrt{2x+yz}+\sqrt{2y+xz}+\sqrt{2z+xy}\)
\(\sqrt{2x+yz}=\sqrt{\left(x+y+z\right)x+yz}=\sqrt{\left(x+y\right)\left(x+z\right)}\le\dfrac{x+2y+z}{2}\\ \Leftrightarrow P=\sum\sqrt{2x+yz}\le\dfrac{x+2y+z+2x+y+z+x+y+2z}{2}=\dfrac{4\left(x+y+z\right)}{2}=2\cdot2=4\)
Dấu \("="\Leftrightarrow x=y=z=\dfrac{2}{3}\)
P = \(1.\sqrt{2x+yz}+1.\sqrt{2y+xz}+1.\sqrt{2z+xy}\)
\(\le\sqrt{\left(1^2+1^2+1^2\right)\left(2x+yz+2y+xz+2z+xy\right)}\)(Bunyacovski)
\(=\sqrt{3\left[4+\left(xy+yz+zx\right)\right]}\)
\(\le\sqrt{3.\left[4+\dfrac{\left(x+y+z\right)^2}{3}\right]}=\sqrt{3.\left(4+\dfrac{4}{3}\right)}\) = 4
Dấu "=" xảy ra <=> x = y = z = 2/3
Cho x,y,z thỏa mãn điều kiện \(0\le x,y,z\le1\). Tìm GTLN của biểu thức \(M=x^{10}+y^6+z^{2016}-xy-yz-xz\)
\(0\le x,y,z\le1\Rightarrow x^{10}\le x;y^6\le y;z^{2016}\le z;0\le xyz\le1\)
CÓ: \(\left(1-x\right)\left(1-y\right)\left(1-z\right)\ge0\)
=>\(1-xyz+\left(xy+yz+zx\right)-\left(x+y+z\right)\ge0\)
=>\(x+y+z-xy-yz-zx-xyz\le1\)
=>\(x^{10}+y^6+z^{2016}-xy-yz-zx\le1\)
Dấy "=" xảy ra <=> trong 3 số x,y,z có 1 số bằng 0, 2 số bằng 1 hoặc 1 số bằng 1, 2 số bằng 0