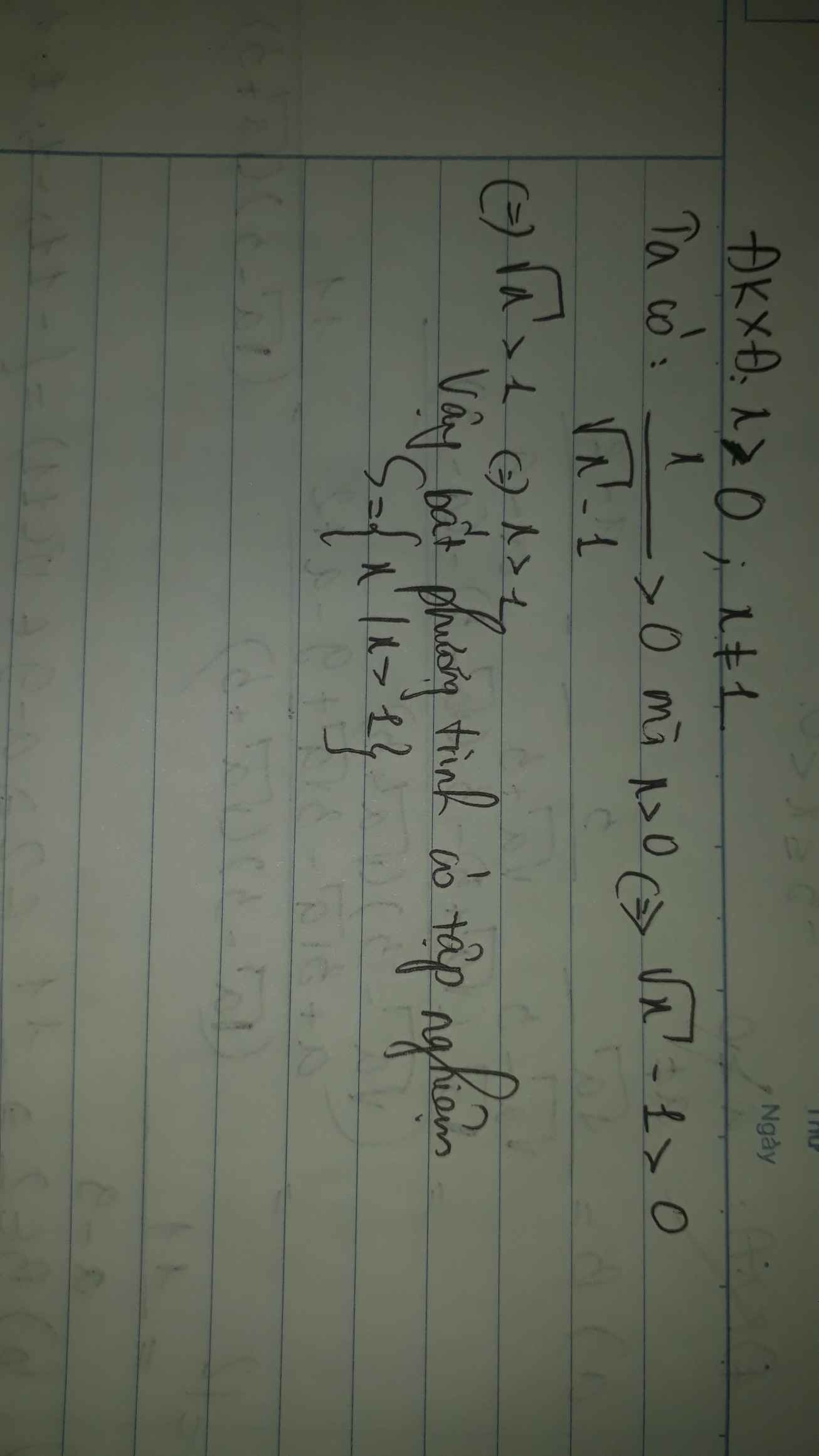giải bất phương trình \(\dfrac{2012}{x}< 2013\) ( x \(\ne\)0)
VT
Những câu hỏi liên quan
Giải phương trình:
\(\dfrac{\sqrt{x-2012}-1}{x-2012}+\dfrac{\sqrt{y-2013}-1}{y-2013}+\dfrac{\sqrt{z-2014}-1}{z-2014}=\dfrac{3}{4}\)
Điều kiện: \(x\ge2012;y\ge2013;z\ge2014\)
Áp dụng bất đẳng thức Cauchy, ta có:
\(\left\{{}\begin{matrix}\dfrac{\sqrt{x-2012}-1}{x-2012}=\dfrac{\sqrt{4\left(x-2012\right)}-2}{2\left(x-2012\right)}\le\dfrac{\dfrac{4+x-2012}{2}-2}{2\left(x-2012\right)}=\dfrac{1}{4}\\\dfrac{\sqrt{y-2013}-1}{y-2013}=\dfrac{\sqrt{4\left(y-2013\right)}-2}{2\left(y-2013\right)}\le\dfrac{\dfrac{4+y-2013}{2}-2}{2\left(y-2013\right)}=\dfrac{1}{4}\\\dfrac{\sqrt{z-2014}-1}{z-2014}=\dfrac{\sqrt{4\left(z-2014\right)}-2}{2\left(z-2014\right)}\le\dfrac{\dfrac{4+z-2014}{2}-2}{2\left(z-2014\right)}=\dfrac{1}{4}\end{matrix}\right.\)
Cộng vế theo vế, ta được:
\(\dfrac{\sqrt{x-2012}-1}{x-2012}+\dfrac{\sqrt{y-2013}-1}{y-2013}+\dfrac{\sqrt{z-2014}-1}{z-2014}\le\dfrac{3}{4}\)
Đẳng thức xảy ra khi \(x=2016;y=2017;z=2018\)
Vậy....
Đúng 2
Bình luận (0)
Giải phương trình sau:
\(\dfrac{x-1}{2013}\)+\(\dfrac{x-2}{2012}\)+\(\dfrac{x-3}{2011}\)=\(\dfrac{x-4}{2010}\)+\(\dfrac{x-5}{2009}\)+\(\dfrac{x-6}{2008}\)
`(x-1)/2013+(x-2)/2012+(x-3)/2011=(x-4)/2010+(x-5)/2009 +(x-6)/2008`
`<=> ((x-1)/2013-1)+((x-2)/2012-1)+((x-3)/2011-1)=( (x-4)/2010-1)+((x-5)/2009-1)+((x-6)/2008-1)`
`<=> (x-2014)/2013 +(x-2014)/2012+(x-2014)/2011=(x-2014)/2010+(x-2014)/2009+(x-2014)/2008`
`<=> x-2014=0` (Vì `1/2013+1/2012+1/2011-1/2010-1/2009-1/2008 \ne 0`)
`<=>x=2014`
Vậy `S={2014}`.
Đúng 2
Bình luận (0)
Giải phương trình sau:
\(\dfrac{x-1}{2013}+\dfrac{x-2}{2012}+\dfrac{x-3}{2011}=\dfrac{x-4}{2010}+\dfrac{x-5}{2009}+\dfrac{x-6}{2008}\)
\(\dfrac{x-1}{2013}-1+\dfrac{x-2}{2012}-1+\dfrac{x-3}{2011}-1=\dfrac{x-4}{2019}-1+\dfrac{x-5}{2010}-1+\dfrac{x-6}{2008}-1\)
\(\Leftrightarrow\dfrac{x-2014}{2013}+\dfrac{x-2014}{2012}+\dfrac{x-2014}{2011}-\dfrac{x-2014}{2010}-\dfrac{x-2014}{2009}-\dfrac{x-2014}{2008}=0\)
\(\Leftrightarrow\left(x-2014\right)\left(\dfrac{1}{2013}+\dfrac{1}{2012}+\dfrac{1}{2011}-\dfrac{1}{2010}-\dfrac{1}{2009}-\dfrac{1}{2008}\ne0\right)=0\Leftrightarrow x=2014\)
Đúng 1
Bình luận (0)
Giải phương trình sau:
\(\dfrac{x-1}{2013}+\dfrac{x-2}{2012}+\dfrac{x-3}{2011}=\dfrac{x-4}{2010}+\dfrac{x-5}{2009}+\dfrac{x-6}{2008}\)
\(\dfrac{x-1}{2013}+\dfrac{x-2}{2012}+\dfrac{x-3}{2011}=\dfrac{x-4}{2010}+\dfrac{x-5}{2009}+\dfrac{x-6}{2008}\)
\(\Leftrightarrow\left(\dfrac{x-1}{2013}-1\right)+\left(\dfrac{x-2}{2012}-1\right)+\left(\dfrac{x-3}{2011}-1\right)=\left(\dfrac{x-4}{2010}-1\right)+\left(\dfrac{x-5}{2009}-1\right)+\left(\dfrac{x-6}{2008}-1\right)\)
\(\Leftrightarrow\dfrac{x-2014}{2013}+\dfrac{x-2014}{2012}+\dfrac{x-2014}{2011}=\dfrac{x-2014}{2010}+\dfrac{x-2014}{2009}+\dfrac{x-2014}{2008}\)
\(\Leftrightarrow\dfrac{x-2014}{2013}+\dfrac{x-2014}{2012}+\dfrac{x-2014}{2011}-\dfrac{x-2014}{2010}-\dfrac{x-2014}{2009}-\dfrac{x-2014}{2008}=0\)
\(\Leftrightarrow\left(x-2014\right)\left(\dfrac{1}{2013}+\dfrac{1}{2012}+\dfrac{1}{2011}-\dfrac{1}{2010}-\dfrac{1}{2009}-\dfrac{1}{2008}\right)=0\)
\(\Leftrightarrow\left(x-2014\right).A=0\)
\(\text{Vì A }\ne0\)
\(\Rightarrow x-2014=0\)
\(\Leftrightarrow x=2014\)
\(\text{Vậy phương trình có tập nghiệm là }S=\left\{2014\right\}\)
Đúng 1
Bình luận (0)
giải bất phương trình sau : ( x - 2014 ) ( x - 2012 ) > 0
\(\Leftrightarrow\left[{}\begin{matrix}x>2014\\x< 2012\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Giải phương trình
X -3/2012+X-2/2013=X-2013/2+X-2012/3
Giải ra dùm mình luôn nha
Bạn tham khảo nhé :
Ta có :
\(\frac{x-3}{2012}+\frac{x-2}{2013}=\frac{x-2013}{2}+\frac{x-2012}{3}\)
\(\Leftrightarrow\)\(\left(\frac{x-3}{2012}-1\right)+\left(\frac{x-2}{2013}-1\right)=\left(\frac{x-2013}{2}-1\right)+\left(\frac{x-2012}{3}-1\right)\)
\(\Leftrightarrow\)\(\frac{x-2015}{2012}+\frac{x-2015}{2013}=\frac{x-2015}{2}+\frac{x-2015}{3}\)
\(\Leftrightarrow\)\(\frac{x-2015}{2012}+\frac{x-2015}{2013}-\frac{x-2015}{2}-\frac{x-2015}{3}=0\)
\(\Leftrightarrow\)\(\left(x-2015\right)\left(\frac{1}{2012}+\frac{1}{2013}-\frac{1}{2}-\frac{1}{3}\right)=0\)
Mà \(\frac{1}{2012}+\frac{1}{2013}-\frac{1}{2}-\frac{1}{3}\ne0\)
\(\Rightarrow\)\(x-2015=0\)
\(\Rightarrow\)\(x=2015\)
Vậy \(x=2015\)
Chsuc bạn học tốt ~
Đúng 0
Bình luận (0)
chuyển vế t cs
x+x-x-x=....
0x=.....
Suy ra vô nghiệm
Đúng 0
Bình luận (0)
(=) [(x-3/2012)-1]+[(x-2/2013)-1]= [(x-2013/2)-1]+[(x-2012/3)-1]
(=) x-2015/2012 . +. x-2015/2013. = x-2015/2. +. x-2015/3
(=)( x-2015 ) × (1/2012 + . 1/2013. -. 1/2 . - 1/3 .) =0
Mà 1/2012+1/2013-1/2-1/3≠0
=) x-2015 =0
(=) x=2015
Đúng 0
Bình luận (0)
Giải phương trình sau:
\(\frac{x+1}{2014}+\frac{x+2}{2013}+\frac{x+3}{2012}+\frac{x+2045}{10}=0\)
bn bị rảnh ak ?
ko trả lời thì đừng có viết linh tinh
Đúng 0
Bình luận (0)
\(\frac{x+1}{2014}+\frac{x+2}{2013}+\frac{x+3}{2012}+\frac{x+2045}{10}=0\)
\(\Leftrightarrow\frac{x+1}{2014}+1+\frac{x+2}{2013}+1+\frac{x+3}{2012}+1+\frac{x+2045}{10}-3=0\)
\(\Leftrightarrow\frac{x+1+2014}{2014}+\frac{x+2+2013}{2013}+\frac{x+3+2012}{2012}+\frac{x+2045-3.10}{10}=0\)
\(\Leftrightarrow\frac{x+2015}{2014}+\frac{x+2015}{2013}+\frac{x+2015}{2012}+\frac{x+2015}{10}=0\)
\(\Leftrightarrow\left(x+2015\right).\left(\frac{1}{2014}+\frac{1}{2013}+\frac{1}{2012}+\frac{1}{10}\right)=0\)
Vì \(\frac{1}{2014}+\frac{1}{2013}+\frac{1}{2012}+\frac{1}{10}\ne0\)
Nên x + 2015 = 0 <=> x = -2015
Vậy x = -2015
Đúng 1
Bình luận (0)
Giải bất phương trình: \(\dfrac{x}{\sqrt{x}-1}\)>0
ĐKXĐ: \(x\ge0\)
\(\dfrac{x}{\sqrt{x}-1}>0\Leftrightarrow\left\{{}\begin{matrix}x\ne0\\\sqrt{x}-1>0\end{matrix}\right.\)
\(\Leftrightarrow x>1\)
Đúng 0
Bình luận (0)
ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne1\end{matrix}\right.\)
Ta có: \(\dfrac{x}{\sqrt{x}-1}>0\)
mà x>0
nên \(\sqrt{x}-1>0\)
hay x>1
Đúng 0
Bình luận (0)
giải bất phương trình
\(\dfrac{x^2}{x-2}\) < 0
Có: x2 > 0
⇒ x - 2 < 0
⇔ x < 2
Vậy: ...
Đúng 3
Bình luận (0)