Cho a = log 2 7 ; b = log 5 7 . Giá trị của log 7 bằng
A.![]()
B. ![]()
C. a + b
D. ![]()
1. cho a=log3 2 và b=log3 5. tính các logarit sau theo a, b; A=log3 80, B=log3 37,5
2. cho log10 3=a, log5=b. tính C=log30 8 theo a, b
3. cho log27 5=a, log8 7=b, log2 3=c. tính D log6 35 theo a, b, c
Bài 1:
\(A=\log_380=\log_3(2^4.5)=\log_3(2^4)+\log_3(5)\)
\(=4\log_32+\log_35=4a+b\)
\(B=\log_3(37,5)=\log_3(2^{-1}.75)=\log_3(2^{-1}.3.5^2)\)
\(=\log_3(2^{-1})+\log_33+\log_3(5^2)=-\log_32+1+2\log_35\)
\(=-a+1+2b\)
Bài 2:
\(\log_{30}8=\frac{\log 8}{\log 30}=\frac{\log (2^3)}{\log (10.3)}=\frac{3\log2}{\log 10+\log 3}\)
\(=\frac{3\log (\frac{10}{5})}{1+\log 3}=\frac{3(\log 10-\log 5)}{1+\log 3}=\frac{3(1-b)}{1+a}\)
Bài 3:
\(\log_{27}5=a; \log_87=b; \log_23=c\)
\(\Leftrightarrow \frac{\ln 5}{\ln 27}=a; \frac{\ln 7}{\ln 8}=b; \frac{\ln 3}{\ln 2}=c\)
\(\Leftrightarrow \frac{\ln 5}{\ln (3^3)}=a; \frac{\ln 7}{\ln (2^3)}=b; \ln 3=c\ln 2\)
\(\Leftrightarrow \frac{\ln 5}{3\ln 3}=a; \frac{\ln 7}{3\ln 2}=b; \ln 3=c\ln 2\)
\(\Rightarrow \frac{\ln 5}{3c\ln 2}=a; \frac{\ln 7}{3\ln 2}=b\)
\(\Rightarrow \ln 35=\ln 5+\ln 7=3ac\ln 2+3b\ln 2\)
Do đó:
\(D=\log_6 35=\frac{\ln 35}{\ln 6}=\frac{\ln 35}{\ln 2+\ln 3}=\frac{\ln 35}{\ln 2+c\ln 2}=\frac{3ac\ln 2+3b\ln 2}{\ln 2+c\ln 2}\)
\(=\frac{3ac+3b}{1+c}\)
Tính giá trị của các biểu thức sau:
a) \(A = {\log _2}3.{\log _3}4.{\log _4}5.{\log _5}6.{\log _6}7.{\log _7}8;\)
b) \(B = {\log _2}2.{\log _2}4...{\log _2}{2^n}.\)
\(a,A=log_23\cdot log_34\cdot log_45\cdot log_56\cdot log_67\cdot log_78\\ =log_28\\ =log_22^3\\ =3\\ b,B=log_22\cdot log_24...log_22^n\\ =log_22\cdot log_22^2...log_22^n\\ =1\cdot2\cdot...\cdot n\\ =n!\)
Đặt \({\log _3}2 = a,{\log _3}7 = b\). Biểu thị \({\log _{12}}21\) theo \(a\) và \(b\).
\(log_{12}21=\dfrac{log_321}{log_312}=\dfrac{log_3\left(7\cdot3\right)}{log_3\left(2^2\cdot3\right)}=\dfrac{log_37+log_33}{log_34+log_33}\)
\(=\dfrac{log_37+1}{log_32^2+1}=\dfrac{log_37+1}{2\cdot log_32+1}=\dfrac{b+1}{2a+1}\)
Hoạt động 3
Cho \(m = {2^7};\,n = {2^3}\)
a) Tính \({\log _2}\left( {mn} \right);{\log _2}m + {\log _2}n\) và so sánh các kết quả đó
b) Tính \({\log _2}\left( {\frac{m}{n}} \right);{\log _2}m - {\log _2}n\) và so sánh các kết quả đó
a: \(log_2\left(mn\right)=log_2\left(2^7\cdot2^3\right)=7+3=10\)
\(log_2m+log_2n=log_22^7+log_22^3=7+3=10\)
=>\(log_2\left(mn\right)=log_2m+log_2n\)
b: \(log_2\left(\dfrac{m}{n}\right)=log_2\left(\dfrac{2^7}{2^3}\right)=7-3=4\)
\(log_2m-log_2n=log_22^7-log_22^3=7-3=4\)
=>\(log_2\left(\dfrac{m}{n}\right)=log_2m-log_2n\)
a) \(\log_2\left(mn\right)=\log_2\left(2^7.2^3\right)=\log_22^{7+3}=\log_22^{10}=10.\log_22=10.1=10\)
\(\log_2m+\log_2n=\log_22^7+\log_22^3=7\log_22+3\log_22=7.1+3.1=7+3=10\)
b) \(\log_2\left(\dfrac{m}{n}\right)=\log_2\dfrac{2^7}{2^3}=\log_22^4=4.\log_22=4.1=4\)
\(\log_2m-\log_2n=\log_22^7-\log_22^3=7.\log_22-3\log_22=7.1-3.1=4\)
log3\(\sqrt{3}\)=... , log100=... , lne3=... , log27 3=... , log\(\sqrt{3}\)3=... , log0,125 2=... , log\(\sqrt[3]{49}\)7=...,
log\(\dfrac{1}{125}\)5=... , log8 4=... , log25\(\dfrac{1}{5}\)=... , log\(\dfrac{1}{5}\)\(\sqrt{5}\)=... , log\(\dfrac{1}{7}\)\(\sqrt[5]{49}\)=... , log4 \(\dfrac{1}{\sqrt{2}}\)=... , log27 \(3\sqrt{3}\)=...
\(log_3\sqrt{3}=log_33^{\dfrac{1}{2}}=\dfrac{1}{2}\)
\(lne^3=log_ee^3=3\)
\(log_{27}3=log_{3^3}3=\dfrac{1}{3}\)
\(\log_{\sqrt{3}}3=log_{3^{\dfrac{1}{2}}}3=1:\dfrac{1}{2}=2\)
\(\log_{0,125}2=log_{2^{-3}}2=\dfrac{1}{-3}\)
\(\log_{\sqrt[3]{49}}7=\log_{7^{\dfrac{2}{3}}}7=1:\dfrac{2}{3}=\dfrac{3}{2}\)
\(\log_{\dfrac{1}{125}}5=\log_{5^{-3}}5=-\dfrac{1}{3}\)
\(\log_84=log_{2^3}2^2=\dfrac{1}{3}\cdot2=\dfrac{2}{3}\)
\(\log_{25}\left(\dfrac{1}{5}\right)=\log_{5^2}5^{-1}=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}\)
\(\log_{\dfrac{1}{5}}\sqrt{5}=\log_{5^{-1}}5^{\dfrac{1}{2}}=\dfrac{1}{-1}\cdot\dfrac{1}{2}=-\dfrac{1}{2}\)
\(log_{\dfrac{1}{7}}\sqrt[5]{49}=\log_{7^{-1}}7^{\dfrac{2}{5}}=\dfrac{1}{-1}\cdot\dfrac{2}{5}=-\dfrac{2}{5}\)
\(\log_4\left(\dfrac{1}{\sqrt{2}}\right)=\log_{2^2}\left(\sqrt{2}\right)^{-1}\)
\(=\log_{2^{-2}}\left(\sqrt{2}\right)^{-\dfrac{1}{2}}=\dfrac{1}{-2}\cdot\dfrac{-1}{2}=\dfrac{1}{4}\)
\(\log_{27}3\sqrt{3}=\log_{3^3}3^{\dfrac{3}{2}}=\dfrac{1}{3}\cdot\dfrac{3}{2}=\dfrac{1}{2}\)
Cho hai số thực dương a, b với \(a \ne 1\). Khẳng định nào sau đây là đúng?
A. \({\log _a}\left( {{a^3}{b^2}} \right) = 3 + {\log _a}b\).
B. \({\log _a}\left( {{a^3}{b^2}} \right) = 3 + 2{\log _a}b\).
C. \({\log _a}\left( {{a^3}{b^2}} \right) = \frac{3}{2} + {\log _a}b\).
D. \({\log _a}\left( {{a^3}{b^2}} \right) = \frac{1}{3} + \frac{1}{2}{\log _a}b\).
\(log_a\left(a^3b^2\right)=log_aa^3+log_ab^2=3+2\cdot log_ab\)
=>B
Cho Log 3 6 = a, Log 2 5 = b . Tính Log 10 90 theo a b
Mình cảm ơn ạ !
Đặt m = log 2 và n = log 7. Hãy biểu diễn log 6125 7 theo m và n.
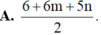
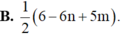
![]()
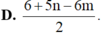
Đáp án D.
Ta có
log 6125 7 = log 6125 + log 7 = log 7 2 . 125 + 1 2 log 7
= 5 2 log 7 + log 5 3 = 5 2 n + 3 log 5 = 5 2 n + 3 1 - log 2
= 5 2 n + 3 - 3 m .
Cho a, b là các số thực dương thỏa mãn log 2 a + log 2 b = 0.
Giải các bất phương trình sau:
a) \({\log _{\frac{1}{7}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right);\)
b) \(2\log \left( {2x + 1} \right) > 3.\)
a) \({\log _{\frac{1}{7}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\) (ĐK: \(x + 1 > 0;2 - x > 0 \Leftrightarrow - 1 < x < 2\))
\(\begin{array}{l} \Leftrightarrow {\log _{{7^{ - 1}}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow - {\log _7}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\log _7}{\left( {x + 1} \right)^{ - 1}} > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\left( {x + 1} \right)^{ - 1}} > 2 - x\\ \Leftrightarrow \frac{1}{{x + 1}} - 2 + x > 0\\ \Leftrightarrow \frac{{1 + \left( {x - 2} \right)\left( {x + 1} \right)}}{{x + 1}} > 0\\ \Leftrightarrow \frac{{1 + {x^2} - x - 2}}{{x + 1}} > 0 \Leftrightarrow \frac{{{x^2} - x - 1}}{{x + 1}} > 0\end{array}\)
Mà – 1 < x < 2 nên x + 1 > 0
\( \Leftrightarrow {x^2} - x - 1 > 0 \Leftrightarrow \left[ \begin{array}{l}x < \frac{{1 - \sqrt 5 }}{2}\\x > \frac{{1 + \sqrt 5 }}{2}\end{array} \right.\)
KHĐK ta có \(\left[ \begin{array}{l} - 1 < x < \frac{{1 - \sqrt 5 }}{2}\\\frac{{1 + \sqrt 5 }}{2} < x < 2\end{array} \right.\)
b) \(2\log \left( {2x + 1} \right) > 3\) (ĐK: \(2x + 1 > 0 \Leftrightarrow x > \frac{{ - 1}}{2}\))
\(\begin{array}{l} \Leftrightarrow \log \left( {2x + 1} \right) > \frac{3}{2}\\ \Leftrightarrow 2x + 1 > {10^{\frac{3}{2}}} = 10\sqrt {10} \\ \Leftrightarrow x > \frac{{10\sqrt {10} - 1}}{2}\end{array}\)
KHĐK ta có \(x > \frac{{10\sqrt {10} - 1}}{2}\)