Đặt \(A=\sqrt[3]{9+4\sqrt{5}}+\sqrt[3]{9-4\sqrt{5}}\)
\(\Leftrightarrow A^3=18+3\cdot\sqrt[3]{\left(9+4\sqrt{5}\right)\left(9-4\sqrt{5}\right)}\left(\sqrt[3]{9+4\sqrt{5}}+\sqrt[3]{9-4\sqrt{5}}\right)\\ \Leftrightarrow A^3=18+3A\sqrt[3]{1}\\ \Leftrightarrow A^3=3A+18\\ \Leftrightarrow A^3-3A-18=0\\ \Leftrightarrow\left(A-3\right)\left(A^2+3A+6\right)=0\\ \Leftrightarrow A=3\left[A^2+3A+6=\left(A+\dfrac{3}{2}\right)^2+\dfrac{15}{4}>0\right]\)
Vậy \(\sqrt[3]{9+4\sqrt{5}}+\sqrt[3]{9-4\sqrt{5}}=3\)
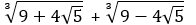


 giải giúp a b
giải giúp a b 