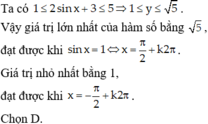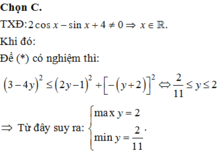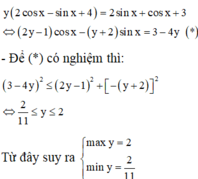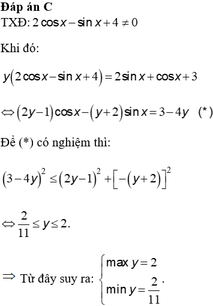tìm GTLN và GTNN của hàm số y=2/2sinx+3
PH
Những câu hỏi liên quan
Tìm GTLN và GTNN của hàm sau:
y
2
sin
x
+
3
Đọc tiếp
Tìm GTLN và GTNN của hàm sau: y = 2 sin x + 3
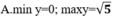
![]()
![]()
![]()
Tìm GTLN và GTNN của hàm số:
y
2
sin
x
+
cos
x
+
3
2
cos
x
-
sin
x
+
4
Đọc tiếp
Tìm GTLN và GTNN của hàm số: y = 2 sin x + cos x + 3 2 cos x - sin x + 4
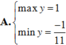
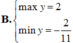
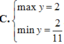
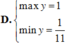
Tìm GTLN và GTNN của hàm số
y
2
sin
x
+
cos
x
+
3
2
cos
x
-
sin
x
+
4
là
Đọc tiếp
Tìm GTLN và GTNN của hàm số y = 2 sin x + cos x + 3 2 cos x - sin x + 4 là
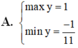
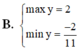
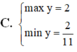
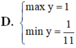
Tìm GTLN và GTNN của hàm số
y
2
sin
x
+
cos
x
+
3
2
cos
x
-
sin
x
+
4
là: A.
m
a...
Đọc tiếp
Tìm GTLN và GTNN của hàm số y = 2 sin x + cos x + 3 2 cos x - sin x + 4 là:
A. m a x y = 1 m i n y = - 1 11
B. m a x y = 2 m i n y = - 2 11
C. m a x y = 2 m i n y = 2 11
D. m a x y = 1 m i n y = 1 11
Tìm GTLN và GTNN của hàm số : 1. y = sinx + 2cosx +1 / 2sinx + cosx + 3
2.y= 2sin^2sinx - 3 sinx cosx + cos^2 x
Giải phương trình : 1. 2sin^2 * 2x + sin7x -1 = sinx
2.cos 4x + 12 sin^2 x -1 = 0
tìm gtln gtnn của hàm số y=|sinx +cos2x|-2sinx
\(y=\left|2sin^2x-sinx-1\right|-2sinx\)
Đặt \(sinx=t\in\left[-1;1\right]\)
\(\Rightarrow y=f\left(t\right)=\left|2t^2-t-1\right|-2t\)
BBT cho \(f\left(t\right)\) trên \(\left[-1;1\right]\):
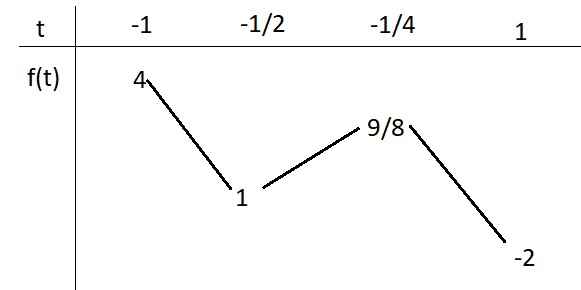
Từ BBT ta thấy \(y_{max}=4\) khi \(sinx=-1\); \(y_{min}=-2\) khi \(sinx=1\)
Đúng 1
Bình luận (0)
Tìm GTLN, GTNN của hàm số y = 4cos²x + 2sinx +2 , giúp em với em cảm ơn !
\(y=4\left(1-sin^2x\right)+2sinx+2=-4sin^2x+2sinx+6\)
Đặt \(sinx=t\in\left[-1;1\right]\Rightarrow y=f\left(t\right)=-4t^2+2t+6\)
\(-\dfrac{b}{2a}=\dfrac{1}{4}\in\left[-1;1\right]\)
\(f\left(-1\right)=0\) ; \(f\left(\dfrac{1}{4}\right)=\dfrac{25}{4}\); \(f\left(1\right)=4\)
\(\Rightarrow y_{max}=\dfrac{25}{4}\) khi \(sinx=\dfrac{1}{4}\)
\(y_{min}=0\) khi \(sinx=-1\)
Đúng 0
Bình luận (0)
Ta có: \(y=4cos^2x+2sinx+2=4-4sin^2x+2sinx+2=-4sin^2x+2sinx+6=-\left(4sin^2x-2sinx+\dfrac{1}{16}-\dfrac{1}{16}-6\right)=-\left(2sin^2x-\dfrac{1}{4}\right)^2+\dfrac{97}{16}\)
Ta có: \(-\left(2sin^2x-\dfrac{1}{4}\right)^2\le0\Rightarrow y\le\dfrac{97}{16}\)
Vậy \(y_{max}=\dfrac{97}{16}\)
Đúng 0
Bình luận (0)
Tìm GTLN,GTNN của hàm số:
a, \(y=3cosx-1\)
b, \(y=5+2sinx\)
c,\(y=\sqrt{3+cos2x}\)
d,\(y=\sqrt{5sinx-1}+2\)
a.\(-1\le cosx\le1\Rightarrow-4\le y=3cosx-1\le2\)
b.-1 \(\le sinx\le1\)\(\Rightarrow3\le y=5+2sinx\le7\)
c.\(\sqrt{3-1}\le\sqrt{3+cos2x}\le\sqrt{3+1}\Rightarrow\sqrt{2}\le y\le2\)
d.\(y=\sqrt{5sinx-1}+2\le\sqrt{5.1-1}+2=4\)
\(y=\sqrt{5sinx-1}+2\ge2\) . " = " \(\Leftrightarrow sinx=\dfrac{1}{5}\Leftrightarrow\left[{}\begin{matrix}x=arcsin\left(\dfrac{1}{5}\right)+2k\pi\\x=\pi-arcsin\left(\dfrac{1}{5}\right)+2k\pi\end{matrix}\right.\) ( k thuộc Z )
Đúng 2
Bình luận (0)
Tìm GTLN, GTNN của hàm số y = √(1−2sinx)
Giải phương trình lượng giác
a) sin4x + cos4x + sin4x = 1
b) tan2x + cotx = 4cos2x
Tìm GTNN, GTLN của \(y=-4cos^2x+2sinx+3\)
Lời giải:
\(y=-4\cos ^2x+2\sin x+3=-4(1-\sin ^2x)+2\sin x+3=4\sin ^2x+2\sin x-1\)
Đặt \(\sin x=t(t\in [-1;1])\) thì:
\(y=4t^2+2t-1\)
\(y'=8t+2=0\Leftrightarrow t=-\frac{1}{4}\)
Lập BBT. Với các giá trị \(y(\frac{-1}{4})=\frac{-5}{4}; y(-1)=1; y(1)=5\) ta thấy:
\(y_{\max}=5\Leftrightarrow t=1\Leftrightarrow x=2k\pi +\frac{\pi}{2}\)
\(y_{\min}=\frac{-5}{4}\Leftrightarrow t=\frac{-1}{4}\Leftrightarrow x=2k\pi -2\tan ^{-1}(4\pm \sqrt{15})\)
Đúng 1
Bình luận (0)