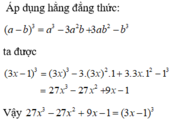27x3 + 125y3
H24
Những câu hỏi liên quan
27x3 + 125y3
\(27x^3+125y^3\)
\(=\left(3x\right)^3+\left(5y\right)^3\)
\(=\left(3x+5y\right)\left[\left(3x\right)^2-3x\cdot5y+\left(5y\right)^2\right]\)
\(=\left(3x+5y\right)\left(9x^2-15xy+25y^2\right)\)
Đúng 0
Bình luận (0)
a)8x3-1000 giúp mik :<
b)0.001+64x3 C)1/125y3+x3
d)27x3-1/8y3
\(a,=8\left(x^3-125\right)=8\left(x-5\right)\left(x^2+10x+25\right)\\ b,=\left(0,1+4x\right)\left(0,01-0,4x+16x^2\right)\\ d,=\left(3x-\dfrac{1}{2}y\right)\left(9x^2+\dfrac{3}{2}xy+\dfrac{1}{4}y^2\right)\\ c,=\left(\dfrac{1}{5}y+x\right)\left(\dfrac{1}{25}y^2-\dfrac{1}{5}xy+x^2\right)\)
Đúng 1
Bình luận (0)
a, 8x3- 1000 = (2x)3 - 103 = (2x -10). (4x2 + 20x +100)
b,\(0,001+64x^3=\left(\dfrac{1}{10}\right)^3+\left(4x\right)^3=\left(\dfrac{1}{10}+4x\right).\left(\dfrac{1}{100}-\dfrac{2}{5}x+16x^2\right)\)
c, \(\dfrac{1}{125}y^3+x^3=\left(\dfrac{1}{5}y\right)^3+x^3=\left(\dfrac{1}{5}y+x\right).\left(\dfrac{1}{25}y^2-\dfrac{1}{5}yx+x^2\right)\)
\(d,27x^3-\dfrac{1}{8}y^3=\left(3x\right)^3-\left(\dfrac{1}{2}y\right)^3=\left(3x-\dfrac{1}{2}y\right).\left(9x^2+\dfrac{3}{2}xy+\dfrac{1}{4}y^2\right)\)
Đúng 0
Bình luận (0)
Phân tích đa thức
1
64
x
6
+
125
y
3
thành nhân tử, ta được A.
(
x
2
4
+
5
y
)
(
x
2
4
-...
Đọc tiếp
Phân tích đa thức 1 64 x 6 + 125 y 3 thành nhân tử, ta được
A. ( x 2 4 + 5 y ) ( x 2 4 - 5 4 x 2 y + 5 y 2 )
B. ( x 2 4 - 5 y ) ( x 4 16 + 5 4 x 2 y + 25 y 2 )
C. ( x 2 4 + 5 y ) ( x 4 16 - 5 4 x 2 y + 25 y 2 )
D. ( x 2 4 + 5 y ) ( x 4 16 - 5 2 x 2 y + 25 y 2 )
Ta có 1 64 x 6 + 125 y 3 = 1 4 x 2 3 + ( 5 y ) 3
= ( x 2 4 + 5 y ) ( x 4 16 − 5 4 x 2 y + 5 y 2 ) = ( x 2 4 + 5 y ) ( x 4 16 - 5 4 x 2 y + 25 y 2 )
Đáp án cần chọn là: C
Đúng 0
Bình luận (0)
Câu 6: (3x – 2)3 có kết quả là
A. 3x3 – 18x2 + 54x – 8
B. 27x3 – 54x2 + 36x – 8
C. 27x3 – 8
D 3x3 – 8
27x3 + 27x2 +9x +1
\(27x^3+27x^2+9x+1\)
\(=\left(3x\right)^3+3\cdot\left(3x\right)^2\cdot1+3\cdot3x\cdot1^2+1^3\)
\(=\left(3x+1\right)^3\)
Đúng 2
Bình luận (0)
=(3x)^3+3*(3x)^2*1+3*3x*1^2+1^3
=(3x+1)^3
Đúng 0
Bình luận (0)
Để tính giá trị của biểu thức 27x^3 + 27x^2 + 9x + 1, chúng ta thực hiện các phép tính theo thứ tự từ trái qua phải:
27x^3 + 27x^2 + 9x + 1 = (27x^3 + 27x^2) + (9x + 1) = 27x^2(x + 1) + 9x + 1
Vậy giá trị của biểu thức là 27x^2(x + 1) + 9x + 1.
Đúng 0
Bình luận (0)
Tính nhanh: (27x3 – 1) : (3x – 1)
(27x3 – 1) : (3x – 1)
(Sử dụng HĐT để phân tích số bị chia thành tích)
= [(3x)3 – 1] : (3x – 1)
(Xuất hiện hằng đẳng thức (7))
= (3x – 1).[(3x)2 + 3x.1 + 12] : (3x – 1)
= (3x – 1).(9x2 + 3x + 1) : (3x – 1)
= 9x2 + 3x + 1
Đúng 0
Bình luận (0)
X x X = 27x3
X x X= 27x 3
<=>X2=81
Mà: 92=81 và (-9)2=81
=>X2=92 và X2= (-9)2
=>x=9 hoặc x= -9
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Viết dưới dạng tích : 27x3 + y3
\(27x^3+y^3=\left(3x+y\right)\left(9x^2-3xy+y^2\right)\)
Đúng 1
Bình luận (0)
\(=\left(3x+y\right)\left(9x^2-3xy+y^2\right)\)
Đúng 1
Bình luận (0)
Điền vào chỗ chấm:
27
x
3
-
27
x
2
+
.
.
.
-
.
.
.
.
.
.
-
1
3
A.
27
x
3
-
27
x
2...
Đọc tiếp
Điền vào chỗ chấm: 27 x 3 - 27 x 2 + . . . - . . . = . . . - 1 3
A. 27 x 3 - 27 x 2 + 3 x - 1 = 3 x - 1 3
B. 27 x 3 - 27 x 2 + 8 x - 1 = 3 x - 1 3
C. 27 x 3 - 27 x 2 + 9 x - 1 = 3 x - 1 3
D. 27 x 3 - 27 x 2 + 9 x - 9 = 3 x - 1 3