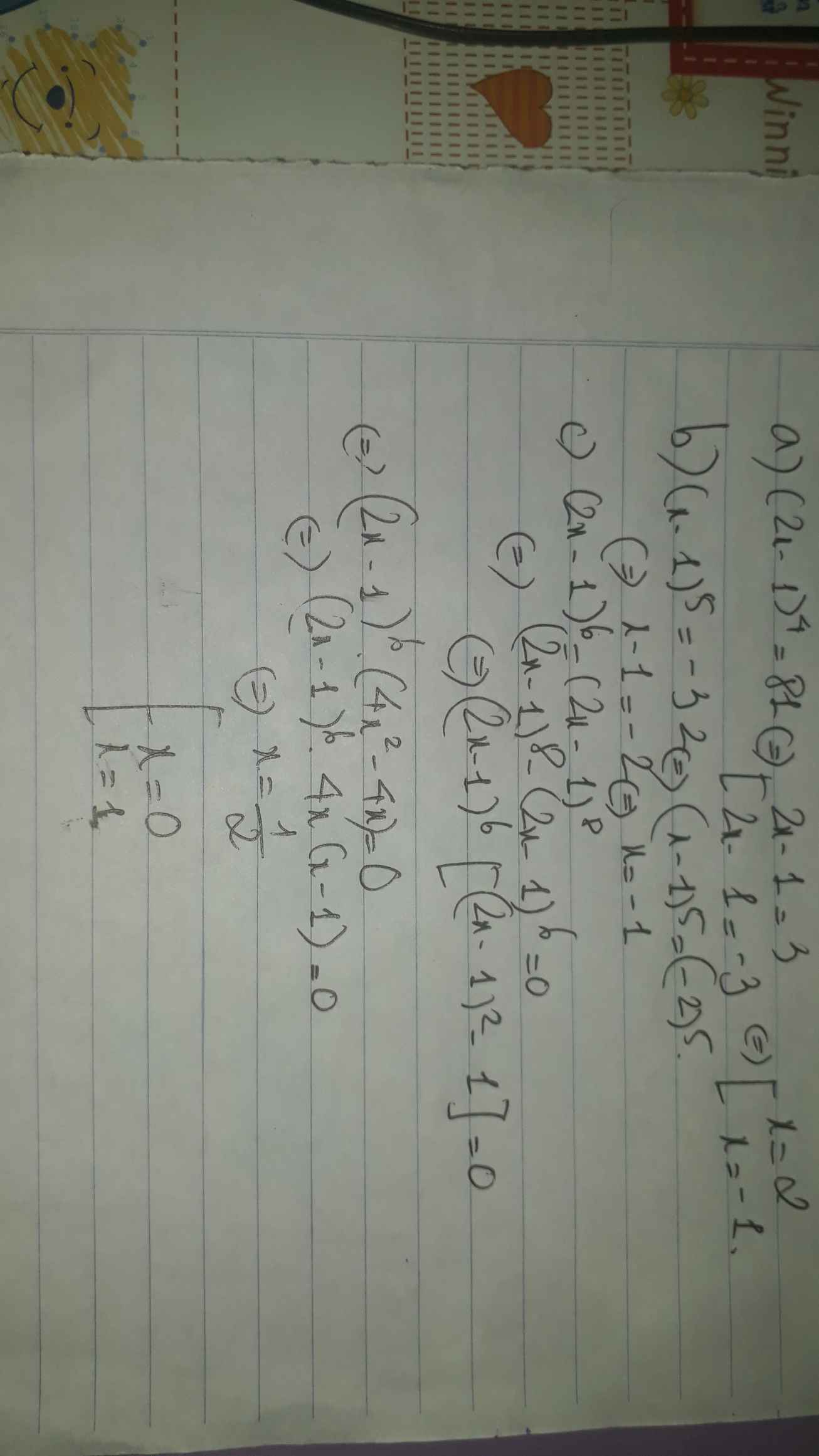2x+1–2x=32
NH
Những câu hỏi liên quan
\(\dfrac{2x}{32}=1\)
\(\dfrac{2x}{32}=1\)
\(\dfrac{2x}{32}=1\)
cả 3 bài đều giống nhau nên mình làm 1 bài thôi nhé
\(\dfrac{2x}{32}=1\Rightarrow2x=32\)
x = 32 : 2
x = 16
Đúng 0
Bình luận (0)
\(\dfrac{2x}{32}=1\)
\(\Rightarrow2x.1=32.1\)
\(\Rightarrow2x=32\)
\(\Rightarrow x=16\)
Đúng 0
Bình luận (2)
\(\dfrac{2x}{32}=1\)
\(\Rightarrow2x=32\)
\(x=16\)
Chúc bn học tốt ![]()
![]()
![]()
![]()
![]()
Đúng 0
Bình luận (0)
2x+1 – 2x = 32
\(\Leftrightarrow2^x=32\)
hay x=5
Đúng 1
Bình luận (0)
(2x + 1)y - 2x - 1 = -32
\(\left(2x+1\right)y-2x-1=-32\)
\(\Leftrightarrow2xy-2x+y-1=-32\)
\(\Leftrightarrow2x\left(y-1\right)+\left(y-1\right)=-32\)
\(\left(2x+1\right)\left(y-1\right)=-32\)
Đề bài nên cho là x,y thuộc Z; chia trường hợp rồi làm :vv
(2x + 1) y − 2x − 1 = −32
⇔2xy − 2x + y − 1 = −32
⇔2x (y − 1) + (y − 1) = −32
(2x + 1) (y − 1) = −32
=> 2x+1 và y-1 thuộc Ư(-32)
| 2x+1 | 1 | -32 | -1 | 32 | 9 | -4 | -9 | -9 | ||||
| y-1 | -32 | 1 | 32 | -1 | -4 | 9 | 4 | -4 | ||||
| x | 0 | -33/2 | -1 | 31/2 | 4 | -5/2 | -5 | -5 | ||||
| y | -31 | 2 | 34 | 0 | -3 | 10 | 5 | -3 |
vậy.............................................................
Bài giải
\(\left(2x+1\right)y-2x-1=-32\)
\(\left(2x+1\right)y-\left(2x+1\right)=-32\)
\(\left(2x+1\right)\left(y-1\right)=-32\)
\(\Rightarrow\text{ }2x+1\text{ , }y-1\inƯ\left(-31\right)=\left\{\pm1\text{ ; }\pm31\right\}\)
Ta có bảng :
| 2x + 1 | - 1 | - 31 | 1 | 31 |
| y - 1 | - 31 | - 1 | 31 | 1 |
| x | - 1 | - 16 | 0 | 15 |
| y | - 30 | 0 | 32 | 2 |
\(\Rightarrow\text{ }\left(x\text{ ; }y\right)=\left(-1\text{ ; }-30\right)\text{ ; }\left(-16\text{ ; }0\right)\text{ ; }\left(0\text{ ; }32\right)\text{ ; }\left(15\text{ ; }2\right)\)
1. Tìm x, y ∈ N biếta) 19 - (x + 23) 24 - 6b) 43 + 32 : (x + 1) - 65c) (2x + 1)3 - 52 102d) 15 . 2x - 7 . 2 +x-2 212e) 1 + 3 + 32 + .... + 3x 314g) 2x - 2y 72. a) So sánh 2150 và 3100b) Tìm chữ số tận cùng của A 22023 + 32024
Đọc tiếp
1. Tìm x, y ∈ N biết
a) 19 - (x + 23) = 24 - 6
b) 43 + 32 : (x + 1) - 65
c) (2x + 1)3 - 52 = 102
d) 15 . 2x - 7 . 2 +x-2 = 212
e) 1 + 3 + 32 + .... + 3x = 314
g) 2x - 2y = 7
2. a) So sánh 2150 và 3100
b) Tìm chữ số tận cùng của A = 22023 + 32024
a)19 - (x + 23)=24- 6
19 - (x + 23) = 16 - 6
19 - (x + 23) = 10
(x + 23) = 19 - 10
x + 23= 9
x + 23 = 33
x + 2 = 3
x= 3-2
x= 1
Đúng 0
Bình luận (7)
sửa lại :
a)19 - (x + 23)=24- 6
19 - (x + 23) = 16 - 6
19 - (x + 23) = 10
(x + 23) = 19 - 10
x + 23= 9
=> x + 8= 9 x= 1
=> x + 8 =-9 x= -17
Đúng 0
Bình luận (1)
Bài 1: Tìm số hữu tỉ x biết:a, ( 2x - 1 )4 81 b, ( x - 1 )5 -32 c, ( 2x - 1 )6 ( 2x - 1 )8Bài 2: Tìm các số tự nhiên x, y biết rằng: a, 2x + 1 . 3y 12x. b, 10x : 5y 20y c, 2x 4y - 1 và 27y 3x + 8
Đọc tiếp
Bài 1: Tìm số hữu tỉ x biết:
a, ( 2x - 1 )4 = 81 b, ( x - 1 )5 = -32
c, ( 2x - 1 )6 = ( 2x - 1 )8
Bài 2: Tìm các số tự nhiên x, y biết rằng:
a, 2x + 1 . 3y = 12x. b, 10x : 5y = 20y
c, 2x = 4y - 1 và 27y = 3x + 8
Bài 2:
a: Ta có: \(2^{x+1}\cdot3^y=12^x\)
\(\Leftrightarrow2^{x+1}\cdot3^y=2^{2x}\cdot3^x\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+1=2x\\x=y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=1\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Tìm x biết
2x/15+2x/35+2x/63+....+2x/195=4/5
Chứng minh rằng 1/2-1/4+1/8-1/16+1/32-1/1/64<1/3
\(\dfrac{2x}{15}+\dfrac{2x}{35}+\dfrac{2x}{63}+...+\dfrac{2x}{195}=\dfrac{4}{5}\\ x\cdot\left(\dfrac{2}{15}+\dfrac{2}{35}+\dfrac{2}{63}+...+\dfrac{2}{195}\right)=\dfrac{4}{5}\\ x\cdot\left(\dfrac{2}{3\cdot5}+\dfrac{2}{5\cdot7}+\dfrac{2}{7\cdot9}+...+\dfrac{2}{13\cdot15}\right)=\dfrac{4}{5}\\ x\cdot\left(\dfrac{1}{3}-\dfrac{1}{5}+\dfrac{1}{5}-\dfrac{1}{7}+\dfrac{1}{7}-\dfrac{1}{9}+...+\dfrac{1}{13}-\dfrac{1}{15}\right)=\dfrac{4}{5}\\ x\cdot\left(\dfrac{1}{3}-\dfrac{1}{15}\right)=\dfrac{4}{5}\\ x\cdot\dfrac{4}{15}=\dfrac{4}{5}\\ x=\dfrac{4}{5}:\dfrac{4}{15}\\ x=3\)
Gọi \(D=\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{8}-\dfrac{1}{16}+\dfrac{1}{32}-\dfrac{1}{64}\)
\(2D=1-\dfrac{1}{2}+\dfrac{1}{4}-\dfrac{1}{8}+\dfrac{1}{16}-\dfrac{1}{32}\\ 2D+D=\left(1-\dfrac{1}{2}+\dfrac{1}{4}-\dfrac{1}{8}+\dfrac{1}{16}-\dfrac{1}{32}\right)+\left(\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{8}-\dfrac{1}{16}+\dfrac{1}{32}-\dfrac{1}{64}\right)\\ 3D=1-\dfrac{1}{2}+\dfrac{1}{4}-\dfrac{1}{8}+\dfrac{1}{16}-\dfrac{1}{32}+\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{8}-\dfrac{1}{16}+\dfrac{1}{32}-\dfrac{1}{64}\\ 3D=1-\dfrac{1}{64}< 1\\ \Rightarrow D=\dfrac{1-\dfrac{1}{64}}{3}< \dfrac{1}{3}\)
Vậy \(\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{8}-\dfrac{1}{16}+\dfrac{1}{32}-\dfrac{1}{64}< \dfrac{1}{3}\)
Đúng 0
Bình luận (0)
(2x+1)^3=125
(2x-1)^4=16
6.3^x-2.3^x=36
2^x+1-2^x=32
\(\left(2x+1\right)^3=125\\ \Rightarrow\left(2x+1\right)^3=5^3\\ \Rightarrow2x+1=5\\ \Rightarrow2x=4\\ \Rightarrow x=2.\\ b,\left(2x-1\right)^4=16\\ \Rightarrow\left(2x-1\right)^4=2^4\\ \Rightarrow2x-1=2\\ \Rightarrow2x=3\\ \Rightarrow x=\dfrac{3}{2}.\\ c,6.3^x-2.3^x=36\\ \Rightarrow3^x.\left(6-2\right)=36\\ \Rightarrow3^x.4=36\\ \Rightarrow3^x=9\\ \Rightarrow3^x=3^2\\ \Rightarrow x=2.\\ d,2^{x+1}-2^x=32\\ \Rightarrow2^x.\left(2-1\right)=32\\ \Rightarrow2^x=2^5\\ \Rightarrow x=5.\)
Đúng 0
Bình luận (0)
tìm x thuộc Q , biết :
a, ( 2x - 1)^4 = 81
b, ( x - 1) ^ 5 = - 32
c, ( 2x - 1)^6 = ( 2x - 1)^8
a. (2x-1)4=81
=>(2x-1)4=34
=>2x-1=3
=>2x=3+1
=>2x=4
=>x=4:2
=>x=2
b.(x-1)5=-32
=>(x-1)5=(-2)5
=>x-1=-2
=>x=-2+1
=>x=-1
c.(2x-1)6=(2x-1)8
mà chỉ có: (-1)6=(-1)8; 06=08; 16=18
=> để (2x-1) \(\in\){-1;0;1} thì x \(\in\){0; 1/2; 1}
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Rút gọn :
1. (2x-5)(3x+1)-(x-3)^2+(2x+5)^2-(3x+1)^3
2. (2x-1)(2x+1)-3x-2)(2x+3)-(x-1)^3+(2x+3)^3
3. (x-2)(x^2+2x+4)-(3x-2)^3+(3x-4)^2
4. (7x-1)(8x+2)-(2x-7)^2-(x-4)^3-(3x+1)^3
5. (5x-1)(5x+1)-(x+3)(x^2-3x+9)-(2x+4)^2-(3x-4)^2+(2x-5)^3
6. (4x-1)(x+2)-(2x+5)^2-(3x-7)^2+(2x+3)^3=(3x-1)^3
1: \(=6x^2+2x-15x-5-x^2+6x-9+4x^2+20x+25-27x^3-27x^2-9x-1\)
=-27x^3-18x^2+4x+10
2: =4x^2-1-6x^2-9x+4x+6-x^3+3x^2-3x+1+8x^3+36x^2+54x+27
=7x^3+37x^2+46x+33
5:
\(=25x^2-1-x^3-27-4x^2-16x-16-9x^2+24x-16+\left(2x-5\right)^3\)
\(=8x^3-60x^2+150-125+12x^2-x^3+8x-60\)
=7x^3-48x^2+8x-35
Đúng 0
Bình luận (0)