Cho các số thực a,b,c thuộc đoạn [0;1]. Tìm Max
\(P=\dfrac{a}{b+c+1}+\dfrac{b}{a+c+1}+\dfrac{c}{a+b+1}+\left(1-a\right)\left(1-b\right)\left(1-c\right)\)
Cho các số thực a, b, c (với a ≠ 0 sao cho: phương trình a x 2 + b x + c = 0 có hai nghiệm thuộc đoạn [0;1]. Tìm giá trị lớn nhất của biểu thức:
A. 1
B. 3
C. 4
D. 5
Cho a,b,c là các số thực thuộc đoạn [0,1 ] .Chứng minh:
\(a\left(b-1\right)+b\left(1-c\right)+c\left(1-a\right)\le1\)
\(a\left(b-1\right)+b\left(1-c\right)+c\left(1-a\right)\le1\\ \Leftrightarrow-abc+ab+bc+ca-a-b-c+1\le2-abc\\ \Leftrightarrow\left(1-a\right)\left(1-b\right)\left(1-c\right)\le2-abc\)
lại có \(abc\le1\) nên \(2-abc\ge1\)
ta chứng minh \(\left(1-a\right)\left(1-b\right)\left(1-c\right)\le1\)
luôn đúng do \(0\le a;b;c\le1\)
vậy bđt dc cm
tick mik nhaaaaa.mik ms l9 thui
Cho a,b,c là các số thực thuộc đoạn [1,2 ].Chứng minh rằng:
\(\left(a+b+c\right)\left(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\right)\le10\)
Không mất tính tổng quát, giả sử \(a\ge b\ge c\).
Khi đó: \(\left(a-b\right)\left(b-c\right)\ge0\)
\(\Leftrightarrow ab+bc\ge ac+b^2\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{a}{c}+1\ge\dfrac{a}{b}+\dfrac{b}{c}\\\dfrac{c}{a}+1\ge\dfrac{c}{b}+\dfrac{b}{a}\end{matrix}\right.\)
\(\Rightarrow\dfrac{a}{b}+\dfrac{b}{c}+\dfrac{c}{a}+\dfrac{b}{a}+\dfrac{c}{b}+\dfrac{a}{c}\le2+2\left(\dfrac{a}{c}+\dfrac{c}{a}\right)\)
Vì \(1\le c\le a\le2\Rightarrow\left(\dfrac{a}{c}-2\right)\left(\dfrac{2a}{c}-1\right)\le0\)
\(\Leftrightarrow\dfrac{a}{c}+\dfrac{c}{a}\le\dfrac{5}{2}\)
\(\Rightarrow\dfrac{a}{b}+\dfrac{b}{c}+\dfrac{c}{a}+\dfrac{b}{a}+\dfrac{c}{b}+\dfrac{a}{c}\le7\)
\(\Leftrightarrow\left(a+b+c\right)\left(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\right)\le10\)
Đẳng thức xảy ra khi \(a=b=2;c=1\) và các hoán vị.
giúp mình với: cho a,b,c là các số thực thuộc đoạn [0;1]. tìm GTLN của biểu thức P=a(1-b)+b(1-c)+c(1-a)
Cho a, b, c là các số thực thuộc đoạn [1;2] thỏa mãn 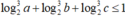 . Khi biểu thức
. Khi biểu thức ![]()
đạt giá trị lớn nhất thì giá trị của tổng a+b+c là
A. 3
B. 3 . 2 1 3 3
C. 4
D. 6
Cho a,b,c là các số thực thuộc khoảng (0:1) thỏa mãn abc=(1-a)(1-b)(1-c)
Tìm GTNN của P=a+b+c\(+\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\)
Cho x,y,z là ba số thực dương thuộc đoạn [1;2] thỏa mãn điều kiện \(a^2+b^2+c^2=6\). CMR \(a+b+c\text{ ≥}0\)
\(1\le x\le2\Rightarrow x-1\ge0\) và \(x-2\le0\Rightarrow\left(x-1\right)\left(x-2\right)\le0\)
\(\Rightarrow x^2\le3x-2\)
Tương tự \(y^2\le3y-2\) và \(z^2\le3z-2\)
\(\Rightarrow x^2+y^2+z^2\le3\left(x+y+z\right)-6\le3.5-6=9\)
Cho hai điểm A,B thuộc đồ thị hàm số y=sinx trên đoạn 0 ; π , các điểm C,D thuộc trục Ox sao cho tứ giác ABCD là hình chữ nhật và C D = 2 π 3 .
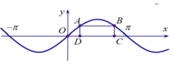
Độ dài đoạn thẳng BC bằng
A. 2 2 .
B. 1 2 .
C.1
D. 3 2 .
Cho hai điểm A, B thuộc đồ thị hàm số y=sinx trên đoạn 0 ; π các điểm C, D thuộc trục Ox sao cho tứ giác ABCD là hình chữ nhật và CD = 2 π 3
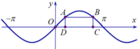
Độ dài đoạn thẳng BC bằng
A. 2 2
B. 1 2
C. 1
D. - 2 2