giúp e dc k ạ 😞
NH
Những câu hỏi liên quan
Giúp e bài 3 vs ạ 😞😞😞
\(a,\\ \left\{{}\begin{matrix}x< 1.hoặc.x>2\\\dfrac{2x^2-3x-2}{3x+1}\le0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< 1.hoặc.x>2\\x\le\dfrac{-1}{2}.hoặc.\dfrac{-1}{3}< x< 1\end{matrix}\right.\\ \Rightarrow x\le-\dfrac{1}{2}.hoặc.-\dfrac{1}{3}< x< 1\)
\(b,\\ \left\{{}\begin{matrix}x< -4.hoặc.x>1\\\dfrac{2x+1}{2x^2-5x+2}\le0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x< -4.hoặc.x>1\\x\le-\dfrac{1}{2}hoặc.\dfrac{1}{2}< x< 2\end{matrix}\right.\\ \Rightarrow x< -4.hoặc.1< x< 2\)
Đúng 1
Bình luận (0)
Giúp em bài 7 với ạ😞😞😞. E cảm ơn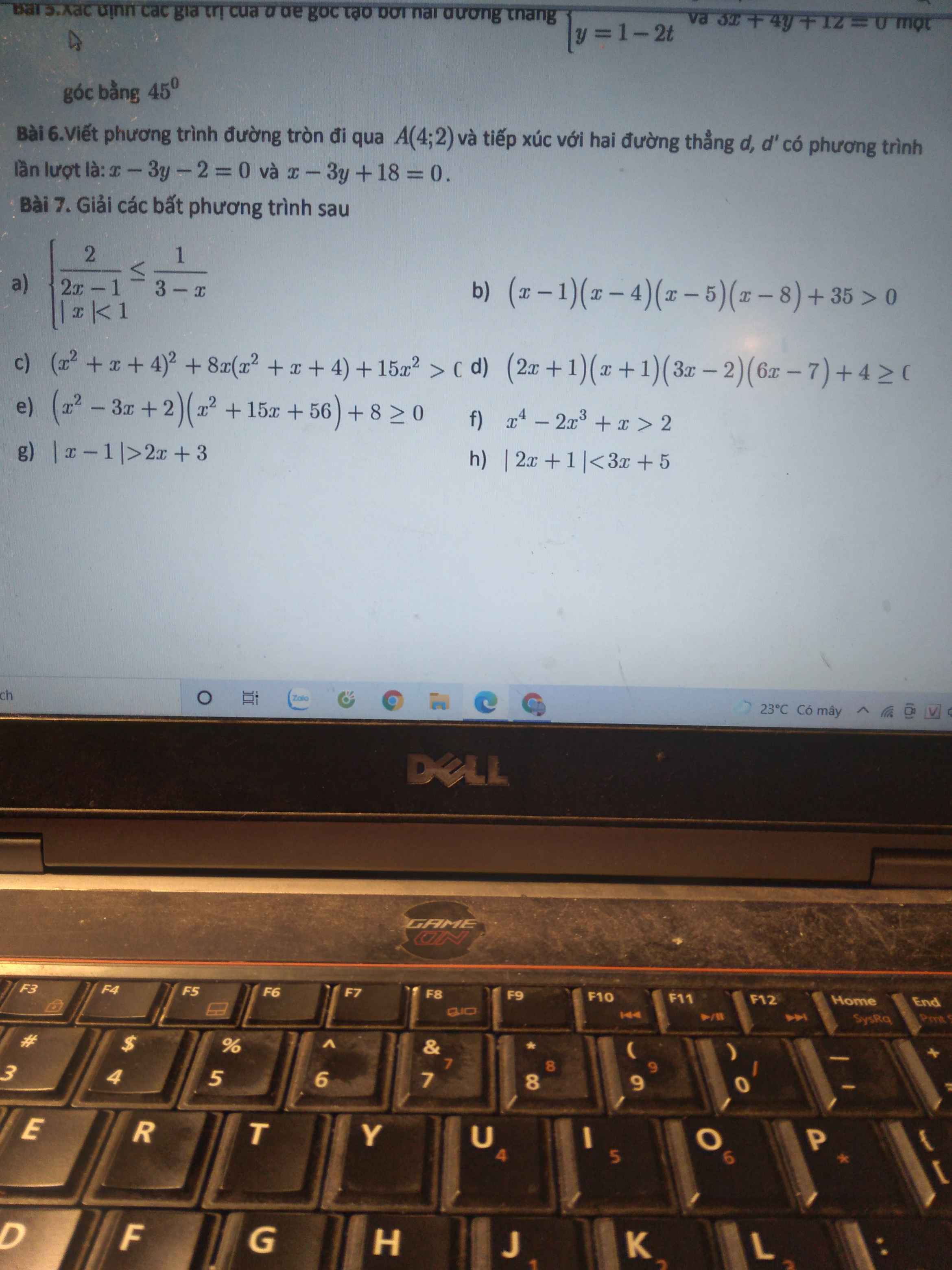
\(\left(x\ne3;x\ne\dfrac{1}{2}\right)\)\(\left\{{}\begin{matrix}\dfrac{2}{2x-1}\le\dfrac{1}{3-x}\\\left|x\right|< 1\Leftrightarrow-1< x< 1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2\left(3-x\right)-2x+1}{\left(2x-1\right)\left(3-x\right)}\le0\left(1\right)\\-1< x< 1\end{matrix}\right.\)\(\)
\(\left(1\right)\Leftrightarrow\dfrac{-4x+7}{\left(2x-1\right)\left(3-x\right)}\le0\)\(\Leftrightarrow\dfrac{-4x+7}{-2x^2+7x-3}\le0\Leftrightarrow x\in\left(-\infty;\dfrac{1}{2}\right)\cup[\dfrac{7}{4};3)\)
\(kết\) \(hợp:-1< x< 1\)\(\Rightarrow x\in\left(-1;\dfrac{1}{2}\right)\cup[\dfrac{7}{4};3)\)
\(b,\)\(\left(x-1\right)\left(x-4\right)\left(x-5\right)\left(x-8\right)+35>0\)
\(\Leftrightarrow\left(x^2-9x+8\right)\left(x^2-9x+20\right)+35>0\)
\(đặt:x^2-9x+8=t\ge-\dfrac{49}{4}\)
\(bpt\Leftrightarrow t\left(t+12\right)+35>0\Leftrightarrow t^2+12t+35>0\Leftrightarrow\left[{}\begin{matrix}t< -7\\t>-5\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x^2-9x+8< -7\\x^2-9x+8>-5\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{9-\sqrt{21}}{2}< x< \dfrac{9+\sqrt{21}}{2}\\x\in\left(-\infty;\dfrac{9-\sqrt{29}}{2}\right)\cup\left(\dfrac{9+\sqrt{29}}{2};+\infty\right)\end{matrix}\right.\)
\(c;\)\(\left(x^2+x+4\right)^2+2.4x\left(x^2+x+4\right)+16x^2-x^2>0\)
\(\Leftrightarrow\left(x^2+x+4+4x\right)^2-x^2>0\)
\(\Leftrightarrow\left(x+2\right)^2\left(x^2+6x+4\right)>0\)
\(\Leftrightarrow x^2+6x+4>0\Leftrightarrow....\)
ý d; giống ý b
\(e;bpt\Leftrightarrow\left(x-2\right)\left(x-1\right)\left(x+7\right)\left(x+8\right)+8>0\)
\(\Leftrightarrow\left(x^2+6x-16\right)\left(x^2+6x-7\right)+8>0\)
\(đặt:x^2+6x-7=t\ge-16\Rightarrow t\left(t-9\right)+8>0\)
(làm giống ý b)
\(f;x^4-2x^3+x-2>0\Leftrightarrow\left(x+1\right)\left(x-2\right)\left(x^2-x+1\right)>0\left(do:x^2-x+1=\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0\right)\)
\(\Rightarrow bpt\Leftrightarrow\left(x+1\right)\left(x-2\right)>0\Leftrightarrow\left[{}\begin{matrix}x< -1\\x>2\end{matrix}\right.\)
\(g;h\) dùng bảng phá giá trị tuyệt đối để làm
Đúng 0
Bình luận (0)
Giúp mình với ạ😞😞😞😞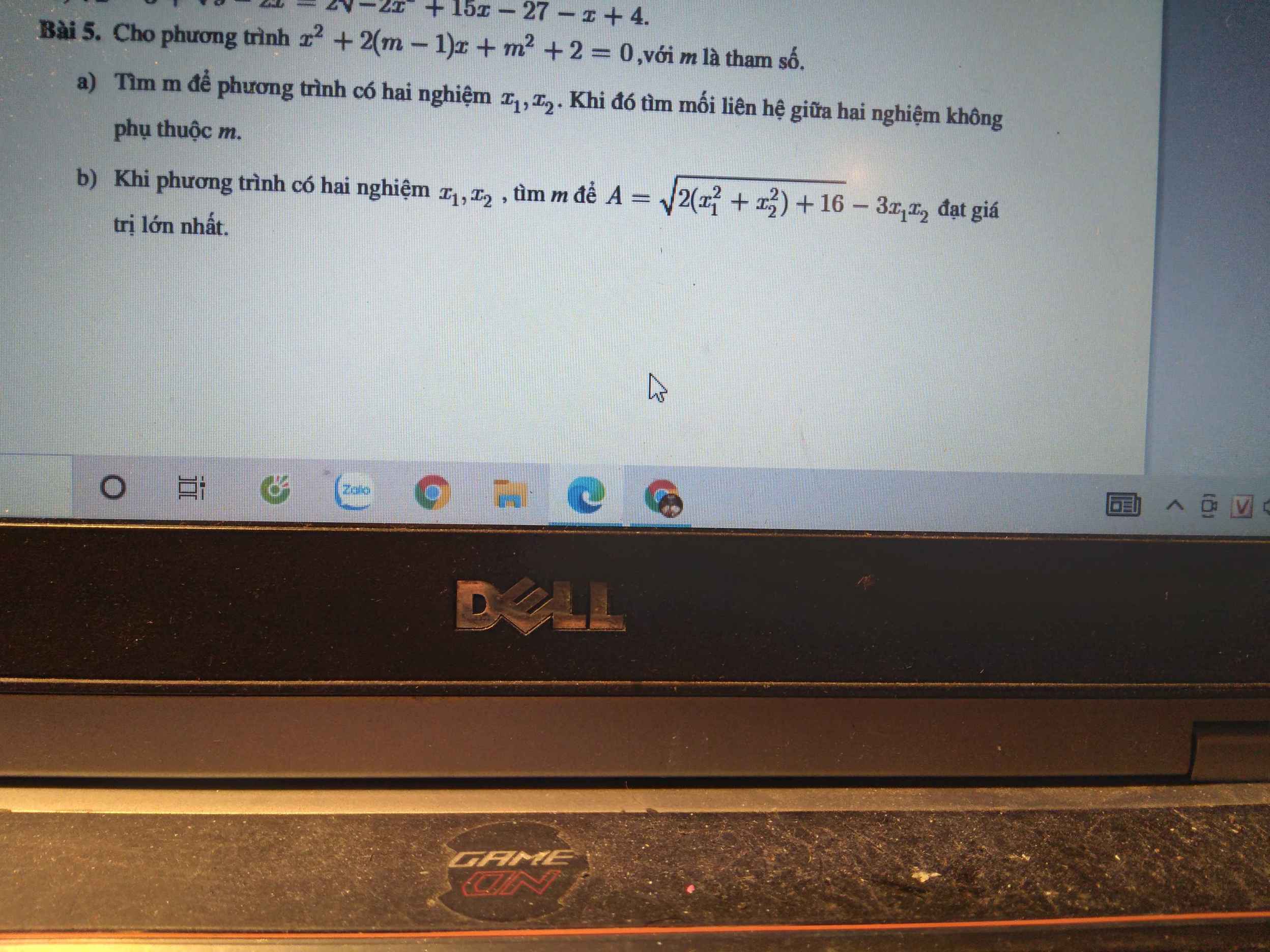
\(\Delta'=\left(m-1\right)^2-\left(m^2+2\right)=-2m-1\ge0\Rightarrow m\le-\dfrac{1}{2}\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=m^2+2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{x_1+x_2+2}{2}=m\\x_1x_2-2=m^2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\left(\dfrac{x_1+x_2+2}{x}\right)^2=m^2\\x_1x_2-2=m^2\end{matrix}\right.\)
\(\Rightarrow\left(\dfrac{x_1+x_2+2}{2}\right)^2=x_1x_2-2\)
Đây là hệ thức liên hệ 2 nghiệm ko phụ thuộc m
b.
\(A=\sqrt{2\left(x_1+x_2\right)^2-4x_1x_2+16}-3x_1x_2\)
\(=\sqrt{8\left(m-1\right)^2-4\left(m^2+2\right)+16}-3\left(m^2+2\right)\)
\(=\sqrt{4m^2-16m+16}-3\left(m^2+2\right)\)
\(=\sqrt{\left(4-2m\right)^2}-3m^2-6\)
\(=\left|4-2m\right|-3m^2-6\)
\(=4-2m-3m^2-6\) (do \(m\le-\dfrac{1}{2}\Rightarrow4-2m>0\))
\(=-3m^2-2m-2\)
\(=-\dfrac{1}{4}\left(12m^2+8m+1\right)-\dfrac{7}{4}\)
\(=-\dfrac{1}{4}\left(6m+1\right)\left(2m+1\right)-\dfrac{7}{4}\le-\dfrac{7}{4}\)
\(A_{max}=-\dfrac{7}{4}\) khi \(m=-\dfrac{1}{2}\)
Đúng 2
Bình luận (0)
Giúp em bài này với ạ. Cần gấp😞😞😞😞😔
Giúp em bài 2 và 3 với ạ 😞😞😞
Giúp em bài 1 vs ạ. Cần gấp😞😞😞
giải dùm mình câu nào cũng dc ạ 😞 3 câu luôn càng tốt ạ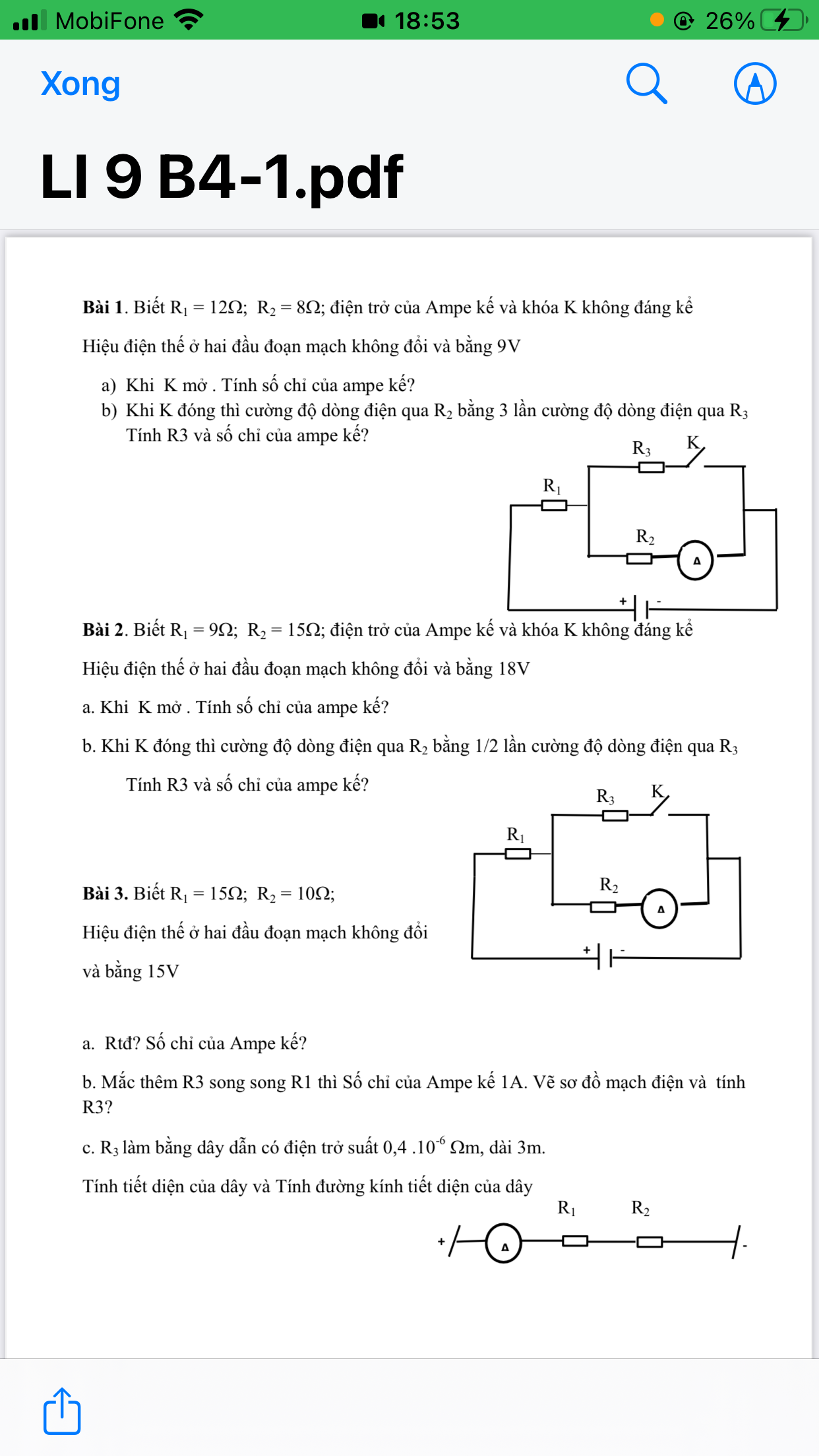
Giúp mk bài 2 ạ 😞😞
Bài 6:
b: x+3y-6=0
=>x+3y=6
=>3y=6-x
=>y=-1/3x+2
Vì (d) vuông góc với y=-1/3x+2 nên -1/3a=-1
=>a=3
Vậy: (d): y=3x+b
Thay x=2 và y=5 vào (d), ta được:
b+6=5
hay b=-1
Đúng 0
Bình luận (0)
các ac giúp e dc k ạ, e cảm ơn rất nhiều
a.Áp dụng định lý pitago vào tam giác vuông PKQ, ta có:
\(QK^2=PQ^2+PK^2\)
\(\Rightarrow QK=\sqrt{6^2+8^2}=\sqrt{100}=10cm\)
Áp dụng t/c đường phân giác góc P, ta có:
\(\dfrac{PQ}{PK}=\dfrac{AP}{AK}\)
\(\Leftrightarrow\dfrac{6}{8}=\dfrac{AP}{AK}\) \(\Leftrightarrow\dfrac{3}{4}=\dfrac{AP}{AK}\) \(\Leftrightarrow\dfrac{AK}{4}=\dfrac{AP}{3}\)
Áp dụng t/c dãy tỉ số bằng nhau, ta có:
\(\dfrac{AK}{4}=\dfrac{AP}{3}=\dfrac{AK+AP}{4+3}=\dfrac{QK}{7}=\dfrac{10}{7}\)
\(\Rightarrow AK=\dfrac{10}{7}.4=\dfrac{40}{7}cm\)
\(\Rightarrow AP=\dfrac{10}{7}.3=\dfrac{30}{7}cm\)
b. Xét tam giác PBQ và tam giác PQK, có:
\(\widehat{PBQ}=\widehat{QPK}=90^0\)
\(\widehat{Q}:chung\)
Vậy tam giác PBQ đồng dạng tam giác PQK ( g.g )
\(\Rightarrow\dfrac{PB}{PK}=\dfrac{PQ}{QK}\)
\(\Leftrightarrow\dfrac{PB}{8}=\dfrac{6}{10}\) \(\Leftrightarrow\dfrac{PB}{8}=\dfrac{3}{5}\)
\(\Leftrightarrow5PB=24\) \(\Leftrightarrow PB=\dfrac{24}{5}cm\)
c. Xét tam giác PBQ và tam giác PBK, có:
\(\widehat{PBQ}=\widehat{PBK}=90^0\)
\(\widehat{PQB}=\widehat{BPK}\) ( cùng phụ với \(\widehat{A}\) )
Vậy tam giác PBQ đồng dạng tam giác PBK ( g.g )
\(\Rightarrow\dfrac{PB}{BK}=\dfrac{QB}{PB}\)
\(\Leftrightarrow PB^2=BK.QB\)
Đúng 2
Bình luận (0)



